U.S. Department of Transportation
Federal Highway Administration
1200 New Jersey Avenue, SE
Washington, DC 20590
202-366-4000
Federal Highway Administration Research and Technology
Coordinating, Developing, and Delivering Highway Transportation Innovations
 |
| This report is an archived publication and may contain dated technical, contact, and link information |
|
Publication Number: FHWA-RD-03-083 Date: June 2003 |
Determination of local scour at bridge piers is one of the critical problems in the design of bridge foundations to resist the erosive action of oncoming flows. Even though local pier scour has been the topic of large numbers of research studies in noncohesive alluvial materials, very little effort has been devoted to the study of pier scour in cohesive materials. The focus of this experimental study is to identify the parameters affecting the scour mechanism at bridge piers located in unsaturated compacted cohesive soils and in saturated cohesive soils and to develop prediction equations to quantify the local scour depths. Based on the analysis of the experimental data, scour depth predictors are developed in terms of the approach flow conditions, initial water content, compaction, and soil shear strength using Montmorillonite clay soils.
In the past, numerous experimental and analytical investigations of local pier scour were conducted in alluvial channels, and series of prediction equations were developed by researchers to estimate the maximum scour depth at bridge piers under different approach flow conditions, for different sediment size and gradations, and for different pier type and sizes. Unfortunately, these studies have been all confined to noncohesive soils. This is undoubtedly due to not only the abundance of streams with these types of beds but also because sand and gravel are easier to both characterize and model physically.
The scour of cohesive materials is fundamentally different from that of noncohesive materials. It involves not only complex mechanical phenomena, including shear stress and shear strength of soils, but also the chemical and physical bonding of individual particles and properties of the eroding fluid. Cohesive materials, once eroded, remain in suspension such that clear-water scour conditions always prevail. Along with the eroding fluid properties, the scour process in this environment is strongly affected by the amount of cohesive material present in the soil mixture as well as the type of mineral clay, initial water content, soil shear strength, and compaction of the clay. The objectives of this paper are to apply the knowledge gained in the past in cohesive material scour to local pier scour, and specifically to: 1) study the effect of compaction, soil shear strength, and the approach flow conditions on pier scour in unsaturated cohesive soils; 2) specify the influence of initial water content of saturated clay on pier scour; and 3) develop scour prediction equations in unsaturated and saturated cohesive soils to quantify the scour that may occur around circular piers.
Pier scour experiments presented in this paper are broadly classified as unsaturated and saturated cohesive soil experiments. In each category of experiments, series of runs utilizing different flumes were conducted by varying the clay properties under different flow conditions. Details and scope of experiments are presented by Hosny(21) and by Molinas, Hosny, and Jones(24, 25).
Flumes
Experiments were conducted in three different test flumes housed at the Engineering Research Center at CSU. These flumes were identified as the river mechanics flume, sediment transport flume, and the steep flume. The river mechanics flume is 5 m wide by 30 m long, the sediment transport flume is 2.4 m wide by 60 m long, and the steep flume is 1.2 m wide by 12 m long. The flow depths in the experiments varied between 0.12 m and 0.3 m, and the corresponding approach Froude numbers ranged from 0.1 to 1.4.
Measurements
In pier scour experiments the measured flow and sediment parameters were velocity, depth of flow, depth of scour, initial water content of soil, Torvane shear strength, and degree of compaction. For velocity measurements, a one-dimensional magnetic flow-meter was utilized. In each experiment, approach velocity to each pier was determined by depth- and width-integrated average of vertical velocity profiles. Similarly, the approach depth was determined from a width- and length-averaged value of water surface elevation measurements. The bed elevations were measured along the test flumes before and after each experiment. The depth of scour was measured during and at the end of each experiment; it was determined by the difference between the measured minimum bottom elevation at the nose of a pier and the maximum elevation away from the structure.
Cohesive Soils
To achieve the objectives of this study, a homogeneous soil containing clay, silt, and fine sand particles, in which cohesion plays a predominant role, was used. Utilizing the X-Ray Diffraction Test, the dominant clay mineral was found to be Montmorillonite. According to the unified soil classification system, the cohesive soil was also classified as medium plasticity clay and the texture as clay loam.
In this study, three series of experiments were performed. In set 1, the effects of clay content were examined as the clay content was varied from 0 to 12 percent. In set 2 experiments, the effects of compaction on unsaturated cohesive soils were studied. The cohesive material placed around the piers was compacted at 58, 65, 73, 80, 87, and 93 percent degree of compaction. In set 3 experiments, the effects of initial water content on saturated cohesive soil erosion were examined by saturating the soils at initial water contents of 32, 35, 40, and 45 percent.
Piers
In the experiments, 1-m long cylindrical piers made of clear Plexiglas with 0.152-m and 0.102-m diameters were used. The scour depth development was measured against time utilizing three measuring tapes attached to the interior wall of each pier and a periscope manufactured by the use of a small inclined mirror.
Tables 25 through 28 present the experimental conditions as well as the resulting scour depths for pier experiments in Montmorillonite clay mixtures. Pier scour experiments, identified as runs MH 13-1 through MH 22-3 in table 25, were conducted in the 5-m wide flume, and runs MH30-1 through MH 32-3 were conducted in the 2.4-m wide flume. In these experiments sand-clay mixtures were prepared utilizing Montmorillonite clay soil. In addition to the measured depth of scour values, the summary tables also present scour hole volumes for each experiment. The pier diameter used in these experiments was 0.15 m.
Table 25. Results of set 1 experiments to study effects of clay content.
| Run ID |
Flow Discharge Q (l/s) |
Clay Content CC (% ) |
Approach Depth Y (m) |
Approach Velocity V (m/s) |
Froude No. Fr |
Scour Depth Ds (m) |
Dimension- less Scour Ds / b |
Volume of Scour Vs (l) |
Dimension- less Volume Vs / b3 |
|---|---|---|---|---|---|---|---|---|---|
| MH 13-1 | 311.49 | 0.0 | 0.237 | 0.280 | 0.184 | 0.088 | 0.58 | 8.100 | 02.288 |
| MH 13-B | 311.49 | 1.5 | 0.237 | 0.301 | 0.198 | 0.076 | 0.50 | 6.701 | 01.893 |
| MH 13-3 | 311.49 | 3.0 | 0.237 | 0.311 | 0.204 | 0.092 | 0.61 | 10.001 | 02.825 |
| MH 14-1 | 283.17 | 0.0 | 0.210 | 0.253 | 0.176 | 0.069 | 0.46 | 2.701 | 00.763 |
| MH 14-2 | 283.17 | 1.5 | 0.210 | 0.282 | 0.196 | 0.077 | 0.50 | 6.555 | 01.852 |
| MH 14-3 | 283.17 | 3.0 | 0.210 | 0.271 | 0.189 | 0.066 | 0.43 | 3.449 | 00.975 |
| MH 15-1 | 339.80 | 0.0 | 0.224 | 0.274 | 0.185 | 0.089 | 0.58 | 8.100 | 02.288 |
| MH 15-2 | 339.80 | 1.5 | 0.224 | 0.307 | 0.207 | 0.105 | 0.69 | 13.999 | 03.955 |
| MH 15-3 | 339.80 | 3.0 | 0.224 | 0.313 | 0.211 | 0.091 | 0.60 | 9.000 | 02.543 |
| MH 16-1 | 413.43 | 0.0 | 0.244 | 0.316 | 0.205 | 0.116 | 0.76 | 16.600 | 04.690 |
| MH 16-2 | 413.43 | 1.5 | 0.244 | 0.356 | 0.230 | 0.127 | 0.83 | 19.601 | 05.537 |
| MH 16-3 | 413.43 | 3.0 | 0.244 | 0.377 | 0.244 | 0.144 | 0.94 | 19.992 | 05.648 |
| MH 17-1 | 283.17 | 6.0 | 0.210 | 0.253 | 0.176 | 0.045 | 0.30 | 1.450 | 00.410 |
| MH 17-2 | 283.17 | 9.0 | 0.210 | 0.282 | 0.196 | 0.052 | 0.34 | 2.730 | 00.771 |
| MH 17-3 | 283.17 | 12.0 | 0.210 | 0.271 | 0.189 | 0.051 | 0.34 | 2.466 | 00.697 |
| MH 18-1 | 339.80 | 6.0 | 0.224 | 0.274 | 0.185 | 0.058 | 0.38 | 3.089 | 00.873 |
| MH 18-2 | 339.80 | 9.0 | 0.224 | 0.307 | 0.207 | 0.057 | 0.37 | 5.073 | 01.434 |
| MH 18-3 | 339.80 | 12.0 | 0.224 | 0.313 | 0.211 | 0.060 | 0.39 | 4.105 | 01.160 |
| MH 19-1 | 413.43 | 6.0 | 0.243 | 0.327 | 0.212 | 0.066 | 0.44 | 6.560 | 01.853 |
| MH 19-2 | 413.43 | 9.0 | 0.243 | 0.358 | 0.232 | 0.096 | 0.63 | 14.584 | 04.120 |
| MH 19-3 | 413.43 | 12.0 | 0.243 | 0.362 | 0.235 | 0.084 | 0.55 | 9.100 | 02.571 |
| MH 20-1 | 382.28 | 6.0 | 0.230 | 0.319 | 0.212 | 0.079 | 0.52 | 6.350 | 01.794 |
| MH 20-2 | 382.28 | 9.0 | 0.230 | 0.347 | 0.231 | 0.105 | 0.69 | 15.576 | 04.400 |
| MH 20-3 | 382.28 | 12.0 | 0.230 | 0.349 | 0.232 | 0.096 | 0.63 | 11.317 | 03.197 |
| MH 21-1 | 339.80 | 6.0 | 0.224 | 0.274 | 0.185 | 0.058 | 0.38 | 3.366 | 00.951 |
| MH 21-2 | 339.80 | 1.5 | 0.224 | 0.307 | 0.207 | 0.105 | 0.69 | 13.999 | 03.955 |
| MH 21-3 | 339.80 | 3.0 | 0.224 | 0.313 | 0.211 | 0.092 | 0.60 | 9.000 | 02.543 |
| MH 22-1 | 283.17 | 6.0 | 0.214 | 0.261 | 0.180 | 0.054 | 0.35 | 1.450 | 00.410 |
| MH 22-2 | 283.17 | 1.5 | 0.214 | 0.282 | 0.195 | 0.077 | 0.50 | 6.701 | 01.893 |
| MH 22-3 | 283.17 | 3.0 | 0.214 | 0.272 | 0.188 | 0.068 | 0.44 | 5.088 | 01.438 |
| MH 30-1 | 368.12 | 0.0 | 0.290 | 0.517 | 0.307 | 0.248 | 1.63 | 47.999 | 13.561 |
| MH 30-2 | 368.12 | 6.0 | 0.290 | 0.534 | 0.317 | 0.158 | 1.04 | 38.000 | 10.736 |
| MH 30-3 | 368.12 | 12.0 | 0.290 | 0.555 | 0.329 | 0.094 | 0.62 | 28.001 | 07.910 |
| MH 31-1 | 368.12 | 3.0 | 0.290 | 0.517 | 0.307 | 0.210 | 1.38 | 46.400 | 13.109 |
| MH 31-2 | 368.12 | 9.0 | 0.290 | 0.522 | 0.310 | 0.166 | 1.09 | 39.336 | 11.113 |
| MH 31-3 | 368.12 | 12.0 | 0.290 | 0.555 | 0.329 | 0.135 | 0.88 | 31.140 | 08.798 |
| MH 32-1 | 254.85 | 0.0 | 0.247 | 0.361 | 0.232 | 0.191 | 1.25 | 32.817 | 09.271 |
| MH 32-2 | 254.85 | 6.0 | 0.247 | 0.353 | 0.227 | 0.122 | 0.80 | 16.387 | 04.630 |
| MH 32-3 | 254.85 | 12.0 | 0.259 | 0.364 | 0.228 | 0.089 | 0.58 | 16.059 | 04.537 |
Notes:
Table 26. Summary of experimental conditions and results for set 2 (effect of compaction on pier scour in cohesive soils).
| RUN ID | Flow Discharge Q (l/s) |
Shear Strength S (kPa) |
Wet Density (w (t/m3) |
Dry Density (dry (t/m3) |
Compaction C (%) |
Approach Depth Y (m) |
Approach Velocity V (m/s) |
Froude No. Fr |
Scour Depth Dsc (m) |
Dimension- less Scour Dsc / b |
Scour Volume Vs (l) |
|---|---|---|---|---|---|---|---|---|---|---|---|
| MH 13-5 | 311.485 | 44.132 | 1.968 | 1.640 | 93 | 0.245 | 0.255 | 0.165 | 0.000 | 0.00 | 0.000 |
| MH 13-6 | 311.485 | 37.267 | 1.841 | 1.534 | 87 | 0.245 | 0.260 | 0.168 | 0.000 | 0.00 | 0.000 |
| MH 14-5 | 283.168 | 31.382 | 1.693 | 1.411 | 80 | 0.216 | 0.266 | 0.182 | 0.046 | 0.30 | 0.900 |
| MH 14-6 | 283.168 | 25.498 | 1.545 | 1.287 | 73 | 0.216 | 0.264 | 0.181 | 0.057 | 0.37 | 1.050 |
| MH 15-5 | 339.802 | 44.132 | 1.968 | 1.640 | 93 | 0.228 | 0.291 | 0.195 | 0.000 | 0.00 | 0.000 |
| MH 15-6 | 339.802 | 37.267 | 1.841 | 1.534 | 87 | 0.228 | 0.302 | 0.202 | 0.000 | 0.00 | 0.000 |
| MH 16-5 | 413.426 | 29.421 | 1.693 | 1.411 | 80 | 0.250 | 0.312 | 0.199 | 0.060 | 0.40 | 1.070 |
| MH 16-6 | 413.426 | 24.518 | 1.545 | 1.287 | 73 | 0.250 | 0.344 | 0.220 | 0.073 | 0.48 | 2.794 |
| MH 17-5 | 283.168 | 31.382 | 1.693 | 1.411 | 80 | 0.216 | 0.266 | 0.182 | 0.047 | 0.31 | 0.811 |
| MH 17-6 | 283.168 | 25.498 | 1.545 | 1.287 | 73 | 0.216 | 0.264 | 0.181 | 0.053 | 0.35 | 1.231 |
| MH 18-5 | 339.802 | 31.382 | 1.693 | 1.411 | 80 | 0.228 | 0.291 | 0.195 | 0.052 | 0.34 | 1.200 |
| MH 18-6 | 339.802 | 19.614 | 1.545 | 1.287 | 73 | 0.228 | 0.302 | 0.202 | 0.065 | 0.42 | 1.513 |
| MH 19-5 | 413.426 | 14.710 | 1.376 | 1.146 | 65 | 0.243 | 0.317 | 0.205 | 0.077 | 0.51 | 4.000 |
| MH 19-6 | 413.426 | 6.865 | 1.227 | 1.023 | 58 | 0.243 | 0.330 | 0.214 | 0.099 | 0.65 | 7.350 |
| MH 20-5 | 382.277 | 14.710 | 1.376 | 1.146 | 65 | 0.237 | 0.313 | 0.206 | 0.072 | 0.48 | 4.601 |
| MH 20-6 | 382.277 | 9.807 | 1.227 | 1.023 | 58 | 0.237 | 0.325 | 0.213 | 0.095 | 0.63 | 6.534 |
| MH 21-5 | 339.802 | 15.691 | 1.376 | 1.146 | 65 | 0.229 | 0.291 | 0.194 | 0.072 | 0.47 | 1.816 |
| MH 21-6 | 339.802 | 8.826 | 1.227 | 1.023 | 58 | 0.229 | 0.302 | 0.202 | 0.101 | 0.66 | 3.436 |
| MH 22-5 | 283.168 | 15.691 | 1.376 | 1.146 | 65 | 0.219 | 0.260 | 0.177 | 0.057 | 0.38 | 1.450 |
| MH 22-6 | 283.168 | 8.826 | 1.227 | 1.023 | 58 | 0.219 | 0.261 | 0.178 | 0.070 | 0.46 | 2.350 |
| MH 27-1 | 311.485 | 9.807 | 1.227 | 1.023 | 58 | 0.261 | 0.439 | 0.274 | 0.170 | 1.12 | 21.000 |
| MH 27-2 | 311.485 | 24.518 | 1.545 | 1.287 | 73 | 0.261 | 0.437 | 0.273 | 0.125 | 0.82 | 12.500 |
| MH 27-3 | 311.485 | 34.325 | 1.693 | 1.411 | 80 | 0.261 | 0.448 | 0.280 | 0.113 | 0.74 | 3.500 |
| MH 35-1 | 424.753 | 9.807 | 1.227 | 1.023 | 58 | 0.271 | 0.549 | 0.336 | 0.229 | 1.50 | 36.101 |
| MH 35-2 | 424.753 | 19.614 | 1.545 | 1.287 | 73 | 0.238 | 0.563 | 0.369 | 0.178 | 1.17 | 20.100 |
| MH 35-3 | 424.753 | 44.132 | 1.841 | 1.534 | 87 | 0.256 | 0.585 | 0.369 | 0.134 | 0.88 | 13.500 |
Notes:
Table 27. Summary of experimental conditions and results for set 3 (effect of initial water content on pier scour in cohesive soils).
| Run ID |
Flow Discharge Q (l/s) |
Shear Strength S (kPa) |
Wet Density 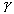 w w(t/m3) |
Dry Density 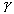 dry dry(t/m3) |
Compac- tion C (%) |
Depth Y (m) |
Velocity V (m/s) |
Froude No. Fr |
Scour Depth Dsc (m) |
Dimension- less Scour Dsc / b |
Scour Volume Vs (l) |
Dimension- less Volume Vs / b3 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| MH 23-6 | 413.426 | 9.807 | 1.481 | 1.287 | 73 | 0.247 | 0.330 | 0.212 | 0.079 | 0.52 | 12.800 | 3.616 |
| MH 24-5 | 382.277 | 6.865 | 1.176 | 1.023 | 58 | 0.237 | 0.313 | 0.206 | 0.117 | 0.77 | 14.290 | 4.037 |
| MH 24-6 | 382.277 | 9.807 | 1.481 | 1.287 | 73 | 0.237 | 0.325 | 0.213 | 0.082 | 0.54 | 11.500 | 3.249 |
| MH 25-4 | 339.802 | 14.710 | 1.623 | 1.411 | 80 | 0.229 | 0.284 | 0.189 | 0.085 | 0.39 | 3.150 | 0.890 |
| MH 25-5 | 339.802 | 9.807 | 1.481 | 1.287 | 73 | 0.229 | 0.291 | 0.194 | 0.066 | 0.43 | 5.999 | 1.695 |
| MH 25-6 | 339.802 | 6.865 | 1.176 | 1.023 | 58 | 0.229 | 0.302 | 0.201 | 0.113 | 0.74 | 7.700 | 2.175 |
| MH 26-4 | 283.168 | 14.710 | 1.623 | 1.411 | 80 | 0.219 | 0.236 | 0.161 | 0.037 | 0.24 | 1.000 | 0.283 |
| MH 26-5 | 283.168 | 9.807 | 1.481 | 1.287 | 73 | 0.219 | 0.260 | 0.177 | 0.047 | 0.31 | 1.450 | 0.410 |
| MH 26-6 | 283.168 | 6.865 | 1.176 | 1.023 | 58 | 0.219 | 0.261 | 0.178 | 0.056 | 0.37 | 1.999 | 0.565 |
| MH 33-1 | 311.485 | 6.865 | 1.176 | 1.023 | 58 | 0.259 | 0.439 | 0.275 | 0.187 | 1.23 | 30.000 | 8.476 |
| MH 33-2 | 311.485 | 9.807 | 1.481 | 1.287 | 73 | 0.259 | 0.437 | 0.274 | 0.130 | 0.85 | 18.750 | 5.297 |
| MH 33-3 | 311.485 | 17.653 | 1.764 | 1.534 | 87 | 0.261 | 0.448 | 0.280 | 0.094 | 0.62 | 9.999 | 2.285 |
Notes:
Table 28. Summary of experimental conditions and results for set 3 (effect of initial water content on pier scour for saturated clay).
| Run ID |
Flow Discharge Q (l/s) |
Initial Water Content IWC (%) |
Wet Density 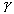 w w(t/m3) |
Dry Density 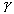 dry dry(t/m3) |
Compac- tion C (%) |
Approach Depth Y (m) |
Approach Velocity V (m/s) |
Froude No. Fr |
Scour Depth Dsc (m) |
Dimension- less Scour Dsc / b |
Scour Volume Vs (l) |
Dimension- less Volume Vs / b3 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| MH 13-4 | 311.5 | 32 | 2.002 | 1.517 | 86 | 0.245 | 0.224 | 0.145 | 0.000 | 0.00 | 00.000 | 0.000 |
| MH 14-4 | 283.2 | 35 | 1.952 | 1.446 | 82 | 0.216 | 0.240 | 0.165 | 0.000 | 0.00 | 00.000 | 0.000 |
| MH 15-4 | 339.8 | 35 | 1.952 | 1.446 | 82 | 0.228 | 0.264 | 0.177 | 0.000 | 0.00 | 00.000 | 0.000 |
| MH 16-4 | 413.4 | 35 | 1.952 | 1.446 | 82 | 0.250 | 0.301 | 0.192 | 0.000 | 0.00 | 00.000 | 0.000 |
| MH 17-4 | 283.2 | 40 | 1.852 | 1.323 | 75 | 0.216 | 0.240 | 0.165 | 0.000 | 0.00 | 00.000 | 0.000 |
| MH 18-4 | 339.8 | 42 | 1.778 | 1.252 | 71 | 0.228 | 0.264 | 0.177 | 0.000 | 0.00 | 00.000 | 0.000 |
| MH 19-4 | 413.4 | 45 | 1.688 | 1.164 | 66 | 0.243 | 0.304 | 0.197 | 0.000 | 0.00 | 00.000 | 0.000 |
| MH 23-1 | 413.4 | 48 | 1.644 | 1.111 | 63 | 0.243 | 0.326 | 0.211 | 0.147 | 0.96 | 04.900 | 1.384 |
| MH 24-1 | 382.3 | 40 | 1.852 | 1.323 | 75 | 0.230 | 0.319 | 0.212 | 0.057 | 0.37 | 01.000 | 0.283 |
| MH 25-1 | 339.8 | 48 | 1.644 | 1.111 | 63 | 0.224 | 0.279 | 0.188 | 0.000 | 0.00 | 00.000 | 0.000 |
| MH 28-1 | 518.2 | 32 | 2.002 | 1.517 | 86 | 0.299 | 0.628 | 0.367 | 0.000 | 0.00 | 00.000 | 0.000 |
| MH 28-2 | 518.2 | 38 | 1.922 | 1.393 | 79 | 0.293 | 0.633 | 0.374 | 0.064 | 0.42 | 00.900 | 0.254 |
| MH 28-3 | 518.2 | 43 | 1.791 | 1.252 | 71 | 0.293 | 0.675 | 0.398 | 0.104 | 0.68 | 02.701 | 0.763 |
| MH 29-2 | 792.9 | 38 | 1.922 | 1.393 | 79 | 0.308 | 0.867 | 0.499 | 0.134 | 0.88 | 02.801 | 0.791 |
| MH 29-3 | 792.9 | 43 | 1.791 | 1.252 | 71 | 0.308 | 0.877 | 0.504 | 0.165 | 1.08 | 09.808 | 2.771 |
| MH 34-1 | 424.8 | 35 | 1.952 | 1.446 | 82 | 0.271 | 0.549 | 0.336 | 0.058 | 0.38 | 01.100 | 0.311 |
| MH 34-2 | 424.8 | 40 | 1.852 | 1.323 | 75 | 0.265 | 0.563 | 0.349 | 0.076 | 0.50 | 01.650 | 0.466 |
| MH 34-3 | 424.8 | 45 | 1.688 | 1.164 | 66 | 0.256 | 0.585 | 0.369 | 0.116 | 0.76 | 03.092 | 0.874 |
| MH 36-1 | 501.2 | 35 | 1.952 | 1.446 | 82 | 0.290 | 0.619 | 0.367 | 0.049 | 0.32 | 01.139 | 0.322 |
| MH 36-2 | 501.2 | 40 | 1.852 | 1.323 | 75 | 0.280 | 0.666 | 0.402 | 0.101 | 0.66 | 03.454 | 0.975 |
| MH 36-3 | 501.2 | 45 | 1.688 | 1.164 | 66 | 0.262 | 0.713 | 0.445 | 0.165 | 1.08 | 05.578 | 1.576 |
| MH 37-1 | 736.2 | 35 | 1.952 | 1.446 | 82 | 0.317 | 0.759 | 0.430 | 0.070 | 0.53 | 03.300 | 0.932 |
| MH 37-2 | 736.2 | 40 | 1.852 | 1.323 | 75 | 0.314 | 0.828 | 0.472 | 0.145 | 0.95 | 08.000 | 2.260 |
| MH 37-3 | 736.2 | 45 | 1.688 | 1.164 | 66 | 0.317 | 0.893 | 0.506 | 0.192 | 1.26 | 11.148 | 3.150 |
| MH 38-1 | 948.6 | 35 | 1.952 | 1.446 | 82 | 0.427 | 0.867 | 0.424 | 0.081 | 0.46 | 01.811 | 0.512 |
| MH 38-2 | 948.6 | 40 | 1.852 | 1.323 | 75 | 0.402 | 0.915 | 0.460 | 0.114 | 0.75 | 04.000 | 1.130 |
| MH 38-3 | 948.6 | 45 | 1.688 | 1.164 | 66 | 0.378 | 0.967 | 0.502 | 0.192 | 1.26 | 13.000 | 3.673 |
| MH 39-1 | 96.6 | 32 | 2.002 | 1.517 | 86 | 0.059 | 1.341 | 1.760 | 0.100 | 0.99 | 02.925 | 2.789 |
| MH 40-1 | 78.6 | 32 | 2.002 | 1.517 | 86 | 0.057 | 1.088 | 1.450 | 0.096 | 0.95 | 02.550 | 2.431 |
| MH 41-1 | 55.2 | 32 | 2.002 | 1.517 | 86 | 0.051 | 0.959 | 1.360 | 0.066 | 0.65 | 01.319 | 1.258 |
| MH 42-1 | 64.6 | 32 | 2.002 | 1.517 | 86 | 0.055 | 0.991 | 1.349 | 0.069 | 0.68 | 01.291 | 1.231 |
| MH 43-1 | 89.2 | 32 | 2.002 | 1.517 | 86 | 0.058 | 1.259 | 1.660 | 0.097 | 0.95 | 03.441 | 3.281 |
| MH 44-1 | 78.4 | 32 | 2.002 | 1.517 | 86 | 0.112 | 0.686 | 0.660 | 0.037 | 0.36 | 00.750 | 0.715 |
Notes:
Dimensional analysis that has been used for correlating the variables affecting the local scour depth at bridge piers has been extended to include cohesive soil properties in order to account for the cohesive bed material. The variables used in the analysis are parameters defining the soil, the fluid, and the geometry of the modeled system. Depth of pier scour, Ds, which is the dependent variable in this analysis, can be expressed as a function of the following independent variables:
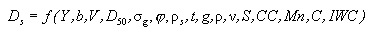 |
(30) |
in which Ds = depth of scour; Y = depth of approach flow; b = pier width; V = velocity of approach flow; D50 = mean sediment diameter; ![]() g = standard deviation of sediment size;
g = standard deviation of sediment size; ![]() = pier shape factor; rs=density of sediment particles; t = time; g = gravitational acceleration;
= pier shape factor; rs=density of sediment particles; t = time; g = gravitational acceleration; ![]() = fluid density; v = fluid kinematic viscosity; S = soil shear strength; CC = clay content; Mn = origin of clay minerals (e.g. Kaolinite, Illite, Montmorillonite); C = degree of compaction; and IWC = initial water content.
= fluid density; v = fluid kinematic viscosity; S = soil shear strength; CC = clay content; Mn = origin of clay minerals (e.g. Kaolinite, Illite, Montmorillonite); C = degree of compaction; and IWC = initial water content.
Applying the dimensional analysis using b, V, and r as repeating variables, and using appropriate simplifications, the following set of dimensionless parameters can be obtained:
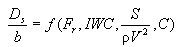 |
(31) |
in which Fr (= ![]() ). In deriving equation 31, the clay content (CC) was eliminated as a variable since, as shown in chapter 4, the effects of this parameter was found to be an independent factor only up to 12 percent clay content. In the cohesive pier scour experiments in Montmorillonite clays, the clay content was kept constant at 32 percent. In the experiments, the variation of scour with time was measured. This relationship was shown to be an asymptotic function with a sharp initial scour development followed by a gradual increase(26). The initial rate of scour hole development is generally controlled by the nature of the clay mineral and other cohesive material parameters such as compaction, initial water content, etc. Past experimental and theoretical studies have shown that the velocity at the nose region of a circular pier is amplified by a factor of 1.6 to 1.7 times the approach velocity, V. Accordingly, the bottom shear stresses that are related to V2 are also amplified and cause local scour in the affected zone. If approach velocities are increased beyond a threshold value defined as critical velocity, the entire approach channel bottom becomes subject to general scour in addition to the local scour. Under these conditions, the scour hole development process continues until equilibrium slopes are attained for the entire reach and may last indefinitely. The experimental study presented in this report limited itself to conditions in which the oncoming flows do not scour the approach reach (clear water conditions). Under these conditions, as soon as the scour hole reaches a depth where the shear stress within the base becomes equal to the critical shear stress of the cohesive material, local scouring ceases. The duration of experiments in the study were long enough to maintain the equilibrium condition for at least 4 hours. The final scour depth values obtained under these conditions are independent of time, and therefore in deriving equation 31 the time parameter, t, is eliminated.
). In deriving equation 31, the clay content (CC) was eliminated as a variable since, as shown in chapter 4, the effects of this parameter was found to be an independent factor only up to 12 percent clay content. In the cohesive pier scour experiments in Montmorillonite clays, the clay content was kept constant at 32 percent. In the experiments, the variation of scour with time was measured. This relationship was shown to be an asymptotic function with a sharp initial scour development followed by a gradual increase(26). The initial rate of scour hole development is generally controlled by the nature of the clay mineral and other cohesive material parameters such as compaction, initial water content, etc. Past experimental and theoretical studies have shown that the velocity at the nose region of a circular pier is amplified by a factor of 1.6 to 1.7 times the approach velocity, V. Accordingly, the bottom shear stresses that are related to V2 are also amplified and cause local scour in the affected zone. If approach velocities are increased beyond a threshold value defined as critical velocity, the entire approach channel bottom becomes subject to general scour in addition to the local scour. Under these conditions, the scour hole development process continues until equilibrium slopes are attained for the entire reach and may last indefinitely. The experimental study presented in this report limited itself to conditions in which the oncoming flows do not scour the approach reach (clear water conditions). Under these conditions, as soon as the scour hole reaches a depth where the shear stress within the base becomes equal to the critical shear stress of the cohesive material, local scouring ceases. The duration of experiments in the study were long enough to maintain the equilibrium condition for at least 4 hours. The final scour depth values obtained under these conditions are independent of time, and therefore in deriving equation 31 the time parameter, t, is eliminated.
Pier scour analysis in this study was conducted under two major categories: 1) unsaturated Montmorillonite clay scour and 2) saturated Montmorillonite clay scour. The distinction is made since for saturated cohesive materials, parameters such as Torvane shear strength and compaction have no physical significance; whereas these parameters are important in unsaturated cohesive material scour.
Unsaturated Conditions
The measured values of (Ds /b) were regressed against the remaining dimensionless groups in equation 31. The best-fit regression equation resulting from the statistical analysis of experimental data is:
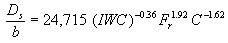 |
(32) |
where the initial water content (IWC) and compaction (C) are in percent. In deriving this expression, the initial water content ranged from 15 to 50 percent, and compaction ranged from 50 to 100 percent. The development of equation 32 is based on laboratory tests in which Froude numbers ranged from 0.18 to 0.37, and soil shear strength values ranged from 0.1 to 0.45 kg/cm2. The higher value of the correlation coefficient, R2 = 0.95, between the observed and predicted scour ratio indicates the strong correlation between measured scour depths and the parameters selected for defining flow and sediment properties. The plot of equation 32 with observed data is presented in figure 34. Pier scour corresponding to Froude numbers less than 0.2 and for compaction ratios higher than 85 percent is zero (scour threshold conditions).
Saturated Conditions
For saturated Montmorillonite clay soils, equation 31 can be simplified further by eliminating the dimensionless soil shear strength parameter, (S/![]() V2), since this term has no physical meaning for saturated clays at high initial water contents (it approaches to 0). Also, for saturated conditions the compaction of cohesive soils, C, is mainly related to the water content and can therefore be removed from the list of independent variables. Introducing the pier scour initiating Froude number, Fi, to define threshold conditions for pier scour and replacing Fr by the excess Froude number, (Fr-Fi), equation 31 becomes:
V2), since this term has no physical meaning for saturated clays at high initial water contents (it approaches to 0). Also, for saturated conditions the compaction of cohesive soils, C, is mainly related to the water content and can therefore be removed from the list of independent variables. Introducing the pier scour initiating Froude number, Fi, to define threshold conditions for pier scour and replacing Fr by the excess Froude number, (Fr-Fi), equation 31 becomes:
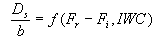 |
(33) |
Using the results of experimental study, Fi and Ds / b are determined as(25, 26):
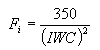 |
(34) |
and
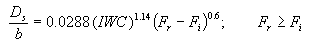 |
(35) |
For approach Froude numbers less than the pier scour initiating Froude number (i.e., Fr < Fi ), depth of scour is zero. For supercritical approach conditions, the value of experimental coefficient 0.0288 was found to be 0.0131. The plot of equation 35 with observed data is presented in figure 35.
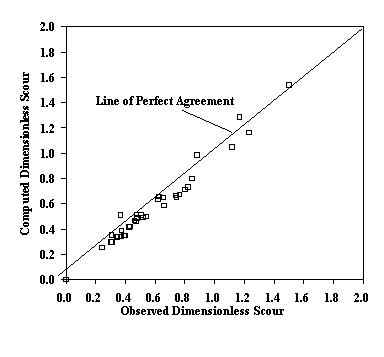
Figure 34. Computed and measured dimensionless pier scour depth for unsaturated Montmorillonite clay.
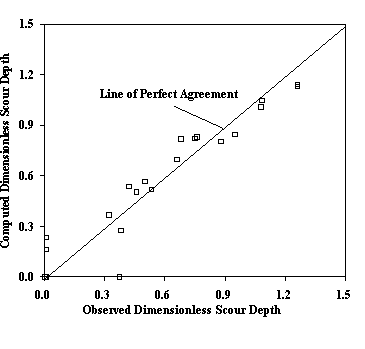
Figure 35. Computed and measured dimensionless pier scour depth for saturated Montmorillonite clay.
There is a distinction between pier scour taking place in unsaturated compacted clayey soils and saturated clayey soils. This differentiation affects parameters controlling local pier scour. For unsaturated compacted Montmorillonite clay soils, a new scour depth predictor is proposed in terms of initial water content, Froude number, soil shear strength, and degree of compaction. The pier scour depth and volume decrease as the compaction of cohesive soils increases. For saturated cohesive soils, the scour depth can be expressed as a function of initial water content and excess Froude number, (Fr-Fi). Under saturated conditions, the scour depth is directly proportional to excess Froude number and is inversely proportional to initial water content.