U.S. Department of Transportation
Federal Highway Administration
1200 New Jersey Avenue, SE
Washington, DC 20590
202-366-4000
Federal Highway Administration Research and Technology
Coordinating, Developing, and Delivering Highway Transportation Innovations
 |
| This report is an archived publication and may contain dated technical, contact, and link information |
|
Publication Number: FHWA-RD-98-155 Date: FEBRUARY 1999 |
Introduction |
|
To use the revised PRS prototype specification successfully, the agency must understand the implications of each decision made while developing or using the specification. These include general PRS decisions such as selecting the distress indicators used to define performance and the AQC's chosen to be included for acceptance, as well as more specific items such as defining appropriate values for the required constant variables. This chapter is designed to provide the typical user of the specification with the practical information required to make intelligent PRS-related decisions.
Defining Pavement Performance |
|
As previously stated, an underlying concept of a PRS is that knowledgeable decisions related to pavement lot acceptance and corresponding pay adjustments are based on the predicted pavement performance. In fact, the PRS is driven by key distress indicators. In the current PRS approach, pavement performance may be defined in terms of the development of any or all of the following distress indicators:
When developing a PRS for a given project, the agency must choose any or all of these distress indicators to define the pavement's performance. Those distress indicators not included in the chosen definition of performance will not be subjected to trigger values and, therefore, will not result in any responsive M & R activities or associated costs. For example, if transverse slab cracking is not included in the agency's definition of performance, slab replacement costs will not be incurred. It is recommended that all four of these distress indicators be used to define the pavement's performance.
Selection of Included AQCs |
|
Along with defining a pavement's performance by selecting distress indicators, the agency must also decide which AQC's are to be sampled and tested for acceptance. To make these selections, the agency must be aware of the interdependent relationships between distress indicator models and AQC's (these relationships are shown in figure 1). For example, if an agency includes transverse slab cracking in its definition of pavement performance, it is recommended that the agency choose to measure slab thickness and concrete strength since they are both key factors in the transverse slab cracking distress indicator model. If the agency were to choose not to sample an AQC that is required by a chosen distress indicator model, distress predictions would be made by holding that particular AQC at its target mean value. The revised PRS allows pay adjustments to be based on the measurement of any or all of the following five AQC's:
Based on the agency's selected definition of pavement performance, intelligent decisions must be made to define what AQC's are to be included in the PRS. It is recommended that the agency first identify those AQC's that are included in the selected distress indicator equations, as indicated above. From those included AQC's, the agency is encouraged to measure only those AQC's that it currently feels comfortable measuring. If this final selected list of AQC's greatly differs from AQC's required for the selected distress indicator models, it is recommended that the agency revisit its choices for included distress indicators. Because of the existence of these interdependent relationships between included distress indicator models and measured AQC's, the selection of both should be looked at as an iterative process.
Identification of Constant Variable Values |
|
Any developed PRS should be project-specific because
the predicted performance of any pavement is directly dependent on project-specific
variables. These variables fall into three categories: design-related
characteristics, climatic characteristics, and applied traffic loads.
Variables representing each of these three categories are used in the
agency-chosen distress indicator models to predict pavement performance.
These variables are labeled constant because they do not change
between the as-designed and as-constructed pavements. (Note: Figure
1 shows a list of the constant variables required by each distress
indicator model.)
Once the governing agency identifies the distress indicator models used
to define pavement performance and the AQC's to be accepted, the next
step is to assign values to the required constant variables that represent
the project's specific design, traffic, and climatic conditions. In an
effort to help agencies with the selection of appropriate constant variable
values, brief descriptions of each of the possible constant variables
are included below. The variables are divided into the three main categories
(design, climatic, and traffic) for clarification.
Pavement Type—Although the current specification is only valid for JPCP, the agency must identify whether the chosen pavement design is to be constructed with or without dowel bars.
Road Location—Calculated user costs (vehicle operating costs) are dependent on the roughness of the pavement over time, as well as the location of a road.(13) The road location setting is defined as either rural and urban.
Lane Configuration—Calculated user costs are also dependent on the identified lane configuration of a road.(13) The lane configuration is defined as a combination of road type (divided or undivided) and total number of lanes (includes all travel lanes in both directions). Divided pavements are those in which the traffic traveling in opposite directions is divided by a barrier or median. The current approach allows the agency to select from the following six different lane configuration choices:
- 2-lanes, undivided pavement.
- 4-lanes, undivided pavement.
- 4-lanes, divided pavement.
- 6-lanes, divided pavement.
- 8-lanes, divided pavement.
- 10-lanes, divided pavement.
Initial Design Life—The total expected amount of time for which the chosen pavement design is expected to carry traffic loads without the application of a global rehabilitation (AC overlay, PCC overlay, diamond grinding). This is the initial design life used in the common pavement design procedures.
Analysis Period—Period of time over which future M & R costs are to be considered in an LCC analysis. The analysis period is typically defined as twice the chosen design life (as a minimum) to ensure that future impacts of M & R are considered.
Lane Width—The agency must define the individual widths of each traffic lane. Typical pavements are constructed using traffic lanes that are 3.7 meters in width. However, many agencies are now constructing widened outer traffic lanes (typically 4.3 meters in width) in an attempt to reduce the development of transverse slab fatigue cracking. The defined lane widths are used to calculate sublot and lot areas. The presence of a widened outer traffic lane is an input required by the transverse joint faulting and transverse cracking models.
Average Transverse Joint Spacing—The transverse joint faulting, transverse joint spalling, and transverse slab cracking distress indicator models all have average transverse joint spacing as a required input. JPCP's are typically constructed using constant joint spacings (e.g., every 4.6 meters) or random joint spacings (e.g., using a repeated pattern of 4.0-4.3-4.6-5.2 m). If a constant joint spacing is used, the constant value is used as the average transverse joint spacing in the distress indicator models. If a random joint spacing is selected, the appropriate transverse joint spacing is set equal to the mean of the joint spacings included in the repeated series. For example, a random joint spacing series of 4.0-4.3-4.6-5.2 m would have a mean transverse joint spacing value of 4.5 m.
Effective Modulus of Subgrade Reaction (k-value)—An effective subgrade k-value is a required input for the transverse joint faulting and slab cracking distress indicator models. The appropriate value for use in these models is the effective modulus of subgrade reaction (including seasonal variations) calculated for design purposes. (Note: This value is for the subgrade, not the top of base.) Additional information on determining an appropriate effective subgrade k-value is contained in appendix HH of Volume 2 of the 1993 AASHTO Guide for Design of Pavement Structures.(14)
Base Type—The specified base type is a required input for the transverse joint faulting model. The current approach allows the agency to select from the following granular (unbound) and stabilized base types:
- None.
- Asphalt-treated aggregate.
- Cement-treated subgrade.
- Cement-treated aggregate.
- Crushed stone, gravel, or slag (untreated).
- Gravel (uncrushed).
- Lean concrete.
- Lime-treated subgrade.
- Limerock.
- Pozzolanic-aggregate mixture.
- Sand.
- Soil aggregate mixture.
- Soil cement.
Dowel Diameter—If the pavement is selected to be constructed with dowel bars, the agency must identify the dowel diameter. The dowel diameter is a required input in the transverse joint faulting distress indicator model and is expressed in units of mm.
Base Permeability Factor—Information regarding the permeability of the base material is required for the transverse joint faulting model. This model includes a base permeability dummy factor as an input. This dummy variable is set equal to 0 when the base is not considered to be permeable and is set equal to 1 when the base is considered to be permeable.
PCC Modulus of Elasticity—The transverse joint faulting model requires a value for the PCC modulus of elasticity. The appropriate modulus of elasticity is expressed in units of MPa.
Mean Annual Daily Average Temperature—The transverse slab cracking distress indicator model requires knowledge about the mean annual daily average temperature. Although the distress model does not require this variable as a direct input, it is used by the agency, along with the average annual total precipitation, to determine the climatic region in which the project exists. Mean annual daily average temperature data are readily available from most climatic databases.Table 3. Identification of climatic regions in the United States based on mean annual average daily temperature and average annual total precipitation.
Average Annual Total Precipitation—The average annual total precipitation is used in combination with the mean annual daily average temperature to determine the climatic region in which the project exists. Annual precipitation data are readily available from most climatic characteristic databases.
Average Daytime Thermal Gradient—An estimate of the average daily thermal gradient through the slab is a required input of the transverse slab cracking distress indicator model. An estimated thermal gradient value may be determined as a function of slab thickness and determined climatic region. The United States is divided into four climatic regions based on the mean annual daily average temperature and average annual total precipitation. The appropriate climatic region is determined using table 3. After determining the appropriate climatic region, an appropriate average daytime thermal gradient through the slab may be determined as a function of climatic region and design thickness using table 4.
|
Average Annual Total Precipitation |
Mean Annual Average Daily Temperature |
|
|---|---|---|
|
£ 13 ºC |
> 13 ºC |
|
£635 mm |
Dry-Freeze Region |
Dry-Nonfreeze Region |
> 635 mm |
Wet-Freeze Region |
Wet-Nonfreeze Region |
|
Average Daytime Thermal Gradient, ºF/in |
|||
|---|---|---|---|
|
Slab Thickness, mm |
Wet-Nonfreeze Climatic Region |
Dry/Wet-Freeze Climatic Regions |
Dry-Nonfreeze Climatic Region |
203 |
1.40 |
1.13 |
1.41 |
229 |
1.30 |
1.05 |
1.31 |
254 |
1.21 |
0.96 |
1.21 |
279 |
1.11 |
0.87 |
1.10 |
305 |
1.01 |
0.79 |
1.00 |
Note: The average daytime thermal gradients shown in this table are in units of ºF/in. The transverse slab cracking distress indicator model requires that the gradient be entered in these units.
1 ºC/cm = 4.572 ºF/in
Average Annual Number of Wet Days—The transverse joint faulting distress indicator model requires knowledge about the average annual number of wet days.Data for this variable are included in most available climatic databases.
Number of Days Above 90 ºF (32 ºC)—The average annual number of days in which the daily maximum temperature exceeds 90 ºF (32 ºC) is a climatic input required by the transverse joint faulting distress indicator model. Daily temperature data are readily available in most climatic databases.
Mean Freezing Index—The mean freezing index is a measure of the severity of frost for a region, expressed in terms of degree-days. The transverse joint faulting and spalling distress indicator models require the mean freezing index as a direct input. Although freezing index data are readily available in most climatic characteristic databases, values may be estimated for the continental United States using figure 13.
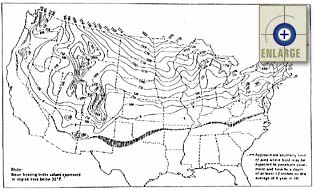 |
Figure 13. Distribution of mean freezing index values in the continental United States (Note: This chart is expressed in English units).(17) |
Annual Freeze-Thaw Cycles (at 7.6 cm below the surface)—The current transverse joint spalling distress indicator requires knowledge about the number of annual freeze-thaw cycles (FTC's) in the pavement (specifically, at 7.6 cm below the pavement's surface). It is to be noted that the number of "in pavement" FTC data is much different than the typically reported number of "air" FTC's. However, it has been found that the number of "in pavement" FTC's may be reasonably estimated from the measured daily maximum temperatures. One "in pavement" FTC may be assumed to occur whenever the measured daily maximum temperature stays at or below freezing (0º C) for at least 2 consecutive days (48 hours).
For example, if consecutive daily maximum temperatures were to read 4, 0, –1, 6 (all in ºC), this would constitute one "in pavement" FTC since the temperature stayed at or below freezing on days 2 and 3 (0 and –1 ºC, respectively). If the consecutive daily maximum temperatures were to read 2, 0, –1, –4, –8, 4 (all in ºC), this would still constitute only one "in pavement " FTC since the temperature stayed at or below freezing on days 2 through 5 (0, –1, –4 and –8 ºC, respectively). However, a series of consecutive daily maximum temperatures reading 2, 0, 5, –2, 5 (all in ºC), results in zero "in pavement" FTC's since the maximum daily temperature stayed at or below freezing for only 1 day (0 on day 2).
Transverse Joint Sealant Type—The transverse joint spalling model is dependent on the type of transverse joint sealant material selected for use on the pavement. In the current approach, the agency may choose from the following three sealant types:
- None (transverse joints are not sealed).
- Liquid asphalt sealant.
- Preformed compression seal.
Presence of Salt—Research in a previous study showed that the development rate of transverse joint spalling was significantly increased when salt (calcium chloride) was used for deicing purposes.(1) The presence of salt was, subsequently, included as an input in the current transverse joint spalling distress indicator model. The agency must, therefore, identify if salt (calcium chloride) is regularly used on the pavement.
Total Cumulative Design ESAL's—The transverse slab cracking and transverse joint faulting models are very much dependent on the number of ESAL's expected to be applied over the analysis life of the pavement.The current PRS approach requires knowledge about the total number of ESAL's expected to be applied in the pavement's first year of service or the total cumulative number of ESAL's to be applied over the pavement's design life. Either one of these values, in combination with information on the identified traffic growth pattern (discussed below), allows for the calculation of the ESAL's expected to be applied in every year of the pavement's analysis life. The current PRS approach also allows for the calculation of these yearly ESAL values based on known average daily traffic (ADT) information.
Traffic Growth Pattern—The agency must define the traffic growth rate and growth type for a given pavement project. The growth rate defines the annual increase in the applied yearly ESAL's (expressed as a percentage). The defined growth type determines how that percentage is applied and is selected by the agency as either simple or compound.
Simple Growth Type Method—If the agency selects a simple traffic growth type, the traffic is assumed to follow a linear relationship over time. Yearly ESAL values using the simple growth method may then be determined using equation 8.
whereESAL n = ESAL I + (n – 1) * [ESAL I * (Rate /100)] (8)
ESAL n = Estimated applied ESAL's at year n.
ESAL I = Initial ESAL's applied during year one of the pavement's service life.
n = Year of pavement service life for which ESAL's are being calculated.
Rate = Chosen traffic growth rate, expressed as a percentage (e.g., if 7.5% is the chosen growth rate, "Rate" = 7.5).
Compound Growth Type Method—If the agency selects a compound traffic growth type, each year's traffic increase is assumed to be calculated as a constant percentage of the previous year's applied ESAL's (compounded). Yearly ESAL values using the compound growth method may then be determined using equation 9.
whereESALn= ESAL I * [1 + (Rate /100)](n – 1) (9)
ESAL n = Estimated applied ESAL's at year n.
ESAL I = Initial ESAL's applied during year one of the pavement's service life.
n = Year of pavement service life for which ESAL's are being calculated.
Rate = Chosen traffic growth rate, expressed as a percentage (e.g., if 7.5% is the chosen growth rate, "Rate" = 7.5).
Selection of AQC Target Values |
|
Identifying the as-designed AQC target values (means and
standard deviations) is one of the most important tasks facing the governing agency.
These chosen target AQC's are used to define the agency's desired
pavement quality. In the current PRS approach, this desired quality is defined as
the quality for which the agency is willing to pay 100 percent of the contractor-submitted
bid price. If the contractor constructs a pavement that is measured to have better
AQC quality than that defined by the as-designed target AQC's, then (on average) the
contractor will receive an incentive payment (greater than 100 percent of the bid price).
Conversely, if the as-constructed AQC pavement quality is measured to be less than
that defined by the as-designed AQC target values, then (on average) the contractor will
receive a disincentive payment (less than 100 percent of the bid price).
Initially, it is recommended that the PRS quality levels
(AQC target values) be set to represent the quality levels specified under the agency's
current specifications. If appreciably lower quality levels are specified for the
target values, the result will be decreased performance. If appreciably higher
quality levels are specified, contract bid prices are likely to increase. While such
higher quality levels (with attendant higher bid prices) might well result in lower
overall LCC's, it would be difficult for an agency to justify increases in specified
quality without the agency first having some experience with PRS (i.e., knowledge of the
effect of specified quality on bid price and expected performance).
For an agency to select AQC target levels that represent the
quality levels currently being specified, the agency must have a thorough understanding of
its current specifications, and particularly its acceptance plans.The agency must
first identify the AQC quality levels (means and standard deviations) for which its
current acceptance plans are asking. Sometimes, these quality levels are not
obvious. In fact, the currently specified quality levels may be different than what the
agency actually desires (i.e., something other than what the agency intended to specify).
To identify exactly what quality level the agency is currently specifying, the
agency's expected payment (EP) curves should be utilized. The EP curve is a graphic
representation of an acceptance plan that shows the relation between the actual quality of
a lot and its expected pay.(4)That quality level (on an EP curve), for which the agency is
willing to pay 100 percent of the contractor-submitted bid price, should be interpreted as
the currently specified quality level for each AQC. If the agency has not developed
EP curves, computer programs are currently available (such as R.M. Weed's OCPLOT) to
assist the highway agency in their development.(18) If the agency acceptance plan cannot easily be converted to an EP
curve, it is suggested that the agency develop a new acceptance plan so that the SHA can
clearly determine the quality level currently being specified. The new acceptance
plan should, of course, consider the typical quality levels being achieved by contractors
in the State.
The agency-defined AQC target values are dependent on the
chosen AQC acceptance sampling and testing plan. The recommended AQC means and
standard deviations may change based on test type and number of replicate test results.
For example, if concrete strength were being measured using 28-day cylinder
compressive strengths, the AQC target values would be very different than those
recommended for the case where 28-day beams were being tested for flexural strength.
It is important to have knowledge of the proposed acceptance sampling and testing
plan prior to construction so that the appropriate AQC target values can be defined.
As stated previously, initially, the target means should be selected to
be equal to the AQC values for which the contractor would expect to receive
100 percent pay. Ideally, these values should correspond to the values
used for design purposes. Target standard deviations should be determined
by analyzing historical AQC data or by referring to published estimates
of standard deviations (appendix D has a number of recommended values
from the literature and field and laboratory testing conducted under this
project). If the agency has a reasonable amount of good historical data
representative of an AQC, it is strongly recommended that the agency analyze
these data to determine appropriate AQC target means and standard deviations.
The following steps can be used to determine target values based on historical
AQC construction data:
Although historical data should ideally be used to determine appropriate AQC target means and standard deviations, the initial recommendations for determining these values are summarized in table 5.
Table 5. Initial recommendations for determining AQC target means and standard deviations.
|
Acceptance Quality Characteristics |
Initial Recommendations for Target Mean |
Initial Recommendations for Target Standard Deviation |
|---|---|---|
Concrete Strength |
The target mean should be interpreted from a developed EP curve. This value should be equal to the current agency mean strength input used in the pavement design procedure (see note 1). |
These values should be based on historical project testing data. If no historical data are readily available, the values may initially be based on published data, or on the laboratory and field data collected under this research study (see appendix D). Both the variations due to testing and to materials should be included. See note 4. |
Slab Thickness |
The target mean should be interpreted from a developed EP curve. This value should be equal to the current agency design thickness determined from the pavement design procedure (see note 2). |
|
Entrained Air Content |
The target mean should be set equal to the current agency-designed entrained air content. This AQC is only significant in areas with significant freezing temperatures. |
|
Initial Smoothness |
The target mean should be interpreted from a developed EP curve. See note 3. |
|
Percent Consolidation Around Dowels |
The target mean should be determined from testing conducted by the agency to determine typical levels achieved, or from published data (see). |
Definition of Lots and Sublots |
|
It is important for the agency to clearly define lots and sublots when using the prototype PRS. The following definitions are recommended for use.
A lot is defined as a discrete quantity of as-constructed pavement to which an acceptance procedure (and corresponding pay adjustment) is applied. All pavement placed within a lot is assumed to have similar characteristics (e.g., traffic loadings, design properties, mix design, support, and representative as-designed AQC distributions). It is recommended that the lot length always be set equal to one day's production unless a significant within-day change occurs in one of the pavement characteristics (e.g., change in aggregate source or stoppage of the paving operations for a significant amount of time). If such a change does occur, it is recommended that a new lot be started at that point in the paving. The lot width is defined as the total width of pavement (one or more traffic lanes) being placed at one time in the mainline paving process. This paving width is also defined as a construction pass since it describes the total width of pavement being placed in one pass of the paving train. (Note: The width of a construction pass can change within a project depending on the number of lanes being paved at one time.) Shoulders are not included in the width of a lot; however, when applicable, the entire width of a widened traffic lane is to be considered as part of the mainline paving.
A sublot is simply defined as a portion of a lot. Each lot is divided into sublots of approximately equal surface area, based on the total linear amount of paving expected in the lot. More specifically, within the prototype PRS, sublots represent the smallest unit of pavement for which pavement performance is predicted (from distress indicators). Therefore, sublot lengths are defined so that all AQC's can be independently sampled from each defined sublot.
Selecting an Appropriate Target Sublot Length
Under the PRS approach, the sublot size is defined in terms of the linear length of longitudinal paving. The minimum sublot length should not be less than 0.16 km; this limit is required to accommodate the measurement of initial smoothness with a California profilograph. Although a maximum sublot length need not be defined, it is recommended that the agency try to select a sublot length that will result in a minimum of three sublots per lot. An appropriate maximum sublot length could then be defined as one-third of the total linear length of longitudinal paving expected for a typical pavement lot (one day of paving). An appropriate sublot length range for a given project can, therefore, be represented by the relationship presented as equation 10.
0.16 km £ PAVINGSUBLOT £ PAVINGSUBLOT-MAX 10
where
PAVINGSUBLOT = Chosen target sublot length for the project (expressed in km).
PAVINGSUBLOT-MAX = Recommended maximum sublot length (based on the recommended minimum of three sublots per lot). This value is calculated as PAVINGLOT/3 and expressed in km.
PAVINGLOT = Estimated total linear length of longitudinal paving expected for a typical pavement lot (expressed in km).
If the maximum sublot length (PAVINGSUBLOT-MAX) is calculated to be less than the chosen minimum sublot length (0.16 km), it is recommended that the chosen sublot length be set to the minimum of 0.16 km. Otherwise, it is recommended that the chosen sublot length be subjectively selected from the computed range (the range shown in equation 10) based on knowledge about the chosen acceptance sampling and testing plan. The agency must determine how many AQC samples and tests are to be taken from each sublot in the field. It is believed that the costs of conducting the required acceptance sampling and testing will greatly influence the selected sublot length. A discussion of the selection of an appropriate acceptance sampling and testing plan is contained in this chapter in the section titled Selecting an AQC Acceptance Sampling and Testing Plan.
Determining the Target Number of Sublots Per Lot
The target number of sublots per lot is estimated based on the agency-chosen target sublot length (PAVINGSUBLOT) and the estimated total linear length of longitudinal paving for a typical pavement lot (PAVINGLOT). This relationship is shown in equation 11.
NUMSUBSTARGET = PAVINGLOT / PAVINGSUBLOT (11)
where
NUMSUBSTARGET = Estimated target number of sublots per lot (based on the chosen target sublot length).
PAVINGLOT = Estimated total linear length of longitudinal paving expected for a typical pavement lot (expressed in km).
PAVINGSUBLOT = Chosen target sublot length (expressed in km).
As an example, if it were estimated that a contractor would pave 1.2 km per day (PAVINGLOT = 1.2 km) with a target sublot length of 0.32 km (PAVINGSUBLOT = 0.32 km), the target number of sublots per lot (NUMSUBSTARGET) would be calculated as the following (using equation 11).
NUMSUBSTARGET = PAVINGLOT / PAVINGSUBLOT
= 1.2 km / 0.32 km
= 3.75 sublots per lot
For this example, the typical number of sublots per lot would then be three or four. Therefore, the agency should develop preconstruction output for the cases of three and four sublots (as a minimum). More information on choosing appropriate ranges of numbers of sublots per lot is provided in the section titled Selecting Simulation Parameters.
Determining Actual Sublot Lengths in the Field
Since the amount of paving varies from lot to lot, a method
for dividing the as-constructed pavement lot into sublots is required. The following
defines this procedure.
Overall, it is recommended that the agency decide on a target sublot length that is practical for the amount and type of sampling and testing being conducted. This chosen target sublot length should then be used to lay out all the sublots prior to the paving of each lot. This can be done on a day-by-day (lot-by-lot) basis. Therefore, only the final sublot of each lot should differ from the chosen target sublot length.
Selecting an AQC Acceptance Sampling and Testing Plan |
|
Acceptance of the as-constructed pavement is based on the field sampling of all of the included AQC's chosen by the agency. The PRS approach ideally focuses on using in situ samples for acceptance, since these samples provide a true indication of the properties of the as-constructed pavement. Better estimates of the in situ quality will ideally lead to better estimates of future pavement performance. Although the current movement is toward in situ sampling, any agency-approved sampling and testing methods may be utilized as long as they are performed in accordance with one of the following standard specifications:
An agency should, therefore, be able to implement the PRS prototype approach without
making major changes to its current sampling methods.
There is also a current movement toward obtaining early age
AQC test results to provide the contractor with quick feedback regarding the measured
as-constructed pavement quality. Any test method chosen for use in a PRS should
ideally be timely, economical, nondestructive, reliable, and reproducible. Although
the current PRS approach does not require the use of such early age testing methods, they
should be considered where appropriate.
Available AQC Sampling and Testing Procedures
The following sections discuss the currently recommended AQC
sampling and testing methods and their use within the PRS approach. Until the agency
gains adequate experience with the current PRS approach and its procedures, it is strongly
recommended that the agency continue to use AQC sampling and testing procedures with which
it feels completely comfortable.
Concrete Strength
Three of the distress indicator models (transverse joint
faulting, transverse fatigue cracking, and transverse joint spalling) included in the
current PRS approach require concrete strength as an input. Specifically, these
models require a 28-day (equivalent laboratory maturity) flexural strength using a
third-point loading. Therefore, the agency must choose a sampling and testing
procedure that results in a direct measurement or indirect estimation of this required
28-day flexural strength.
Traditionally, the 28-day flexural strength has been
measured directly by conducting flexural strength testing on beams at 28 days of
equivalent laboratory maturity. However, most agencies have abandoned the practice
of using beams for acceptance and have concentrated on easier, more practical methods of
estimating strength (such as 28-day cylinder compressive strength). In addition, there has
been a movement toward accepting concrete strength based on early age (3-, 7-, or 14-day)
strength tests. The selection of a concrete strength sampling and testing plan
requires that the agency decide on a sampling type, the timing of sampling, a test type,
and the timing of testing. Some of the more typical concrete strength sampling and testing
choices available to an agency are described in more detail below.
Sampling Specimen Types for Concrete Strength
It is recommended that the sample concrete strength be measured using beams, cylinders, or cores. If beams or cylinders are selected, these samples are cast at the time of construction. If cores are selected, they are to be extracted from the pavement at an agency-chosen time between 3 and 28 days of equivalent laboratory maturity. The details of each sampling type are presented in the following sections.
Beams. Beam specimens (with agency-specified dimensions) are
to be molded, handled, and cured in accordance with AASHTO T-23, Making and Curing
Concrete Test Specimens in the Field.(19) Beam specimens for each sublot are made with plastic concrete taken
from in front of or behind the paver at predetermined random longitudinal sampling
locations. Random longitudinal sampling locations are identified in accordance with
the guidelines set forth in the section titled Selecting a Random
Longitudinal Sampling Location. The number of beam specimens per sampling
location (replicate specimens) and the number of sampling locations per sublot are defined
by the agency in accordance with the guidelines set forth in the section titled Recommended Number of AQC Samples
Per Sublot.
Cylinders. Cylinder specimens are molded, handled, and cured in accordance
with AASHTO T-23, Making and Curing Concrete Test Specimens in the Field.(19) All
cylinder specimens are cast in molds with a nominal length-to-diameter ratio of 2.
An appropriate cylinder specimen diameter is determined based on the following:
- A minimum 102-mm cylinder diameter is used when the maximum aggregate size is 32 mm or less.
- A minimum 152-mm cylinder diameter is used when the maximum aggregate size is greater than 32 mm.
- A minimum 102-mm diameter is used when the maximum aggregate size is 32 mm or less.
- A minimum 152-mm diameter is used when the maximum aggregate size is greater than 32 mm.
Prior to testing, all core specimens are trimmed to a nominal length-to-diameter ratio of 2.; A correction factor is applied (in accordance with AASHTO T-24, Obtaining and Testing Drilled Cores and Sawed Beams of Concrete) to cores having a length-to-diameter ratio of less than 1.94, whereas cores having a length-to-diameter ratio between 1.94 and 2.10 require no such correction.(19) Cores with a length-to-diameter ratio exceeding 2.10 are reduced in length to fall within the ratio limits of 1.94 to 2.10.
Timing of Concrete Strength Testing
The agency must define the timing of the concrete strength
specimen testing to be used in estimating the 28-day (equivalent 28-day laboratory
maturity) flexural strength (third-point loading) of the as-constructed pavement.
Each specimen (molded beam, molded cylinder, or extracted core) is tested independently
and translated (if necessary) into an MR at an equivalent 28-day
laboratory maturity.
The timing of concrete strength specimen testing is defined
by the agency and expressed in terms of an equivalent laboratory maturity. The
agency-chosen timing of concrete strength specimen testing must meet the following
requirements:
Testing-Related Procedures (Conducted Prior to Specimen Testing)
The agency must perform the following testing-related laboratory and field procedures (as required) in preparation for concrete strength specimen testing.
Development of Mix-Specific Maturity Curves
Prior to the placement of any as-constructed pavement, the
agency must develop required mix-specific maturity curves in the laboratory. The
representative maturity curves (expressed as flexural, compressive, or split-tensile
strength versus maturity) are determined using the Arrhenius maturity method and in
accordance with ASTM C-1074, Standard Practice for Estimating Concrete Strength by the
Maturity Method.(20) The required developed maturity curves (and corresponding
equations) are included as Series A attachments to the specification (see appendix A in this volume). This laboratory maturity
calibration is only required if sample testing is conducted when the equivalent laboratory
maturity is less than 28 days.
These mix-specific strength to maturity relationships are
determined prior to construction by the testing laboratory contracted to develop the
concrete mixture design. The following procedure is used by the laboratory personnel
to develop these required relationships.
- Compressive strength of cylinders—AASHTO T-22, Compressive Strength of Cylindrical Concrete Specimens.(19)
- Split-tensile strength of cylinders—AASHTO T-198, Splitting Tensile Strength of Cylindrical Concrete Specimens.(19)
- Flexural strength (third-point loading) of beams—AASHTO T-97, Flexural Strength of Concrete (Using Simple Beam With Third-Point Loading).(19)
The number of testing ages should be sufficient to enable the user to confidently predict strength from maturity. As a minimum, it is recommended that three specimens be tested (for each strength type) at equivalent laboratory maturities of 1, 3, 5, 7, 14, and 28 days.
Development of Mix-Specific Inter-Strength Relationships
Prior to the placement of any as-constructed pavement, the
agency must also develop required mix-specific inter-strength relationships (i.e.,
compressive-to-flexural-strength, and/or split-tensile-to-flexural strength relationships)
in the laboratory. These specific inter-strength relationships are determined by
comparing the different 28-day maturity strength values measured for the development of
the appropriate maturity curves. The required inter-strength relationships (curves
and equations) are included as Series B attachments to the PRS (see appendix A in this volume).
It is recommended that the compressive-to-flexural-strength
equation be assumed to have the following form:
MR(28-day) = A * (f'C(28-day)0.5) (12)
where
MR(28-day) = Estimated flexural strength (third-point loading) at a 28-day equivalent laboratory maturity, MPa.
f'C(28-day) = Estimated compressive strength at a 28-day equivalent laboratory maturity, MPa.
A = Mix-specific coefficient determined through calibration using the means of the measured compressive and flexural strength data at a 28-day equivalent laboratory maturity.
The mix-specific coefficient (A) is determined using the computed compressive and
flexural strength 28-day maturity sample means (used in the development of the maturity
curves). Equation 12 is solved for A using these computed sample means.
Although the equation form shown in equation 12 is recommended, any agency-accepted
equation relating compressive to flexural strength may be used.
It is recommended that the split-tensile-to-flexural-strength equation be assumed to have
the following form:
MR(28-day) = A * (ST(28-day)/B)0.75 (13)
where
MR(28-day) = Estimated flexural strength (third-point loading) at a 28-day equivalent laboratory maturity, MPa.
ST(28-day) = Estimated split-tensile strength at a 28-day equivalent laboratory maturity, MPa.
A = Mix-specific coefficient determined through calibration using the means of the measured compressive and flexural strength data (at a 28-day equivalent laboratory maturity) in equation 12.
B = Mix-specific coefficient determined through calibration using the means of the measured split-tensile and flexural strength data (at a 28-day equivalent laboratory maturity).
The mix-specific coefficient (B) is determined using the computed split-tensile and flexural strength 28-day maturity sample means (used in the development of the maturity curves). Equation 13 is solved for B, by knowing A and using these computed sample means.
Specific Concrete Strength Testing Procedures
Representative flexural strength (third-point loading) values at a 28-day equivalent laboratory maturity are determined for each specimen using one of the following three testing procedures.
Measuring Maturity in the As-Constructed Pavement
If the agency selects core specimens as the sampling type, the maturity of the as-constructed pavement is monitored for each sublot. Temperatures are measured at one central location per sublot using a thermocouple placed at mid-depth of the pavement slab (the thermocouple is embedded into the pavement using an agency-approved method). The thermocouple is connected to an agency-approved maturity meter. The maturity meter should begin recording pavement temperatures at the time when the thermocouple becomes completely covered with concrete. Temperatures are measured for a given sublot until all of the cores representing the sublot are extracted from the as-constructed pavement.
As stated earlier, the strength measured for each sampled
concrete strength specimen must be translated into an equivalent 28-day (28 days of
equivalent laboratory maturity) flexural strength. The procedures for estimating
this 28-day flexural strength differ depending on the agency-chosen sample type, test
type, and timing of testing. The general procedure used to determine an appropriate
equivalent 28-day flexural strength value (for a given sample specimen) involves using the
following procedures. All testing is conducted using the agency-chosen testing
method.
DIFFMAT(A) = [STRMEASURED- MAT(A) – STREXPECTED- MAT(A)](14)
where
DIFFMAT(A) = Computed difference between the measured strength value and the expected strength value. Strength is measured in units of MPa.
STRMEASURED- MAT(A) = Measured strength value at the agency-selected equivalent laboratory maturity. Strength is measured in units of MPa.
STREXPECTED- MAT(A) = Expected strength value (from the appropriate maturity curve) at the agency-selected equivalent laboratory maturity. Strength is measured in units of MPa.
STRESTIMATED-MAT(28 days) = [STREXPECTED- MAT(28 days) + DIFFMAT(A)] (15)
where
STRESTIMATED- MAT(28 days) = Estimated equivalent 28-day strength value (strength type is the same as the agency-chosen testing type) at an equivalent laboratory maturity of 28 days. Strength is measured in units of MPa.
STREXPECTED- MAT(28 days) = Expected strength value (from the appropriate maturity curve) at an equivalent laboratory maturity of 28 days. Strength is measured in units of MPa.
DIFFMAT(A) = Computed difference between the measured strength value and the expected strength value. Strength is measured in units of MPa.
Figure 14 shows an example of translating from a measured strength value to an equivalent 28-day maturity value of the same strength type (i.e., the procedure outlined in these first five steps).
 |
Figure 14. Example of using a developed maturity curve to translate from a measured strength value to a representative 28-day expected strength value. |
Finally, the mean of all of the estimated equivalent 28-day flexural strength values
(representing each sample specimen from one sample location) is computed and used to
represent that sample location. Some of the steps of the procedure may not be
required, depending on the agency-chosen sampling and testing procedures.
Slab Thickness
Two of the distress indicator models (transverse joint
faulting and transverse fatigue cracking) included in the current PRS approach require
slab thickness as an input. The thickness of the as-constructed pavement is
determined by measurements taken on cores extracted from each sublot making up an
as-constructed pavement lot. Core specimens are extracted from the hardened pavement
slab between 48 and 72 hours after placement, in accordance with AASHTO T-24, Obtaining
and Testing Drilled Cores and Sawed Beams of Concrete.(19) All cores used for
the acceptance of slab thickness must have a minimum diameter of 102 mm. The
representative thickness of each core is determined in accordance with AASHTO T-148, Measuring
Length of Drilled Concrete Cores.(19)
Slab thickness is measured on all cores extracted for the
evaluation of concrete strength; these measured values are used in lieu of extracting
additional slab thickness cores. When required, randomly selected slab thickness
core locations (independent of any cores taken for the evaluation of concrete strength)
are determined in accordance with the section titled Selecting a Random Core Location.
The number of slab thickness core specimens per sublot is defined by the agency in
accordance with the guidelines set forth in the section titled Recommended Number of AQC Samples
Per Sublot.
Entrained Air Content
The measured entrained air content of the pavement slab is
included as an optional input in the transverse joint spalling model included in the
current PRS approach. Although it is officially considered optional, it is
recommended that the agency choose to measure entrained air content if transverse joint
spalling is to be included in the definition of pavement performance. If entrained
air content is selected for measurement in the field, the following sampling and testing
procedures apply.
The entrained air content of the as-constructed pavement is
determined using one of the following agency-approved sampling and testing methods:
Initial Smoothness
The two smoothness-related distress indicator models (PSR
and IRI) included in the current PRS approach require initial smoothness as an input.
The PSR distress indicator model requires an initial PSR, whereas the IRI model
requires an initial IRI. The selection of an initial smoothness sampling and testing
plan requires that the agency answer the following three questions:
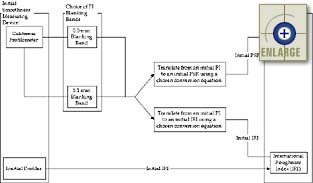 |
Figure 15. Flow chart showing the available methods for handling smoothness under the PRS approach. |
If a profiling device is used to measure initial IRI, measurement is made in accordance
with provided agency-approved procedures.
Regardless of the chosen sampling method, a minimum of two
pavement profiles (one in each wheelpath) must be measured for each lane within each
defined sublot. The total number of required pavement profiles per sublot is defined
by the agency in accordance with the guidelines set forth in the section titled Recommended Number of AQC Samples
Per Sublot. The location of a wheelpath is 0.92 m from a longitudinal
joint or longitudinal pavement edge and parallel to the centerline of the mainline
paving. For widened traffic lanes, the outer wheelpath is 0.92 m from the
pavement-edge paint stripe, rather than the outer pavement edge. Each profile should
terminate 4.5 m from each bridge approach pavement or existing pavement that is joined by
the new pavement. The PI or IRI determined for each profile is converted to a
standard unit of mm/km.
During the initial paving operations, or after a long
shutdown period, the pavement surface must be tested with the appropriate profile
measuring device. Membrane curing damaged during the testing operation must be
repaired by the contractor and at the contractor's expense. If the initial pavement
smoothness, paving methods, and paving equipment are acceptable, paving operations may
proceed. After initial testing, profiles of each day's paving will be run prior
to continuing paving operations.
The agency will apply the following appropriate limits based
on the chosen initial smoothness indicator (PI or IRI):
If an average PI / IRI of _____ mm/km [limit to be inserted by the agency] is exceeded in any daily paving operation, the paving operation will be suspended and not resume until corrective action is taken.
Within each sublot, all areas represented by high points having deviations in excess of
_____ mm (limit to be inserted by the agency—10 mm is recommended) in 7.6 m or less
must be corrected at the contractor's expense. Corrections must be made using an
approved profiling device or by removing and replacing the pavement, as directed by the
engineer. Bush hammers or other impact devices must not be used. Where
corrections are made, the surface texture is re-established to provide a uniform texture
equal to the surrounding uncorrected pavement by the contractor and at the contractor's
expense.
Choosing an Appropriate Blanking Band for Computing Profile Index
When measuring initial smoothness using a California
profilograph, the resulting profilograms may be reduced using a 0.0- or 5.1-mm blanking
band. Currently, most SHA's measure initial smoothness in terms of PI5.1-mm;
however, more agencies are starting to move toward using PI0.0-mm.
As mentioned previously, it is strongly recommended to use the PI0.0-mm
as it provides better control over initial smoothness.
Converting From a Profile Index to an Initial PSR or IRI
Under the PRS approach, if an agency decides to measure
initial smoothness in terms of PI0.0-mm, the agency must develop
their own conversion equation from PI0.0-mm to initial PSR or
IRI. Unfortunately, there is little published information regarding such conversion
equations. If the agency does not wish to develop a relationship based on PI0.0-mm,
initial smoothness may be measured in terms of PI5.1-mm and
converted to an initial PSR or IRI using an available conversion equation. Many such
relationships have been developed under past research. Some of the currently
available PI5.1-mm to initial PSR and PI5.1-mm
to initial IRI relationships are summarized in tables 6 and 7. The agency-chosen
relationship is an input in the PaveSpec 2.0 computer software.
Table 6. Published relationships between measured PI (using a 5.1-mm blanking band) and initial PSR.(21)
Equation |
Source |
|---|---|
PSR = 5.0 – 0.0714(PI5.1-mm) |
Darter et al.(2) |
PSI = –0.04762(PI5.1-mm) + 4.443 |
Walker and Lin(22) |
PSI = 4.06 – 0.0256(PI5.1-mm) |
Uddin, Hudson, and Elkins(23) |
Note: Present serviceability index (PSI) is used interchangeably with PSR. Also, the PI required by these equations is expressed in units of in/mi.
Table 7. Published relationships between measured PI (using a 5.1-mm blanking band) and initial IRI.
|
Equation |
Source |
|---|---|
IRI = 52.9 + 6.0(PI5.1-mm) |
Kombe and Kaleva(24) |
IRI = 73.7 + 2.83(PI5.1-mm) |
Kombe and Kaleva(24) |
PIa.p. = –22.3 + 0.3(IRI) |
Goulias, Dossey, and Hudson(25) |
IRI = 36.4 + 3.11(PI5.1-mm)(for PI=5-7 in/mi) |
Kulakowski and Wambold(26) |
Note: The PI required by these equations is expressed in
units of in/mi.
1.0 in/mi = 15.78 mm/km
Measuring Initial Smoothness in Terms of IRI
If the agency chooses to measure initial smoothness in terms
of IRI (directly), the only available option is to predict smoothness over time in terms
of IRI (see figure 15). Although there are currently no
reliable methods for measuring initial IRI on newly placed concrete, research continues in
this area. In anticipation of the development of such an initial IRI measurement
method, this option was included in the revised PRS prototype.
Percent Consolidation Around Dowels
The measured percent consolidation around dowels is included
as an optional input in the transverse joint faulting model included in the current PRS
approach. If percent consolidation around dowels is selected for measurement in the
field, the following sampling and testing procedures apply.
The representative percent consolidation around one randomly
selected dowel bar in a sublot is determined based on a comparison of the density of two
selected cores extracted from the hardened concrete slab. Core specimens are
extracted from the hardened pavement slab between 48 and 72 hours after placement, in
accordance with AASHTO T-24, Obtaining and Testing Drilled Cores and Sawed Beams of
Concrete.(19) All cores used for the acceptance of percent consolidation around
dowels must have a minimum diameter of 102 mm.
The first of the two required cores is taken through a
predetermined randomly selected dowel bar in a randomly selected transverse joint (random
sampling core locations are determined in accordance with the guidelines set forth in the
section titled Selecting a Random Dowel
Location). The outside edge of the core through the randomly selected dowel
bar must not be within 60 mm of a defined wheelpath or pavement edge, 100 mm of a vibrator
path, or 50 mm of a transverse joint. The dowel bar piece is separated from the
concrete core material by an agency-approved method. The density of this concrete
material is measured in a saturated surface dried condition in accordance with ASTM C-642,
Standard Test Method for Specific Gravity, Absorption, and Voids in Hardened Concrete(20), and
labeled as DENTHROUGH-DOWEL(n).
The second of the two required cores is taken at a location
along a line passing through the first core (through the dowel bar) and parallel to the
centerline of the pavement unit. The specific longitudinal location of this second
core is assumed to be at midslab of the leave slab (the slab away from the joint in the
direction of traffic) adjacent to the randomly selected transverse joint. The
density of this concrete material is measured in a saturated surface dried condition in
accordance with ASTM C-642, Standard Test Method for Specific Gravity, Absorption, and
Voids in Hardened Concrete(20), and labeled as the DENMID-SLAB(n).
The number of samples per sublot (i.e., pairs of cores) is
defined by the agency in accordance with the guidelines set forth in the section titled Recommended Number of AQC Samples
Per Sublot. The maximum of all of the midslab core densities measured within
the given lot (MAX-DENMID-SLAB) is determined and assumed to represent the
density of a core with 100 percent consolidation. The representative percent
consolidation for each sampling location (set of cores) is, therefore, determined using
equation 16.
%Consolidation=(DENTHROUGH-DOWEL(n) /MAX-DENMID-SLAB)*100 (16)
Additional sampling and testing for any of the AQC's
for acceptance testing may be requested at any time by the contractor or by the agency.
Retesting is, however, required if any AQC test results are found to be of
lesser quality than agency-defined rejectable quality limits (RQL's). These
limits define the minimum quality allowed to stay in place (and subjected to a pay
adjustment) on the as-constructed pavement. For concrete strength, slab thickness,
air content, and percent consolidation around dowels, retesting is required if the
measured sample value is less than the defined RQL; however, for initial
smoothness, retesting is required if the measured sample value is greater than the
defined RQL.
At the same time, the agency defines maximum quality limits
(MQL's) to be applied to each AQC specimen sample value. The MQL's define
the limit on the additional quality (beyond the target values) for which the agency is
willing to pay an incentive. Therefore, if a specimen sample value is measured to be
of greater quality than the defined MQL, the representative specimen sample value (used in
the acceptance procedures) is set equal to the defined MQL (i.e., the contractor will not
receive credit for quality provided in excess of the MQL). For concrete strength,
slab thickness, air content, and percent consolidation around dowels, the MQL is an upper
limit on quality; however, for initial smoothness, the MQL is a lower limit on
quality.
The purpose of retesting is to determine if the AQC quality
provided by the contractor is truly less than the quality defined by the RQL. If
retesting procedures determine conclusively that an identified area of pavement is
deficient in quality (having lesser quality than the respective agency-defined RQL), the
result will be the removal and replacement of the identified area. AQC sampling and
testing values from the replaced material is then used in replacement of the original
sampling and testing results for that sampling location. However, the AQC samples
taken from the replaced material are subjected to MQL's equal to the respective AQC
target values (i.e., the contractor may not get credit for AQC quality better than the
target values when material has been removed and replaced).
If retesting procedures determine conclusively that an
identified area of pavement is not deficient in quality (having greater quality than the
respective agency-defined RQL), the additional AQC samples taken for retesting are added
to the original AQC sample value set. The average of all the AQC sampling and
testing results (original and retesting) representing a sampling location is then used as
the representative AQC value for that sampling location. If the contractor and
agency agree that a testing error occurred when determining an AQC test value, this test
value is excluded from the acceptance process.
Selection of Appropriate RQL's and MQL's
The agency should choose RQL and MQL values carefully, so
they truly define the range of AQC quality that the agency desires. The selected
RQL's should identify the absolute minimum quality that the agency will accept as
part of the as-constructed pavement. Any lesser quality pavement will be removed and
replaced. The selected MQL's should identify the absolute maximum quality for
which the agency is willing to pay an incentive. These quality limits provide the
agency with a method of defining practical limits that keep the focus of the contractor on
the as-designed target quality values. In turn, the contractor knows exactly how
much additional quality he can provide (in excess of the AQC target values) and still
expect to get paid an incentive. Again, it is important to stress that under the PRS
approach, the AQC target values represent the true agency-desired quality.
All AQC test values that are measured to be between the
agency-defined RQL and MQL will be kept in place and used for acceptance. Pay
adjustments are determined and based on this measured AQC quality.
Specific Retesting Responsibilities
The specific retesting procedures are identified by the
agency and provided as part of the specification. The agency will conduct all of the
sampling and testing for any retesting activities. The conditions for determining
whether the agency or contractor is responsible for the cost of the retesting will be
determined in accordance with the specific retesting procedures provided in the
specification. The pavement may only be retested once in accordance with the
retesting methods provided in the specification. The sublot retesting sampling
locations are determined in accordance with the guidelines set forth in the section titled
Selection of Random Sampling
Locations.
Recommended Number of AQC Samples Per Sublot
The number of AQC samples per sublot has a large effect on the risks to the agency and contractor. The recommended number of samples per sublot (for each AQC) is specific to the chosen sampling and testing method. Figure 16 shows the effect of the number of samples per sublot on the as-designed present worth LCC standard deviation for a typical example (3 sublots per lot, 500 lots per simulation). Each point on the chart represents the standard deviation of 500 simulated lot LCC's. This computed standard deviation is an indicator of the risks or uncertainty involved in estimating the LCC from small sample sizes.
 |
Figure 16. Example showing the typical effects of number of samples per sublot on the LCC standard deviation (3 sublots per lot, 500 lots per simulated point) |
The points making up the chart in figure 16 were simulated
by setting four different AQC's (concrete strength, slab thickness, entrained air
content, and initial smoothness; percent consolidation around dowels was not included in
this example) equal to their target means and standard deviations. At each
simulation point, all of the included AQC's used the sample size reflected in the
chart (i.e., for the simulated point where n = 10, 10 samples per sublot were taken for
each of the four included AQC's).
Figure 16 shows that there is great benefit of using more
than one sample per sublot. It also shows that the LCC standard deviation tends to
stabilize for sample sizes greater than or equal to five. Therefore, it is
recommended that sample sizes of two to five samples per sublot be used for acceptance in
the field. It is, however, recognized that it may not be practical to take three to
five samples per sublot for all AQC sampling types (e.g., linear traverse testing for
entrained air content). Hence, it is recommended that the agency select AQC sample
size by balancing the risk of errors in quality determination with the practicality and
cost of sampling and testing. It is important to note that regardless of the number
of samples per sublot chosen for each AQC, the selected number of AQC samples per sublot
will be the same for both as-constructed and as-designed simulations. Therefore, the
same effects of simulation error or bias on the simulated LCC's will be present in
both the as-constructed and as-designed representative LCC means. Table 8 shows
recommended minimum number of samples per lot for different AQC sampling and testing
types.
AQC |
Sample Type |
Testing Type |
Recommended Minimum Number of Sampling Locations per Sublot, n |
Recommended Minimum Number of Replicate Samples per Sampling Location |
|---|---|---|---|---|
Concrete Strength |
Beams |
28-day modulus of rupture |
2–5 |
2 |
Early age modulus of rupture |
||||
Cylinders |
28-day compressive |
2–5 |
2 |
|
Early age compressive |
||||
8-day split-tensile |
||||
Early age split-tensile |
||||
Cores |
28-day compressive |
2–5 |
N/A |
|
Early age compressive |
||||
28-day split-tensile |
||||
Early age split-tensile |
||||
Slab Thickness |
Cores |
N/A |
2–5 |
N/A |
Entrained Air Content |
Fresh Concrete |
Air pressure meter |
3–5 |
2 |
Cores |
Linear traverse |
1–3 |
N/A |
|
Initial Smoothness |
Profile Index |
California profilograph |
2 times the number of lanes (inner and outer wheelpaths of each lane) |
None |
IRI |
Inertial profiler |
2 times the number of lanes (inner and outer wheelpaths of each lane) |
None |
|
Percent Consolidation Around Dowels |
Pairs of Cores |
Relative density comparison |
1–3 |
None |
Note: IRI = international roughness index
Selection of Random Sampling Locations
Selecting a Random Longitudinal Sampling Location
Random longitudinal sampling locations need to be identified whenever concrete strength or entrained air content is determined using samples of plastic concrete. Plastic concrete will be taken from in front of the paver (or behind the paver for air content, if so desired) at every randomly determined longitudinal sampling location. These estimated sampling locations are determined for each sublot in a given lot prior to the start of the paving of that lot (typically, these longitudinal sampling locations are determined on a day-to-day basis, before the start of that day's paving). Each specific required longitudinal sampling location (within a given sublot) may be determined using the following procedures:
As an example, let us assume that we have a project with a target sublot length of 200 m (PAVINGSUBLOT = 200 m). A random number is generated to be 0.422 using an agency-approved random generation method. Therefore, the longitudinal offset from the sublot's starting station is computed as 200 m * 0.422 = 84.4 m. This example is illustrated in figure 17.
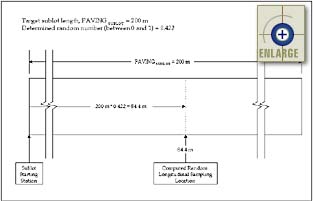 |
Figure 17. Example of locating a randomly selected longitudinal sampling location. |
Selecting a Random Core Location
Random core sampling locations need to be identified whenever concrete strength, slab thickness, or entrained air content is estimated based on core samples taken from the hardened concrete slab. These estimated core sample locations are determined within each sublot in a given lot prior to the start of the paving of that lot. Again, these random core locations are typically determined on a day-to-day basis, before the start of that day's paving. Each random core location requires the determination of a longitudinal and horizontal offset within a sublot. Each specific required core location (within a given sublot) may be determined using the following procedures:
This process is demonstrated by the following example:
The longitudinal offset is calculated to be 200 m * 0.345 = 69 m, and the horizontal offset from the outer edge is calculated to be 7.32 m * 0.645 = 4.7 m. The agency may choose to apply restrictions to the randomly generated core locations to avoid sampling near joints or directly in the wheelpaths. This example is illustrated in figure 18.
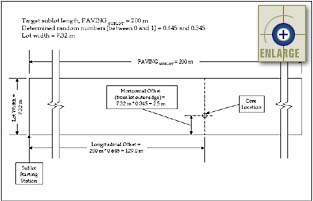 |
Figure 18. Example of locating a randomly selected core location. |
Selecting a Random Dowel Location
The selection of a random dowel location for the testing of percent consolidation around dowels first involves the selection of a random transverse joint, followed by the selection of a random dowel bar within the selected transverse joint. Each required core location (within a given sublot) may be determined using the following procedure:
This process is demonstrated by the following example:
First, the number of actual transverse joints in the sublot
with a length equal to PAVINGSUBLOT is computed to be 43.8
joints by dividing PAVINGSUBLOT by the chosen average transverse
joint spacing (i.e., 200 m/4.57 m = 43.8 joints). This computed value is then
rounded down to 43 and used as the number of joints appearing in the sublot. After
the 43 joints are numbered consecutively in the sublot, the computed number of joints (43
joints) is then multiplied by the first random number (0.246) to determine the selected
transverse joint for investigation. For this example, this specific joint is
determined as 43 joints * 0.246 = 10.6 joints (rounded to 11). Therefore, the
eleventh joint in the sublot is chosen to conduct the percent consolidation sampling.
Next, the specific dowel bar to be investigated must be
determined. After the 23 dowel bars are numbered for the joint (starting at the
outer edge), the second random number (0.302) is multiplied by the number of dowel bars in
one transverse joint (23) to determine the specific dowel bar for investigation.
This dowel bar is determined to be 0.302 * 23 = 6.95, which is then rounded to 7.
Therefore, for this example, dowel bar #7 of transverse joint #11 is investigated for
percent consolidation. This example is illustrated in figure 19.
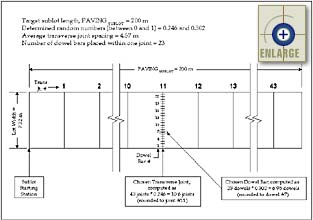 |
Figure 19. Example of locating a randomly selected dowel bar for the investigation of percent consolidation around dowels. |
| Selecting a Maintenance and Rehabilitation Plan | |
The agency must identify an M & R plan for use in the PRS approach. The agency is encouraged to define an M & R plan that closely resembles that actually used in the field. An M & R plan consists of identifying the type and frequency of routine maintenance, localized rehabilitation, and global rehabilitation activities. More information on each of these categories is included below.
The current PRS approach limits routine maintenance activities to the following three:
The agency must decide which of these three activities (if any) are to be included in the yearly M & R cost calculations. For each chosen maintenance activity, the agency must define the frequency of maintenance application (in terms of years). For example, the agency may choose to seal transverse joints every 3 years, whereas transverse crack sealing may be addressed every year. In addition to determining the frequency of maintenance application, the agency must also identify the amount of maintenance to be applied during each visit to the field. The agency answers this question by identifying the percentage of joints or cracks to seal at each scheduled visit to the field.
Local Rehabilitation Activities
Localized rehabilitation activities are defined as those that may be used to correct localized pavement distresses. Localized distresses are defined as those that may affect an individual joint (transverse joint spalling and transverse joint faulting) or slab (transverse slab cracking). The current PRS approach provides the agency with the following choices for localized rehabilitation activities as they relate to the respective distresses:
As with the chosen routine maintenance activities, the agency must define which local rehabilitation activities to apply and when to apply them. This is accomplished by the following three steps:
The agency is encouraged to define a localized rehabilitation plan that closely resembles the rehabilitation activities actually used by the agency in the field.
Global Rehabilitation Activities
Global rehabilitation activities are those activities that are applied to the entire lot (all sublots within the lot) at one time in response to declining global pavement conditions. These activities are specifically applied to address pavement condition indicators such as decreasing pavement smoothness (IRI or PSR), increasing amounts of localized distress, or increasing amounts of applied localized rehabilitation. Trigger values for these pavement condition indicators are typically defined to determine the timing of a global rehabilitation. The current PRS approach limits the global rehabilitation activities to the following three:
As with the chosen localized maintenance activities, the agency must define which global rehabilitation activity to apply and when to apply it. This is accomplished by the following three steps:
Again, the agency is encouraged to define a global rehabilitation plan that closely resembles the rehabilitation activities actually used by the agency in the field.
Cost-Related Decisions |
|
Maintenance and Rehabilitation Unit Costs
The agency must define unit costs for each of the M & R
activities chosen for inclusion in the M & R plan. These selected unit costs,
combined with knowledge of the predicted yearly distresses, allow for the calculation of
the yearly M & R costs. The unit costs for the possible M & R activities are
typically expressed in the following formats.
Maintenance Unit Costs
Localized Rehabilitation Unit Costs
It is recommended that current agency cost records be reviewed in order to obtain the most accurate unit cost values possible for each of the chosen M & R activities.
Under the current PRS approach, the agency has the option to include user costs in the pay factor determination process. User costs are expressed in dollars per kilometer per vehicle and are made up of the following four components:
User costs are calculated for each year based on the predicted pavement smoothness (PSR
or IRI). Details of the user cost calculations are presented in chapter 4, in the
section titled Calculation
of the Representative LCC for a Given Lot.
Under the current approach, the agency may define a certain
percentage of these calculated yearly user costs to be included in the overall lot LCC.
If the agency does not feel that any user costs should influence the pay factor,
this chosen user cost percentage may be set to zero. However, if user costs are
included, it is recommended that the agency include a specific percentage that results in
pay factors with which the agency is comfortable. Typically, user cost percentages
up to 5 percent have resulted in reasonable pay factors. Larger percentages result in pay
adjustments that are quite high.
The agency is required to select a discount rate to be used in determining PW LCC’s. As defined previously, the discount rate is estimated as the difference between the interest and inflation rates, representing the real value of money over time. This relationship is shown below in equation 17.
DISCOUNT = INTEREST – INFLATION (17)
where
DISCOUNT = Estimated discount rate, percent.
INTEREST = Estimated interest rate, percent.
INFLATION = Estimated inflation rate, percent.
The interest rate, often referred to as the market interest rate, is associated with the cost of borrowing money and represents the earning power of money.(10) The inflation rate is typically defined as the rate of increase in the prices of goods and services (construction of highways) and represents changes in the purchasing power of money.(10) In 1997, FHWA recommended that LCC’s should be calculated using "a reasonable discount rate that reflects historical trends over long periods of time."(10) Past research in the U.S. has revealed that the real long-term rate of return on capital (i.e., the discount rate) has generally been between 3 and 5 percent.(10,27) It is recommended that the agency select an appropriate discount rate value from this 3- to 5-percent range.
|
Selecting an Appropriate Bid Price for Developing Level 1 Preconstruction Output |
|
If the agency chooses to compute lot pay factors using Level 1 pay adjustment procedures, an appropriate bid price is required to develop the preconstruction output. This appropriate bid price is required since this preconstruction output must be developed prior to the project being let (prior to obtaining any contractor bids). It is recommended that the agency compute this appropriate bid price as the average of the unit bid prices observed on similar paving projects during the previous year (in dollars per square meter). The agency may use data from other past years; however, those costs should be updated to include the effects of inflation.
Selecting Simulation Paramaters |
|
The generation of preconstruction output involves the simulation of lot LCC’s. The as-designed lot LCC’s for both Level 1 and Level 2, as well as the points making up the Level 1 individual AQC pay factor curves, are determined as the average of "n" simulated lots. Although the simulated LCC mean is generally found to stabilize when n is greater than 200 to 250, it is suggested that the agency use a representative value of n = 500. Additional simulations beyond n = 500 do not change the mean LCC’s significantly.
Simulations for Different Numbers of Sublots Per Lot
Although the sampling and testing plan is easily identified
for each as-constructed sublot, it is not as easy to identify the number of sublots that
will make up each as-constructed lot in the field. The number of actual
as-constructed sublots will most likely vary from lot to lot (day to day) due to changes
in weather, plant and paver equipment problems, and other factors. Because the
simulated preconstruction output (Level 1 individual AQC pay factor curves and the Level 2
as-designed target LCC) is dependent on the number of sublots per lot, this
preconstruction output must then be simulated for the different numbers of projected
sublots per lot. This task is easily accomplished within the PaveSpec 2.0 software
by defining the range of number of sublots per lot for which simulations will be
completed.
After determining the target number of sublots per lot
(NUMSUBS—see the section titled Definition
of a Sublot [in chapter 5]), it is recommended that the agency generate
representative preconstruction output for a range of 1 to NUMSUBS + 3. For example,
if the target number of sublots per lot was estimated as NUMSUBS = 4, it would be
recommended that the agency generate preconstruction output for the cases of 1 to 7
sublots. If an agency chooses to simulate only one particular case for use in
acceptance, it is recommended that the preconstruction output resulting from the
simulation of NUMSUBS per lot be used.