U.S. Department of Transportation
Federal Highway Administration
1200 New Jersey Avenue, SE
Washington, DC 20590
202-366-4000
Federal Highway Administration Research and Technology
Coordinating, Developing, and Delivering Highway Transportation Innovations
 |
| This report is an archived publication and may contain dated technical, contact, and link information |
|
Publication Number: FHWA-RD-01-168 Date: July 2006 |
This chapter provides an evaluation of early observations based on initial performance data and identifies performance differences both within and between the SPS-5 projects, but it is not intended to be a comprehensive analysis of the experiment. Appendix A includes a summary of the amount of distress and performance data that had been collected at each of the 18 SPS-5 sites as of the time of this report.
Six performance indicators were reviewed initially to evaluate potential differences between the test sections (both within and between projects) and to identify performance trends from the early observations. These performance and structural response indicators included fatigue cracking, rutting, longitudinal cracking in and outside the wheel path, transverse cracking, IRI, and deflections measured by sensors 1 and 7.
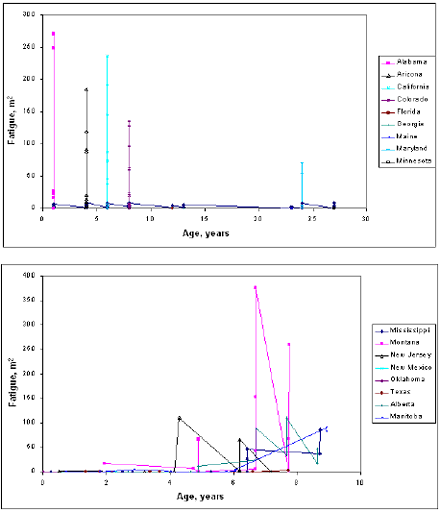
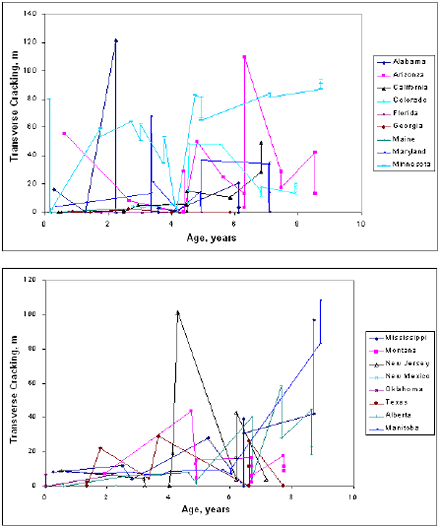
The time-series data were plotted to observe trends for each of the monitoring data elements. The examples in figures 10 through13 compare the performance of the test sections for the different experimental factors for all of the SPS-5 projects (between-project differences). Figure 10 compares the total fatigue cracking for those sections with and without RAP in the HMA overlay mixture; figures 11 and 12 compare the total length of transverse cracks and rut depths for those sections with different surface preparations (defined as minimum and intensive). Figure 13 compares the IRI values for all SPS-5 projects for the existing pavements in different categories (fair and poor). As shown, a wide range of the performance indicators existed within the projects, making it difficult to identify any effect of the key experimental factors on performance. There also was extensive variability between the replicate projects within the same cell, making any graphic comparison difficult to interpret.
Time-series data were also plotted for individual projects to observe and evaluate trends between test sections of the same project and identify possible anomalies in performance data. Examples of the time-series distress data plots are shown in figures 14 through 19 for the Manitoba project. As shown, the data were variable and, more important, many of the distresses abruptly increased and decreased with time. Similar graphic comparisons of the individual test sections within a project were prepared for all other SPS-5 projects.
Examples of these inconsistent time-series data are provided in figures 20 through 24. Figures 20 and 21 show a significant decrease in fatigue cracking for the California and Colorado projects. For the California project (figure 20), the areas of fatigue cracking did not decrease for all test sections, whereas in Colorado (figure 21), the area of fatigue cracking for all test sections significantly decreased, suggesting that some type of maintenance may have been performed.
Figure 10. Graph. Fatigue cracking measured over time for the SPS-5 projects for those sections with HMA overlay mixtures with and without RAP.
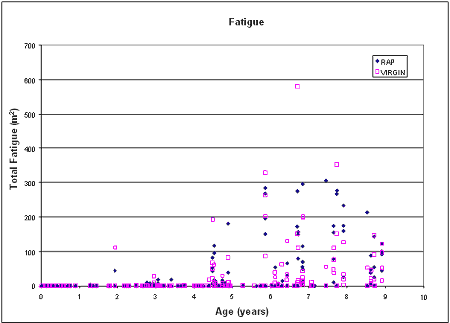
Figure 11. Graph. Total transverse cracking measured along the SPS-5 projects over time or age for those sections with minimum and intensive surface preparation.
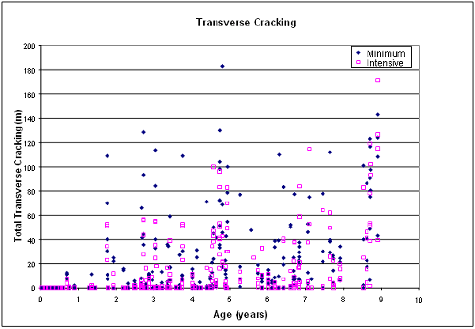
Figure 12. Graph. Rut depths measured over time for the SPS-5 projects for those sections with minimum and intensive surface preparation.
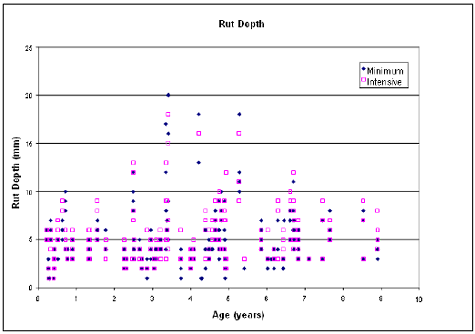
Figure 13. Graph. IRI measured over time for the SPS-5 projects for existing pavements in the fair and poor categories.
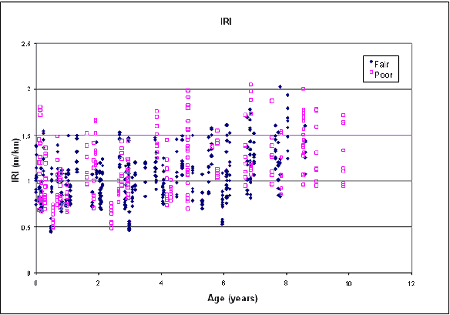
Figure 14. Graphs. Longitudinal cracking outside the wheel path time-series for the Manitoba project.
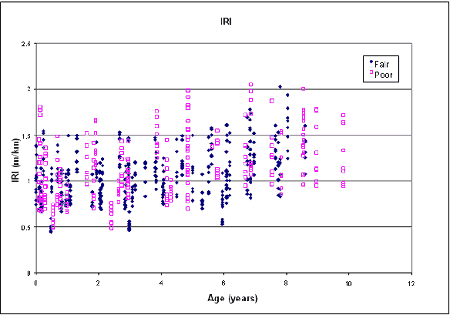
Figure 15. Graphs. Longitudinal cracking within the wheel path time-series data for the Manitoba project.
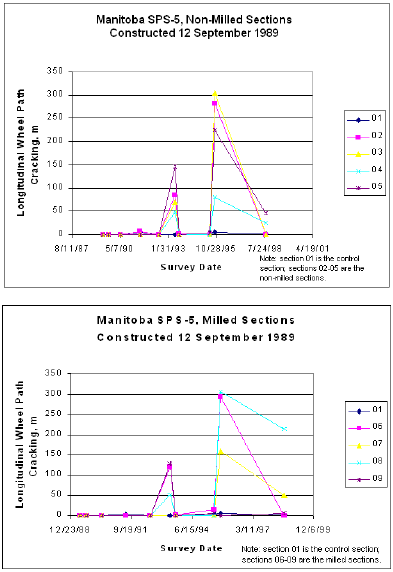
Figure 16. Graphs. Fatigue cracking time-series for the Manitoba project.

Figure 17. Graphs. Transverse crack length time-series data for the Manitoba project.
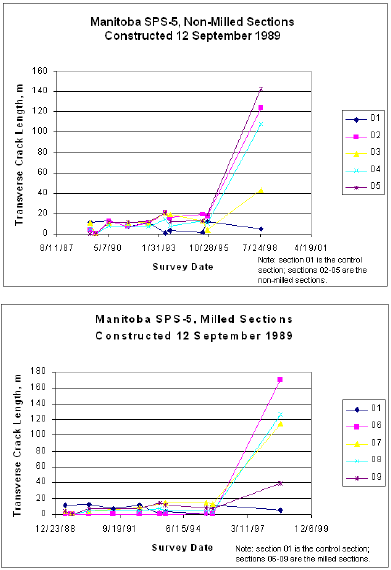
Figure 18. Graphs. Rut depths for the Manitoba project.

Figure 19. Graphs. IRI values for the Manitoba project.
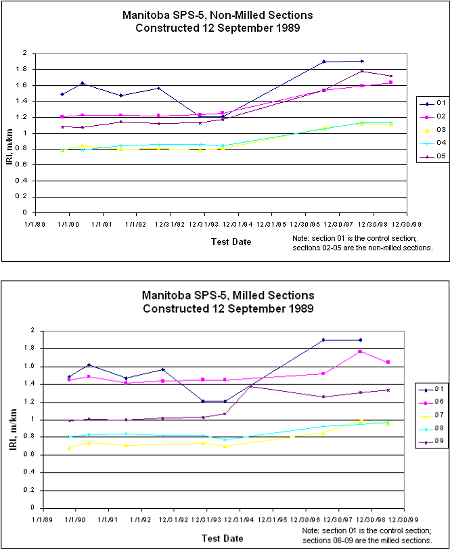
Figure 20. Graphs. Fatigue cracking time-series data for the California project.
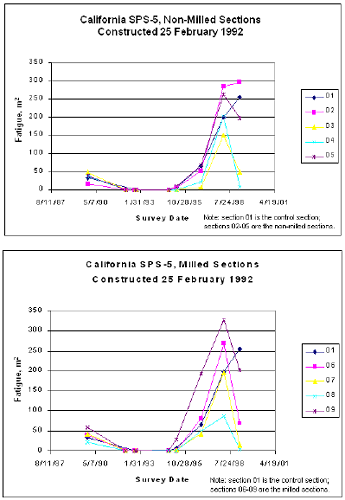
Figure 21. Graphs. Fatigue cracking time-series data for the Colorado project.
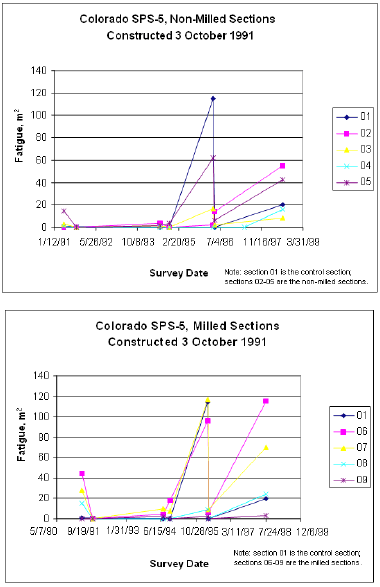
Figure 22. Graphs. Transverse crack length time-series data for the Montana project.
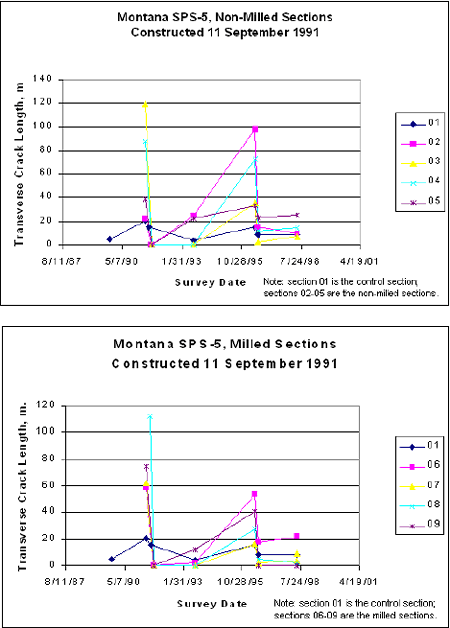
Figure 23. Graphs. Rut-depth time-series data for the Maryland projects.

Figure 24. Graphs. IRI value time-series data for the Maine project.
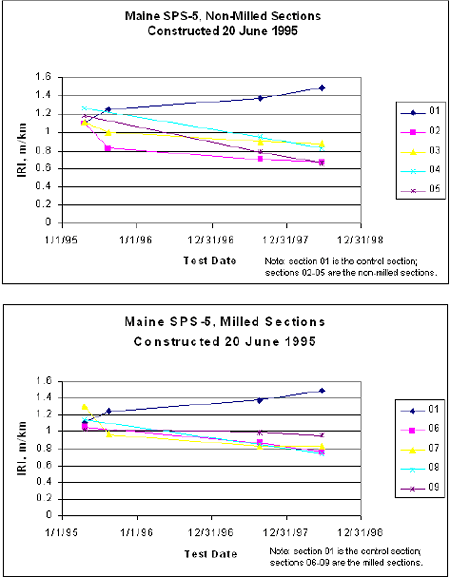
Figure 22 also shows a substantial decrease in the total length of transverse cracks for all test sections in Montana. Figure 23 shows a slight but abrupt increase in the rut depths for all test sections in Maryland, and then a substantial decrease in rut depth for selected test sections of that project. Figure 24 shows a continual decrease in the IRI values for all test sections in Maine except the control section.
This decrease in the magnitudes of the individual distresses (or inconsistent time-series data) is probably related to differences in the distress interpretation between different surveyors and measurement error, or possible maintenance applications that were not recorded in the database. In either case, these inconsistent trends severely complicate graphical comparisons and other analyses based on early distress observations. Thus, four specific distresses were used to evaluate early performance trends from the experiment: fatigue cracking, transverse cracking, rut depths, and IRI.
Table 19 tabulates the percentage of core test sections with distress magnitudes that can be used in comparative studies and in future calibration and validation studies of distress prediction models. About 25 percent of the core test sections exhibited distress magnitudes that exceed the "minimum value" for each of the four distresses. The following discussion provides a brief overview of the four major distress types or performance indicators.
| Performance Indicator | Distress Magnitude Minimal Value |
Core Test Sections Exceeding Minimal Value | |
|---|---|---|---|
| Percentage of Sections | Number of Sections | ||
| Fatigue cracking | >25 m2 | 25.7 | 35 |
| Transverse cracking | > 9 m | 43.4 | 59 |
| Rut depth | >7 mm | 22.8 | 31 |
| IRI | >1.4 m/km | 25.0 | 34 |
Note: The above table excludes all of the control test sections.
Fatigue Cracking
Fatigue cracking occurred on many test sections, but more frequently at older projects. In fact, projects less than 7.3 years old had little to no fatigue cracking, while those in service for more than 7.3 years had extensive fatigue cracking. Table 20 lists the average area of fatigue cracking observed at each project and the age of that project. Figure 25 is a graphic illustration of that data—the average area of fatigue cracking and the total number of test sections with fatigue cracking at a project. The average area of fatigue cracking was consistently less for younger projects. In general, all negative performance indicators increased with age.
| Project | Age, years | Fatigue Cracking of the Control Section, m2 | Average Area of Fatigue Cracking, m2 | Number of Sections with Fatigue Cracking |
|---|---|---|---|---|
| Missouri | 0.0 | 0.0 | 0.0 | 0 |
| Oklahoma | 2.1 | 0.0 | 0.0 | 0 |
| New Mexico | 2.9 | 0.0 | 0.0 | 0 |
| Maine | 4.1 | 0.0 | 0.0 | 0 |
| Florida | 4.3 | 0.0 | 0.0 | 0 |
| Georgia | 6.2 | 0.0 | 0.0 | 0 |
| New Jersey | 7.0 | 193.7 | 1.7 | 3 |
| Maryland | 7.2 | 70.0 | 1.5 | 2 |
| California | 7.3 | 254.7 | 104.6 | 8 |
| Alabama | 7.7 | 248.6 | 16.1 | 4 |
| Texas | 7.8 | 5.1 | 0.0 | 0 |
| Colorado | 7.9 | 19.9 | 97.1 | 8 |
| Montana | 8.0 | 0.0 | 131.0 | 6 |
| Minnesota | 8.9 | 0.0 | 0.0 | 0 |
| Mississippi | 8.9 | 29.2 | 60.1 | 7 |
| Alberta | 8.9 | 1.8 | 33.8 | 8 |
| Arizona | 9.2 | 66.6 | 45.0 | 2 |
| Manitoba | 10.0 | 129.0 | 80.8 | 8 |
Figure 26 shows the average area of fatigue cracking for each project over time. The area of fatigue cracking increased with age (or traffic), as expected. The California, Colorado, and Montana projects had the greatest areas of fatigue cracking, while the Texas and Minnesota projects had no fatigue cracking at about the same age. To explain the differences between these projects requires that the traffic and materials data be available.
Transverse Cracking
Transverse cracking occurred on all but four of the SPS-5 projects—Florida, Georgia, Maine,and Missouri—all of which were less than 7 years old. Most projects that were older than 7 years exhibited at least moderate levels of transverse cracking, even including those in a no-freeze climate. For example, the Arizona, Mississippi, and Texas projects had extensive lengths of transverse cracking.
Table 21 lists the average length of transverse cracks for each project. Figure 27 is a graphic illustration of that data—the average length of transverse cracks and the total number of test sections with transverse cracks at a project. The length of transverse cracks increased with age.
Figure 28 shows the average length of transverse cracks with time for each project (time-series data). The average length of transverse cracks significantly increased and decreased with time for some projects. This extensive variability complicates any interpretation of the graphic comparisons. Materials data for each mixture are needed to determine the reasons for the extensive cracking in some warmer climates.
Figure 25. Graphs. Fatigue cracking observed on each project as of January 2000.
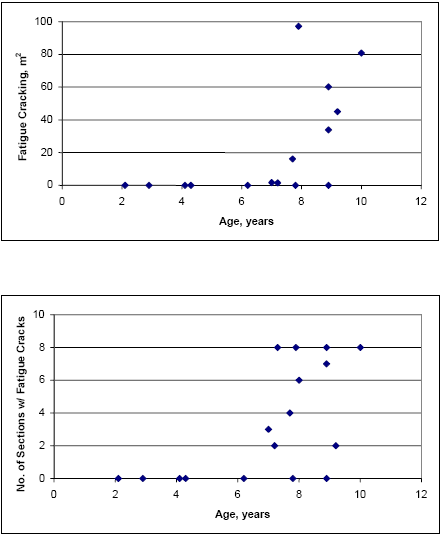
Figure 26. Graphs. Average area of fatigue cracking for each project over time.
| Project | Age, years | Average Length of Transverse Cracks, m | Number of Sections with Transverse Cracks |
|---|---|---|---|
| Missouri | 0.0 | 0.0 | 0 |
| Oklahoma | 2.1 | 3.9 | 0 |
| New Mexico | 2.9 | 0.5 | 1 |
| Maine | 4.1 | 0.0 | 0 |
| Florida | 4.3 | 0.0 | 0 |
| Georgia | 6.2 | 0.0 | 0 |
| New Jersey | 7.0 | 11.4 | 6 |
| Maryland | 7.2 | 19.8 | 7 |
| California | 7.3 | 34.0 | 8 |
| Alabama | 7.7 | 1.3 | 2 |
| Texas | 7.8 | 15.1 | 6 |
| Colorado | 7.9 | 17.1 | 8 |
| Montana | 8.0 | 12.3 | 7 |
| Minnesota | 8.9 | 92.0 | 8 |
| Mississippi | 8.9 | 55.1 | 8 |
| Alberta | 8.9 | 34.3 | 8 |
| Arizona | 9.2 | 24.4 | 5 |
| Manitoba | 10.0 | 108.7 | 8 |
Rut Depths
Rut depths exceeding 7 mm were measured on eight projects: Arizona, Alberta, Manitoba, Maryland, Mississippi, Montana, Oklahoma, and Texas. However, on half of these projects only one or two of the test sections had rut depths exceeding 7 mm. The four projects where most of the test sections exceeded rut depths of 7 mm were Maryland, Mississippi, Montana, and Oklahoma. In all probability, rut depths measured along these four projects were more related to the HMA mixture characteristics and properties than to any key factor included in the experiment. Table 22 lists the average rutting measured on each project. All projects were in extremely different climates.
Smoothness—IRI Values
Table 22 lists the average IRI values for each project and the percentage of test sections within each project that exceeded an IRI value of 1.2 m/km. Most projects with IRI values exceeding 1.2 m/km were older than 7 years, with the exception of the Alabama project. These were the same test sections that had extensive transverse and fatigue cracking. Transverse and fatigue cracking probably caused the increased roughness (increased IRI values) at these sites. In fact, the authors found in previous studies that the IRI is related to the standard deviation of the rut depth, transverse cracking, fatigue cracking, and other distresses.(10) Thus, there are interactions among the performance measures that should be considered in future studies using data from this experiment.
Figure 27. Graphs. Length of transverse cracks observed on each project as a function of time.

Figure 28. Graphs. Average length of transverse cracking for each project over time.
| Project | Age, years | Rut Depths, mm | IRI, m/km | ||||
|---|---|---|---|---|---|---|---|
| Control Section | Average Rutting on Project | Percentage of Test Sections Exceeding 7 mm | Control Section | Average IRI on Project | Percentage of Test Sections Exceeding 1.2 m/km | ||
| Missouri | 0.0 | – | – | 0 | – | – | 0 |
| Oklahoma | 2.1 | 10 | 7 | 25 | 1.32 | 1.00 | 0 |
| New Mexico | 2.9 | 3 | 3 | 0 | 0.45 | 0.49 | 0 |
| Maine | 4.1 | 14 | 5 | 0 | 1.49 | 0.79 | 0 |
| Florida | 4.3 | – | 3 | 0 | – | 0.62 | 0 |
| Georgia | 6.2 | 11 | 3 | 0 | – | 0.57 | 0 |
| New Jersey | 7.0 | 9 | 3 | 13 | 1.54 | 1.14 | 25 |
| Maryland | 7.2 | 9 | 3 | 13 | 1.54 | 1.14 | 25 |
| California | 7.3 | 6 | 5 | 0 | 1.73 | 1.45 | 38 |
| Alabama | 7.7 | – | 3 | 0 | 0.79 | 0.89 | 0 |
| Texas | 7.8 | – | 5 | 0 | 2.38 | 1.39 | 63 |
| Colorado | 7.9 | 11 | 4 | 0 | 1.20 | 1.11 | 13 |
| Montana | 8.0 | – | 8 | 50 | 1.02 | 1.16 | 25 |
| Minnesota | 8.9 | 8 | 3 | 0 | 3.47 | 1.59 | 88 |
| Mississippi | 8.9 | 14 | 12 | 100 | 1.67 | 1.62 | 88 |
| Alberta | 8.9 | 8 | 5 | 0 | 1.94 | 1.35 | 50 |
| Arizona | 9.2 | 8 | 4 | 0 | 1.34 | 1.33 | 50 |
| Manitoba | 10.0 | 13 | 4 | 0 | 1.90 | 1.31 | 50 |
An analysis of variance (ANOVA) was completed for each of the four major distress types to determine if the main factors of the experiment had an effect on those distresses from these early observations. The major factors included in the ANOVA are listed below:
Results from this one-way ANOVA are summarized in table 23, which indicates that surface preparation and climatic conditions had an effect on the pavement distress (the p-values are low, indicating a low probability of a chance event). However, the overriding factor that had a significant effect on all of the distresses was age. In fact, the age of the overlay was so important that it probably reduced the effect of some of the other key experimental factors. Age represents both aging effects on materials (i.e., stiffness increases) and of temperature and moisture. Age also was correlated with traffic loadings over time, although different SPS-5 experiments had different traffic levels.
| Experimental Factor | Performance Indicator/Surface Distress | |||
|---|---|---|---|---|
| Fatigue Cracking | Transverse Cracking | Rut Depths | IRI | |
| Nominal HMA overlay thickness | 0.514 | 0.847 | 0.942 | 0.865 |
| HMA overlay mixture | 0.304 | 0.529 | 0.354 | 0.110 |
| Existing pavement condition | 0.600 | 0.126 | 0.133 | 0.0003 |
| Nominal milling depth | 0/762 | 0.0007 | 0.832 | 0.0060 |
| Precipitation | <0.0001 | 0.185 | <0.0001 | 0.687 |
| Freeze index | 0.0005 | <0.0001 | 0.128 | 0.0607 |
| Age of overlay | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
The following summarizes the effect of the key factors of the experiment on individual distresses using data extracted from the IMS in January 2000. A description of the effects and possible reasons for those effects are discussed in the next section of this chapter.
Fatigue Cracking—Age of the overlay and the climatic factors, temperature and moisture, were important and had an effect on the fatigue cracking at each project. The thickness of the overlay was much less important than these two factors, based on these early observations. More fatigue cracking occurred on those test sections placed in a climate with less precipitation but higher freeze indices.
Transverse Cracking—Age of the overlay, milling depth, and freeze index were found to have an important effect on the length of transverse cracks along each test section. Longer transverse cracks occurred on the older pavements in areas with higher freeze indices. In addition, fewer or shorter transverse cracks occurred on sections that had been milled.
Rut Depths—Age of the overlay and precipitation were the two factors found to have an important effect on rut depths. The rut depth increased as the age of the overlay increased, as expected. Sections with increased precipitation had larger rut depths. However, increased precipitation may not have been the primary factor related to increased rut depths. The HMA mixture properties were probably more important, but they were unavailable for the ANOVA. The precipitation may have been a biased effect, simply because those projects with the higher rut depths were located in climates with higher precipitation. This topic needs further study using the materials testing data.
IRI—The age of the overlay, condition of the pavement before overlay placement, and surface preparation or milling depth were factors found to be important relative to the IRI values. The IRI values of the overlay were found to be lower for the overlays placed over pavements in the fair category and when the existing surface was milled before overlay.
The remaining sections of this chapter discuss the effect of each key factor of the experiment in relation to the magnitude and relative occurrence of observed distresses. Tables 24 through 27 summarize the differences on the average performance measures between the key factors of the experiment.
| Distress or Performance Indicator | Surface Preparation—Milled or Non-milled Surfaces | |||
|---|---|---|---|---|
| Control | Minimal (Non-Milled) | Intensive (Milled) | ||
| Fatigue cracking | Mean, m2 | 37.5 | 17.1 | 16.1 |
| Std. deviation, m2 | 73.4 | 51.4 | 55.4 | |
| COV*, % | 196 | 301 | 344 | |
| Transverse cracking | Mean, m | 36.8 | 18.5 | 12.7 |
| Std. deviation, m | 50.8 | 30.8 | 24.8 | |
| COV, % | 37 | 166 | 195 | |
| Rut depth | Mean, mm | 10 | 5 | 5 |
| Std. deviation, mm | 3.7 | 3.0 | 2.9 | |
| COV, % | 38 | 62 | 56 | |
| IRI | Mean,k/km | 1.48 | 1.10 | 1.05 |
| Std. deviation, m/km | 0.53 | 0.32 | 0.29 | |
| COV, % | 36 | 29 | 28 | |
* COV = coefficient of variance
| Distress or Performance Indicator | Existing Pavement Condition | |||
|---|---|---|---|---|
| Control | Poor | Fair | ||
| Fatigue cracking | Mean, m2 | 37.5 | 18.7 | 15.5 |
| Std. deviation, m2 | 73.4 | 54.3 | 53.7 | |
| COV*, % | 196 | 290 | 347 | |
| Transverse cracking | Mean, m | 36.8 | 12.7 | 17.8 |
| Std. deviation, m | 50.8 | 28.4 | 28.1 | |
| COV, % | 37 | 223 | 158 | |
| Rut depth | Mean, mm | 10 | 5 | 5 |
| Std. deviation, mm | 3.7 | 2.7 | 3.2 | |
| COV, % | 38 | 56 | 60 | |
| IRI | Mean,k/km | 1.48 | 1.13 | 1.04 |
| Std. deviation, m/km | 0.53 | 0.341 | 0.283 | |
| COV, % | 36 | 30 | 27 | |
| Distress or Performance Indicator | Overlay Thickness | |||
|---|---|---|---|---|
| Control | Thin (51 mm) | Thick (127 mm) | ||
| Fatigue cracking | Mean, m2 | 37.5 | 15.3 | 17.9 |
| Std. deviation, m2 | 73.4 | 48.2 | 58.2 | |
| COV*, % | 196 | 314 | 326 | |
| Transverse cracking | Mean, m | 36.8 | 16.0 | 15.3 |
| Std. deviation, m | 50.8 | 29.3 | 26.9 | |
| COV, % | 37 | 183 | 176 | |
| Rut depth | Mean, mm | 10 | 5 | 5 |
| Std. deviation, mm | 3.7 | 2.9 | 3.0 | |
| COV, % | 38 | 59 | 58 | |
| IRI | Mean,k/km | 1.48 | 1.07 | 1.08 |
| Std. deviation, m/km | 0.53 | 0.314 | 0.304 | |
| COV, % | 36 | 29 | 28 | |
| Distress or Performance Indicator | Overlay Mixture | |||
|---|---|---|---|---|
| Control | With RAP | Without RAP,Virgin Mix | ||
| Fatigue cracking | Mean, m2 | 37.5 | 18.6 | 17.9 |
| Std. deviation, m2 | 73.4 | 54.4 | 52.3 | |
| COV*, % | 196 | 292 | 360 | |
| Transverse cracking | Mean, m | 36.8 | 16.2 | 15.0 |
| Std. deviation, m | 50.8 | 29.2 | 27.0 | |
| COV, % | 37 | 180 | 179 | |
| Rut depth | Mean, mm | 10 | 5 | 5 |
| Std. deviation, mm | 3.7 | 3.0 | 2.9 | |
| COV, % | 38 | 58 | 59 | |
| IRI | Mean,k/km | 1.48 | 1.09 | 1.06 |
| Std. deviation, m/km | 0.53 | 0.314 | 0.304 | |
| COV, % | 36 | 29 | 29 | |
Surface Preparation
The amount of transverse cracking of sections with intensive surface preparation before overlay was much lower than for sections with minimal surface preparation (table 24). Figure 29 illustrates that the percentage of test sections with more than 9 m of transverse cracking was much larger for those sections with minimal surface preparation, regardless of the freeze environment.
The IRI was slightly larger for sections with minimal surface preparation. The difference in the values in table 24 is small. However, figure 30 illustrates that the percentage of test sections with an IRI value greater than 1.2 m/km was much larger for sections with minimal surface preparation.
Figure 29. Graph. Percentage of test sections that have more than 9 m of transverse cracking.
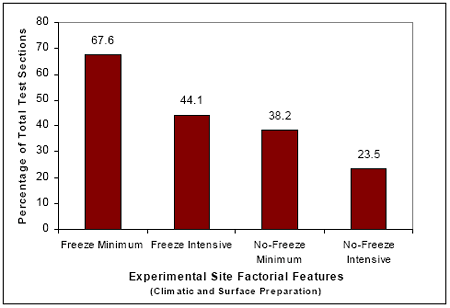
Figure 30. Graph. Percentage of test sections that have an IRI value greater than 1.2 m/km.

Neither the amount of fatigue cracking nor the amount of rutting observed on the sections after overlay appeared to be affected by the surface preparation before overlay.
Pavement Condition
The amount of transverse cracking observed on sections that were in fair condition before overlay was higher than in sections that were in poor condition before overlay (table 25). A bias may have influenced this result: in some cases, the surveyors classified the cracking as reflective cracking while other surveyors classified it as transverse cracking.
Sections in fair condition before overlay were rougher than those in poor condition before overlay. Figure 30 illustrates that this conclusion was not consistent among the different surface preparation conditions. The percentage of sections with more than 1.2 m/km of IRI was larger for sections in poor condition with minimal surface preparation than any of the other categories. However, the sections that were in poor condition with intensive surface preparation had the least number of sections with an IRI greater than 1.2 m/km. This observation indicates that the early pavement condition can be overcome by the amount of surface preparation before overlay.
HMAC Overlay Thickness and Material
Very little difference was observed between the distresses for either the overlay thicknesses or the virgin/RAP mixtures (tables 26 and 27, respectively). While little difference was observed, the oldest of these projects was 10 years old. Hence, it is possible that the amount of distress was not affected by these factors in the short term, but these factors might be very important to the amount of distress in the long term. Only long-term monitoring will answer this question and many similar questions for other design features.
It should be noted that some of these observations were not new findings (for example, condition of the pavement before overlay affects the roughness of the overlay), but they demonstrate that results from the SPS-5 experiment are consistent with previous experience. Early observations from the SPS-5 experiment clearly demonstrate its potential value and that the experimental objectives can be met over the long term. Clearly, findings from the SPS-5 experiment will affect highway agency designs and standards.
The construction and deviation reports were also found to be extremely valuable and important to explain possible anomalies in the experiment and performance differences from the other projects and test sections. Use of these reports should limit or reduce the possibility of having biased conclusions from the data related solely to construction. However, to extract the full benefit of SPS-5, the materials testing program planned for this experiment must be completed and the truck traffic data must be collected on projects that had no data at the time of this report.