U.S. Department of Transportation
Federal Highway Administration
1200 New Jersey Avenue, SE
Washington, DC 20590
202-366-4000
Federal Highway Administration Research and Technology
Coordinating, Developing, and Delivering Highway Transportation Innovations
| REPORT |
| This report is an archived publication and may contain dated technical, contact, and link information |
|
| Publication Number: FHWA-HRT-16-058 Date: December 2016 |
Publication Number: FHWA-HRT-16-058 Date: December 2016 |
Table 3 shows the number of crashes, the number of crashes avoided by each group, the maximum likelihood estimates of crash probability, and the 95-percent confidence limit (CL) for those estimates. The probability of a crash was reduced for the full CACC system (both braking and alarm enabled) compared to the other groups. This effect was tested using a generalized linear model (GLM) with a binomial distribution and logit link function. The effect of condition was significant ( χ2(3) = 10.6, p = 0.01). Post hoc testing showed that only the CACC-AB significantly differed from the ACC control (p = 0.003).
| Condition | No. of Avoided Crash | No. of Crashed | Crash Probability | Lower 95-Percent CL |
Upper 95-Percent CL |
|---|---|---|---|---|---|
| ACC | 13 | 15 | 0.54 | 0.35 | 0.71 |
| CACC-AB | 24 | 4 | 0.14 | 0.05 | 0.32 |
| CACC-A | 13 | 15 | 0.54 | 0.35 | 0.71 |
| CACC-B | 14 | 14 | 0.50 | 0.32 | 0.68 |
| Total | 64 | 48 | 0.43 | nc | nc |
| nc = Not computed. | |||||
The reaction times to the onset of the crash event are shown in figure 2, which displays the mean reaction times and 95-percent CLs about the means for the four conditions. Three participants in the CACC-B group never reacted and therefore were not included in the reaction time analysis. A GLM with normal response distribution and identity link function showed the condition effect significant (χ2(3) = 59.2, p < 0.0001). Post hoc testing showed that the ACC group mean reaction time did not differ significantly from the CACC-AB group mean but that all the other group mean comparisons yielded significant differences.
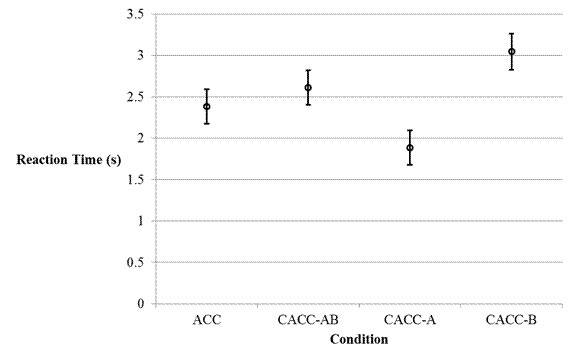
Figure 2. Graph. Reaction time from onset of braking by platoon-lead vehicle.
The TTC findings are displayed in figure 3, which shows the adjusted TTC means and 95-percent CLs about the means for the fourconditions. Figure 3 is based on a sample size of 92 participants. The remaining 20participants had uninterpretable adjusted TTC estimates; 3 of those 20 are the same participants who had no reaction time and never applied the brakes. The remaining 17participants had uninterpretable adjusted TTC values because they were decelerating at a rate less than that of the lead vehicle (also decelerating) at the time of impact, thereby generating adjusted minimum TTC values of negative infinity. Table4 shows that the ACC and CACC-A groups had the highest frequency of such values. Although the frequency of negative infinity occurrence is too low to enable meaningful statistical tests for group differences, the trend seems to suggest that automated braking contributed to mitigating the probability of inadequate braking responses.
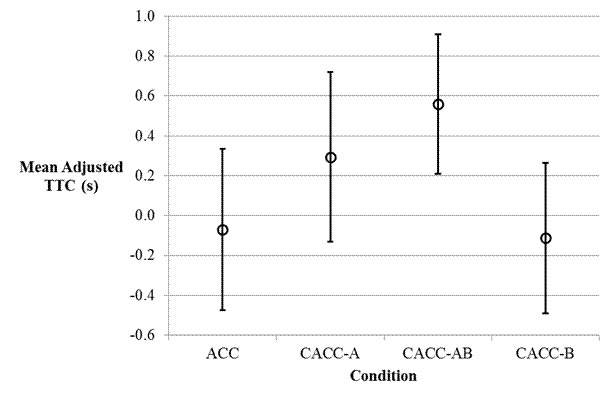
Figure 3. Graph. TTC results.
Table 4. Frequency of drivers for whom precise values of adjusted TTC could not be calculated.
| Group | Number of Subjects with Minimum TTC Values of Negative Infinity |
|---|---|
| ACC | 7 |
| CACC-AB | 0 |
| CACC-A | 9 |
| CACC-B | 1 |
GLM models with normal response distribution and identity link function showed the effect of condition significant (χ2(3) = 8.54, p = 0.04). As can be seen in figure 3, the CACC-AB group had a substantial positive adjusted TTC (i.e., on average, members of this group have almost 0.6 s extra to respond to the collision event). The ACC and CACC-B groups had significantly lower mean adjusted TTC values than the CACC-AB group. The CACC-A group mean was not significantly different from any of the other three group means.