U.S. Department of Transportation
Federal Highway Administration
1200 New Jersey Avenue, SE
Washington, DC 20590
202-366-4000
Federal Highway Administration Research and Technology
Coordinating, Developing, and Delivering Highway Transportation Innovations
| REPORT |
| This report is an archived publication and may contain dated technical, contact, and link information |
|
| Publication Number: FHWA-HRT-16-040 Date: July 2016 |
Publication Number: FHWA-HRT-16-040 Date: July 2016 |
This chapter describes the methodology and results from an open-road study that examined different flash patterns for use with yellow RRFBs.
When IA-11 was issued in July 2008 for the RRFBs, the only flash pattern that had been tested was the 2-5 flash pattern.(4) Because the 2-5 flash pattern appears be a 2-3 flash pattern according to the human eye, several devices were installed with the 2-3 flash pattern rather than the 2-5 flash pattern. Only after looking at the flash pattern using an oscilloscope were transportation professionals able to determine that the original devices had a 2-5 flash pattern, which is why FHWA changed the flash pattern from a 2-3 flash pattern to a 2-5 flash pattern in Official Interpretation 4(09)-21.(9)
An inability to accurately determine the number of pulses within the 2-5 RRFB flash pattern was later confirmed in the closed-course study (see chapter 3). The same study found that certain flash patterns (i.e., those that could be characterized as having limited or no dark periods within the flash pattern) negatively influenced the amount of time participants needed to identify a pedestrian's direction of travel. Prior to developing the proposed provisions for incorporating the RRFB a rapid-flashing beacon traffic control device into the MUTCD, it is important to determine which flash patterns are acceptable from the perspectives of effectiveness and simplicity.(1) This study sought to determine if less complicated flash patterns and flash pattern with different proportions of dark and light periods could be as or more effective than the 2-5 flash pattern.
The objective of this study was to determine if the use of simpler flash patterns or flash patterns with a greater proportion of dark periods resulted in different driver yielding rates at uncontrolled crosswalks in an open-road setting. This study's measure of effectiveness (MOE) was the number of drivers who did and did not yield at crosswalks during staged pedestrian crossings.
The cities of College Station, TX, and Garland, TX, along with TAMU agreed to participate in the study by providing locations where the research team could install temporary equipment. Table 54 lists the sites included in the study. A goal was to try to match the distribution of site characteristics used in the original FHWA study on RRFBs.(16) For example, the research team preferred locations on multilane roads so that yielding behavior associated with the multiple threats issue could be observed. Because of limited ability to mount temporary beacons on overhead mast arms, the research team did not consider locations where the RRFB had been installed on mast arms over the roadway.
| Site ID | Posted Speed Limit (mi/h) | Number of Lanes | Median | Crossing Distance (ft) |
|---|---|---|---|---|
| CS-02 | 40 | 4 | Flush | 56 |
| CS-03 | 30 | 2 | Flush | 37 |
| GA-02 | 40 | 4 | Flush | 58 |
| GA-06 | 40 | 4 | Raised | 80 |
| GA-07 | 45 | 4 | Raised | 82 |
| GA-10 | 40 | 4 | Raised | 62 |
| GA-11 | 40 | 4 | Raised | 62 |
| GA-13 | 40 | 4 | Raised | 55 |
To conduct an in-field evaluation of multiple flash patterns, the research team needed to be able to set the flash pattern and brightness of the beacons at the study sites in a quick, reliable, and consistent manner. Because of the difficulties with working with different equipment in different cities and unknown characteristics for the beacons at these locations (such as brightness), the research team designed temporary controllers to be used with temporary light bars. In the field, the temporary light bars were mounted in front of existing RRFB light bars.
The temporary light bar setup was designed such that it was not obvious that the beacons being observed during the staged pedestrian crossings were any different from the permanent RRFB equipment. Figure 50 shows an example of TTI personnel installing the temporary light bar at a site, and figure 51 shows an example of the installed light bar being used by a staged pedestrian. The staged pedestrian had a remote control to activate the light bars and activated the device if a non-staged pedestrian approached the crossing while the temporary light bars were installed.

Figure 50. Photo. Installation of the light bar in field.

Figure 51. Photo. CS-02 study site with installed temporary light bars and staged pedestrian crossing.
The study budget and parameters made it possible to test four different conditions at each study site. One of the four conditions was reserved for collecting driver yielding data with the existing equipment. Data were collected with the existing equipment in order to control for differences between the existing equipment and the temporary equipment. The other three conditions used the temporary equipment. Of the three remaining conditions, one condition was reserved for the 2-5 flash pattern.
To determine flash patterns for the other two conditions, a flash pattern workshop was held at TTI. The workshop included a selection of licensed transportation engineering professionals, representatives of FHWA, and TTI research staff. The patterns were initially reviewed using a mockup of a rectangular beacon light bar and a controller in a conference room. Several pre-developed patterns were shown to the participants. Based on participant comments, new patterns were developed. For example, some flash patterns were changed to have more dark periods or to have periods where both beacons were on. A reason for wanting increased dark periods for some of the flash patterns for this study was a preliminary finding from a closed-course research study (see chapter 3) that indicated drivers could determine the direction a pedestrian was walking in a crosswalk more quickly when the flashing traffic control devices had larger dark periods.
After identifying a short list of potential patterns during the meeting in the conference room, the meeting moved to a TTI closed-course location to look at the potential patterns in the field during the nighttime setting. The participants parked the vehicle 200 ft from a crosswalk on a two-lane approach with RRFB assemblies located on both sides of the roadway. The patterns developed during the conference room meeting were demonstrated to the meeting participants in the field. Based on the meeting participants' comments, two potential patterns were selected. These two patterns were demonstrated to FHWA representatives, and final approval was given to use these two flash patterns as the two remaining conditions for the open-road study.
Figure 52 illustrates the three patterns selected for testing in the field using the temporary light bars. The patterns considered in this study included the following:
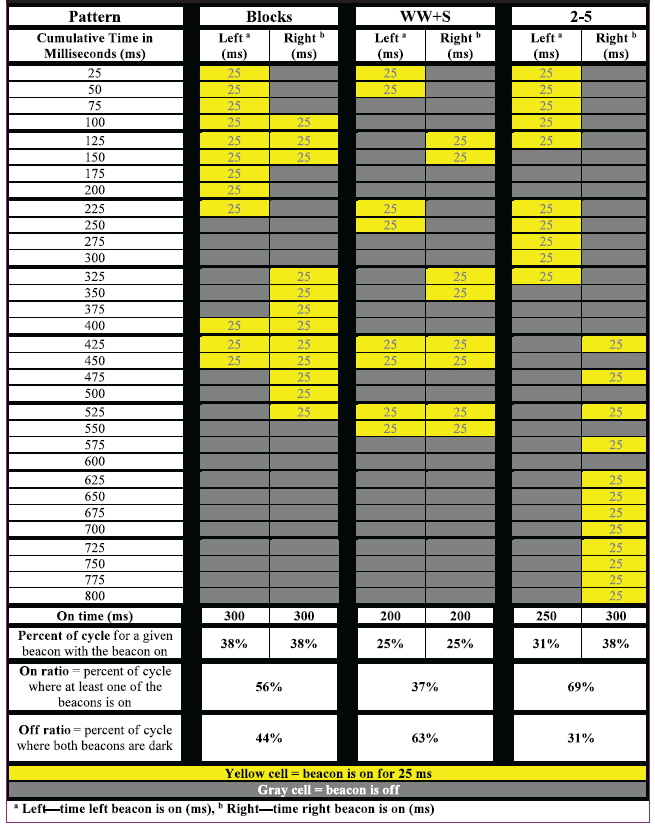
Figure 52. Illustration. Flash patterns studied.(49)
Preliminary findings from the closed-course study (see chapter 3) indicate that brightness of the beacons can influence how quickly a participant can detect a pedestrian within a crosswalk. Therefore, the same brightness level was used for the three flash patterns tested with the temporary light bars. Table 55 shows the target and measured intensity for the beacons when measured at horizontal and vertical angles of 0 degrees. The table also shows the measured optical power along with the on and off ratios (i.e., percent of the cycle where at least one of the beacons was on or where both beacons were dark, respectively).
| Flash Pattern with Temporary Equipment | Target Intensity (Candela) | Measured Target Intensity (Candela) | Optical Power (Candela-s/min) | On Ratio (Percent) | Off Ratio (Percent) |
|---|---|---|---|---|---|
| 2-5 | 1,400 | 1,414 | 58,300 | 69 | 31 |
| Blocks | 1,400 | 1,415 | 63,700 | 56 | 44 |
| Wig-wag and simultaneous (WW+S) | 1,400 | 1,418 | 42,500 | 37 | 63 |
Based on a statistical analysis of past driver yielding data at RRFB locations in Texas, the research team estimated it would take between 7 and 13 sites to obtain a sufficient sample of data to permit detection of at least a 5 percent difference in driver yielding.(36) With available resources for the study, a total of eight sites were selected for testing. Based on previous experience, the minimum number of staged pedestrian crossings for each condition was set at 40.
The order that treatments were presented could have had an effect on results; therefore, flash pattern order for the sites was randomized. Table 56 lists the order that the flash patterns were installed at each site.
| Site ID | Initial Flash Pattern | Second Flash Pattern | Third Flash Pattern | Fourth Flash Pattern |
|---|---|---|---|---|
| GA-02 | Temporary; 2-5 | Existing; 2-5 or 2-3 | Temporary; blocks | Temporary; WW+S |
| CS-02 | Existing; 2-5 or 2-3 | Temporary; blocks | Temporary; WW+S | Temporary; 2-5 |
| CS-03 | Temporary; blocks | Temporary; WW+S | Temporary; 2-5 | Existing; 2-5 or 2-3 |
| GA-06 | Temporary; WW+S | Temporary; 2-5 | Existing; 2-5 or 2-3 | Temporary; blocks |
| GA-07 | Temporary; 2-5 flash | Existing; 2-5 or 2-3 | Temporary; blocks | Temporary; WW+S |
| GA-10 | Existing; 2-5 or 2-3 | Temporary; blocks | Temporary; WW+S | Temporary; 2-5 |
| GA-11 | Temporary; blocks | Temporary; WW+S | Temporary; 2-5 | Existing; 2-5 or 2-3 |
| GA-13 | Temporary; WW+S | Temporary; 2-5 | Existing; 2-5 or 2-3 | Temporary; blocks |
Note: Flash patterns are defined as follows: 2-5 = 2-5 flash pattern and 2-3 = 2-3 flash pattern.
The data were collected during daytime conditions in February and March 2014. The research team avoided Monday mornings and Friday afternoons along with weekends because travel patterns for those time periods can be different from travel patterns associated with a typical weekday.
The research team used a staged pedestrian protocol to collect driver yielding data to ensure that oncoming drivers received a consistent presentation of approaching pedestrians. Under this protocol, a member of the research team acted as a pedestrian using the crosswalk to stage the conditions under which driver yielding would be observed. Each staged pedestrian wore similar clothing (gray t-shirt, blue jeans, and gray tennis shoes) and followed specific instructions in crossing the roadway. The staged pedestrian was accompanied by a second researcher, who observed and recorded the yielding data on pre-printed datasheets. Additional information on the staged pedestrian protocol followed is available in chapter 4 of this report or in "Driver Yielding to Traffic Control Signals, Pedestrian Hybrid Beacons, and Rectangular Rapid-Flashing Beacons in Texas."(36)
After completing the data collection, researchers entered the crossing data and the site characteristics data from the field worksheets into an electronic database. The average yielding rate for a site was calculated; however, data for individual crossings were used in the statistical evaluation. Table 57 lists the driver yielding rates for each site, type of light bar, and flash pattern. As shown in the final row of the table, the three flash patterns used with the temporary light bar had similar average driver yielding rates—between 78 and 80 percent. When comparing the results for the individual sites, some sites did have larger differences between the different flash patterns.
| Site | Temporary Light Bars with WW+S (Percent) | Temporary Light Bars with Blocks (Percent) | Temporary Light Bars with 2-5 Flash Pattern (Percent) |
Existing Light Bars with 2-5 or 2-3 Flash Patterns (Percent) |
|---|---|---|---|---|
| CS-02 | 63 | 50 | 61 | 44 |
| CS-03 | 84 | 94 | 87 | 76 |
| GA-02 | 76 | 75 | 67 | 98 |
| GA-06 | 96 | 81 | 85 | 96 |
| GA-07 | 78 | 92 | 84 | 92 |
| GA-10 | 90 | 94 | 89 | 94 |
| GA-11 | 87 | 90 | 82 | 92 |
| GA-13 | 80 | 84 | 84 | 95 |
| Total | 80 | 80 | 78 | 81 |
When a driver approaches a crossing, the driver either yields and stops the vehicle or does not yield to the waiting staged pedestrian. This binary behavior (yield or no yield) can be modeled using logistic regression. A significant advantage of using logistic regression is it permits consideration of individual crossing data rather than reducing all the data at a site to only one value. For the dataset available within this study, that means over 1,100 data points could be available (i.e., all the unique staged crossings recorded) rather than only 32 data points (i.e., the number of study sites by number of flash patterns). The larger sample size provides more detailed data and could result in finding significant relationships that would not be apparent with a smaller dataset.
Using logistic regression to model the relationships assumes that the logit transformation of the outcome variable (i.e., yielding rate) has a linear relationship with the predictor variables, which results in challenges in interpreting the regression coefficients. Odds ratios can be used to illustrate how to interpret the logistic regression results. The interpretation of such coefficients is not on the yield rate changes directly but a change in the odds of motorists yielding (odds are defined as the ratio of the number of yielding motorists to the number of non-yielding motorists). The regression coefficients can be transformed and interpreted as odds ratios of different levels of the corresponding independent variable. In other words, the odds ratio is the expected change in the odds of motorists yielding per unit change of the independent variable. More details on these types of models can be found in the literature.(47) All the statistical analyses were performed using R, an open-source statistical language, and environment and two open-source packages for fitting GLMMs.(48,45)
From the preliminary review of the results in table 57, it appears that there were only minor, if any, differences between the tested flash patterns. The results from the GLMM are shown in table 58. Statistical significance of coefficients was obtained from comparing the coefficient (i.e., parameter estimate) to a value of zero. If an estimate is found to be statistically different from zero, then the variable has a statistically significant effect on the odds of driver yielding. Additionally, if the coefficient is different from zero, then the odds ratio is different from 1. Conversely, coefficients without statistical significance indicate an odds ratio indistinguishable from one, thus indicating that the variable has no bearing on driver yielding rate. In this study, the reference level for a driver yielding in the model was estimated as follows: temporary light bar with a 2-5 flash pattern in College Station, TX.
| Variable | Estimate | Standard Error | DF | t-value | p-value |
|---|---|---|---|---|---|
| Referencea | 1.3864637 | 0.9582977 | 941 | 1.4467986 | 0.1483 |
| Temporary; blocks | 0.1662325 | 0.1503383 | 941 | 1.1057233 | 0.2691 |
| Temporary; WW+S | 0.1164097 | 0.1452238 | 941 | 0.8015884 | 0.4230 |
| Garland, TX | 0.8213472 | 0.5663119 | 5 | 1.4503443 | 0.2067 |
| Crossing distance (ft) | -0.0090980 | 0.0184713 | 5 | -0.4925464 | 0.6432 |
Estimate = Natural logarithm of the ratio = odds (coefficient level)/odds (reference level). In the case of reference level, estimate is the log-odds of the average yielding rate at the reference level.
t-value = Conservative estimate of the z-value, which is the standard normal score for the estimate, given the hypothesis that the actual odds ratio equals 1.
p-value = Probability that the observed log-odds ratio is at least as extreme as the estimate, given the hypothesis that the actual odds ratio equals 1.
aReference level driver yielding in the model is estimated for the following conditions: 2-5 flash pattern used with temporary light bars in College Station, TX.
Because a previous study on RRFBs found that posted speed limit, crossing distance, and city influenced driver yielding, the analysis considered those variables initially. However, for this set of sites, posted speed limit and crossing distance were correlated; therefore, posted speed limit was removed. Site selection was heavily influenced by whether four lanes were present and whether the beacons were located on the roadside rather than overhead. In other words, site selection was not a function of the posted speed limit and crossing distance, and a high number of sites had one posted speed limit (40 mi/h for six of the eight sites), which did not provide a sufficient range for parameter estimation on that variable. The city (Garland, TX, or College Station, TX) was included as a fixed effect, with the results shown in table 59. Both city and crossing distance were found to be not significant for this dataset.
The p-values from table 58 were adjusted to allow multiple comparisons, as shown in table 59. The table indicates that there were no significant differences between the 2-5 flash pattern and the WW+S flash pattern (p-value = 0.707), between the 2-5 flash pattern and the blocks flash pattern (p-value = 0.517), between the blocks flash pattern and WW+S flash pattern (p-value = 0.941), or between blocks, WW+S, or the 2-5 flash pattern (p-value = 0.516).
| Hypothesis | Estimate | Standard Error | z-value | Pr( >|z|)a |
|---|---|---|---|---|
| Temporary; blocks - Temporary 2-5 flash pattern = 0 | 0.16623 | 0.14994 | 1.109 | 0.517 |
| Temporary; WW+S - Temporary; 2-5 flash pattern = 0 | 0.11641 | 0.14484 | 0.804 | 0.707 |
| Temporary; blocks - Temporary; WW+S = 0 | 0.04982 | 0.14872 | 0.335 | 0.941 |
| (Temporary; blocks and Temporary; WW+S) - (Temporary; 2-5 flash pattern) = 0 | 0.14132 | 0.12728 | 1.11 | 0.516 |
aAdjusted p-values were reported using a single-step method.
The previous evaluation kept the temporary light bar constant, while this evaluation kept the 2-5 flash pattern constant. Comparing the results between the 2-5 flash pattern used with the temporary light bars and the results when the 2-5 flash pattern was used with the existing equipment indicates that a difference may exist. As shown in table 57, the average yielding for the 2-5 flash pattern with temporary light bars was 78 percent, while the average yielding for the existing equipment was slightly higher at 81 percent. Overall, the driver yielding rates were higher for the existing light bars for the Garland, TX, sites, and the driver yielding rates were lower for the existing light bars for the College Station, TX, sites.
Table 60 shows the results for the LMM, which found that the equipment (p-value = 0.0010) and the city (p-value = 0.0205) were both significant. Because these statistical significant differences existed, they indicate that characteristics of the city, the roadway, and the beacons other than flash pattern had an effect on driver yielding. Even with accounting for crossing distance and city, a statistical significant difference was found between the existing and temporary light bars. Therefore, other characteristics that were not measured (i.e., brightness) are possibly influencing a driver's decision to yield or not yield. The reference level driver yielding in the model was estimated as having existing light bars in College Station, TX.
| Variable | Estimate | Standard Error | DF | t-value | p-value |
|---|---|---|---|---|---|
| Referencea | 1.4748929 | 0.8625094 | 644 | 1.71002 | 0.0877 |
| Temporary beacons | -0.5002792 | 0.1516542 | 644 | -3.298814 | 0.0010 |
| Garland, TX | 1.6766262 | 0.5014311 | 5 | 3.343682 | 0.0205 |
| Crossing distance (ft) | -0.0131371 | 0.0166508 | 5 | -0.788978 | 0.4659 |
Estimate = Natural logarithm of the ratio = odds (coefficient level)/odds (reference level). In the case of reference level, estimate is the log-odds of the average yielding rate at the reference level.
t-value = Conservative estimate of the z-value, which is the standard normal score for the estimate, given the hypothesis that the actual odds ratio equals 1.
p-value = Probability that the observed log-odds ratio is at least as extreme as the estimate, given the hypothesis that the actual odds ratio equals 1.
aReference level driver yielding in the model is estimated for the following conditions: existing light bars in College Station, TX.