U.S. Department of Transportation
Federal Highway Administration
1200 New Jersey Avenue, SE
Washington, DC 20590
202-366-4000
Federal Highway Administration Research and Technology
Coordinating, Developing, and Delivering Highway Transportation Innovations
| REPORT |
| This report is an archived publication and may contain dated technical, contact, and link information |
|
| Publication Number: FHWA-HRT-12-023 Date: December 2012 |
Publication Number: FHWA-HRT-12-023 Date: December 2012 |
The mechanistic validation was conducted using linear elastic models to predict the responses of the pavement (stresses, strains and deflections) to load. The software KENPAVE, developed by Huang, was used to model typical flexible and rigid pavement structures.(28) The mechanistic responses were then used to predict performance using the MEPDG.(27)
Several pavement structures were simulated using the KENLAYER computer program, which is part of the KENPAVE software.(28) The intention was to evaluate different asphalt concrete and base layer thicknesses at different stiffnesses and different subgrade moduli. A factorial study was designed and is presented in table 18.
The combination of all parameters in the factorial experiment resulted in 1,296 sections. It was necessary to find a common variable for comparing the mechanistic responses among different combinations of the input parameters. The choice was the SN used in the 1993 AASHTO Guide for Design of Pavement Structures.(8)
The SN gives a quantitative description of the structural capacity of the different layers in a pavement section. It is a function of layer thicknesses, layer coefficients, and drainage coefficients. The layer coefficients are measures of the relative ability of a unit thickness of a given material to function as a structural component of the pavement. The SN and layer coefficients for a three-layer system can be computed using the following equations:
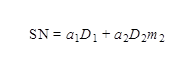
Figure 88. Equation. Computation of SN.
![]()
Figure 89. Equation. Computation of the layer coefficient a2 for base course.
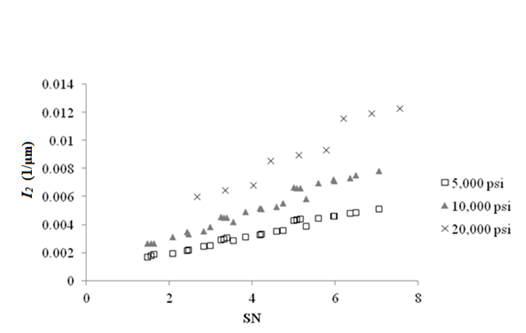
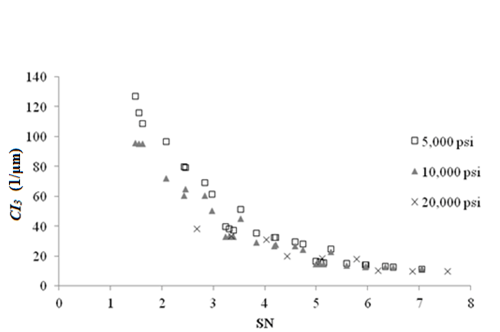
Where a1 and a2 are the layer coefficients of the asphalt concrete and base layers, respectively, D1 and D2 are the layer thicknesses in inches, and m2 is the base layer drainage coefficient, assumed as unity for simplicity. SN is a dimensionless variable. In figure 89, E2 refers to Mr of the base course in pounds per square inch. Figure 90 describes the trends between deflection parameter I2 and the SN. For simplicity, the data were grouped by subgrade modulus. I2 is the deflection parameter chosen for the final structural logistic model based on roughness performance. Figure 91 describes the trends between deflection parameter CI3 and the SN. CI3 is the deflection parameter chosen for the final structural logistic model based on rutting performance. Figure 92 describes the trends between I1 and SN. I1 is the deflection parameter chosen for the final structural logistic model based on fatigue cracking.
1 1/μm = 25.4 1/mil
1 psi = 6.89 kPa
Figure 90. Graph. Deflection parameter I2 as a function of SN for a flexible pavement with HMA modulus of 500,000 psi (3,445,000 kPa).
1 1/μm = 25.4 1/mil
1 psi = 6.89 kPa
Figure 91. Graph. Deflection parameter CI3 as a function of SN for a flexible pavement with HMA modulus of 500,000 psi (3,445,000 kPa).

1 1/μm = 25.4 1/mil
1 psi = 6.89 kPa
Figure 92. Graph. Deflection parameter I1 as a function of SN for a flexible pavement with HMA modulus of 500,000 psi (3,445,000 kPa).
Comparison with MEPDG Predicted Performance
The MEPDG performance prediction models were used to compare predicted distress performance at the end of the design life with the estimated structural condition obtained from the logistic structural model. This task was accomplished by applying the MEPDG models using the mechanistic responses calculated by KENPAVE.
Two models were considered-rutting and fatigue cracking. At first, the roughness model was also in the scope. However, this particular model requires additional site factors that often dominate the analysis. Therefore, comparison between the outcome of the structural logistic model based on roughness and predicted roughness performance was not included in this exercise. The calculations were performed in Microsoft Excel® and not within the MEPDG software due to computational time required to run all scenarios. Since the objective is the comparison and trend evaluation, this assumption had no impact on the outcome or conclusions.
The equation in figure 93 predicts the number of load repetitions to fatigue cracking failure, where is the tensile strain at the bottom of the asphalt surface layer and ει is the elastic modulus of the asphalt layer. k'1 and C are parameters calculated using equations in figure 94 through figure 96 where Va is the percentage of air voids and Vb is the percentage of binder content
![]()
Figure 93. Equation. Calculation of number of load repetitions to fatigue failure.
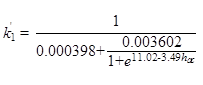
Figure 94. Equation. Calculation of correction for different asphalt layer thickness effects.
![]()
Figure 95. Equation. Calculation of laboratory to field adjustment factor.
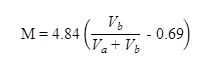
Figure 96. Equation. Calculation of parameter M.
The damage related to fatigue cracking, D, is computed using the Miner's law and bottom-up cracking in percentage of total lane area is obtained through the equation in figure 97 in which C1, C'1, C2 and, C'2 are constants.
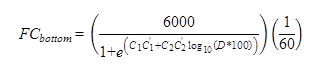
Figure 97. Equation. Fatigue cracking prediction.
Figure 32 describes the sensitivity of I1 structural logistic model probability based on fatigue cracking to MEPDG predicted fatigue cracking performance for flexible pavements. The trends agree with expectations; high probabilities of good structural condition are associated with low levels of fatigue cracking. As the probability decreases, the predicted area of fatigue cracking increases.
The analysis using the rutting model was simplified, and only rutting at the asphalt concrete surface layer was used. The current MEPDG model for asphalt concrete rutting is provided in figure 98 where εp is the plastic strain, εr is the elastic strain, T is temperature (Fahrenheit), N is the number of load applications, and k1 is a constant that depends on depth at which the elastic strain is calculated and is given by equations in figure 99 and figure 100. The total rutting in the asphalt layer is calculated by integrating the calculated plastic strain over the thickness of the layer.
![]()
Figure 98. Equation. Calculation of permanent deformation.

Figure 99. Equation. Calculation of k1.
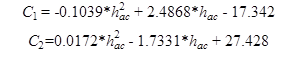
Figure 100. Equation. Calculation of C1 and C2.
Figure 101 describes the sensitivity of CI3 structural logistic model probability based on rutting to MEPDG predicted AC rutting performance for flexible pavements. In this plot, the trends agree with expectations; high probabilities of good structural condition are associated with small permanent deformation. As the probability decreases, predicted AC rutting increases.
|
|
| 1 inch = 25.4 mm 1 psi = 6.89 kPa |
Figure 101. Graph. Sensitivity of CI3 structural logistic model probability based on rutting performance to MEPDG predicted rutting for flexible pavements.
Several pavement structures were simulated using the KENSLAB computer program, which is part of the KENPAVE software. The intention was to evaluate different JPCP slab thicknesses and subgrade moduli and the consequences to the outcome of the structural logistic models. A factorial study was designed and is presented in table 19.
The deflection parameters of the best logistic models developed were computed for the structures simulated in the factorial experiment. Only the FWD test at the center of the slab was evaluated. The objective was to verify if trends would agree with expected outcomes from the models. At first, the deflection parameters were plotted against the slab thickness (i.e., considered as reference for the strength of the pavement section). Figure 31 shows the trends between deflection parameter I1 and slab thickness for three subgrade moduli. I1 was chosen for the final structural logistic model based on roughness performance. The results indicate that I1 increased as slab thickness increased, as expected.
Figure 102 shows the trends between deflection parameter D6 and slab thickness. D6 was chosen for the final structural logistic model based on faulting performance. D6 is decreased as slab thickness increased, as expected. However, the sensitivity reduced when the subgrade modulus was stiffer.
|
1 psi = 6.89 kPa |
Figure 102. Graph. Sensitivity of deflection parameter D6 to slab thickness.
Figure 103 shows the trends between CI4 and slab thickness. CI4 was chosen for the final structural logistic model based on transverse slab cracking. CI4 is sensitive to slab thickness, and the trend indicates that the value decreased as slab thickness increased, as expected.
|
1 psi = 6.89 kPa |
Figure 103. Graph. Sensitivity of deflection parameter CI4 to slab thickness.
Comparison with MEPDG Predicted Performance
In the case of rigid pavements, the MEPDG was used to generate the performance predictions. There were only 15 scenarios to run, and the rigid pavement analysis was performed much faster than the flexible pavement analysis.
Figure 33 shows the sensitivity of I1 structural logistic model probability based on roughness to the MEPDG predicted roughness performance for rigid pavements. It can be seen that the trends agree with expectations; high probabilities of good structural condition are associated with low values of IRI. As the probability decreased, the predicted IRI increased. This plot confirms that the structural logistic model is capable of providing an assessment of structural condition that is tied to an expectation of performance.
Figure 104 shows the sensitivity of D6 structural logistic model probability based on faulting to MEPDG predicted faulting performance for rigid pavements. Again in this plot, the trends agree with expectations; high probabilities of good structural condition are associated with low faulting values. As the probability decreases, predicted joint faulting increases. It is important to note that in this particular model, although the trends agree with expectation, the variation in probability of acceptable structural condition is small when compared to previous models.
|
|
| 1 psi= 6.89 kPa |
Figure 104. Graph. Sensitivity of D6 structural logistic model probability based on faulting performance to MEPDG predicted faulting for rigid pavements.
Figure 105 shows the sensitivity of CI4 structural logistic model probability based on slab cracking to MEPDG predicted slab cracking performance for rigid pavements. The x-axis is presented in log scale to improve the visualization. The trends agree with expectations; high probabilities of good structural condition are associated with low to no slab cracking. As the probability decreases, predicted percentage of cracked slab increases.
|
|
Figure 105. Graph. Sensitivity of CI4 structural logistic model probability based on slab cracking performance to MEPDG predicted slab cracking for rigid pavements.