U.S. Department of Transportation
Federal Highway Administration
1200 New Jersey Avenue, SE
Washington, DC 20590
202-366-4000
Federal Highway Administration Research and Technology
Coordinating, Developing, and Delivering Highway Transportation Innovations
| REPORT |
| This report is an archived publication and may contain dated technical, contact, and link information |
|
| Publication Number: FHWA-HRT-15-036 Date: December 2015 |
Publication Number: FHWA-HRT-15-036 Date: December 2015 |
This chapter illustrates the use of the backcalculated elastic layer modulus values for a range of topics related to day-to-day design applications as well as for research purposes. These examples demonstrate the application of the backcalculated elastic moduli and not a detailed or complete analysis related to any one topic.
Histograms can be prepared to determine the spread and mean for a particular material or pavement layer. Figure 5 and figure 6 in chapter 3 are examples of a test section basis for one of the case studies. These histograms can be used for judging the reasonableness of the results, identifying outliers, and comparing the mean value to the default value included in the MEPDG for that material.(1) Additionally, figure 35 and figure 36 show a histogram of the GB layers or aggregate bases classified as an AASHTO A-1-a material from all LTPP test sections in Georgia and Minnesota. The overall distribution has a positive skew for both sets of data with a mean of about 28 ksi for the Georgia sections and 23 ksi for the Minnesota sections.
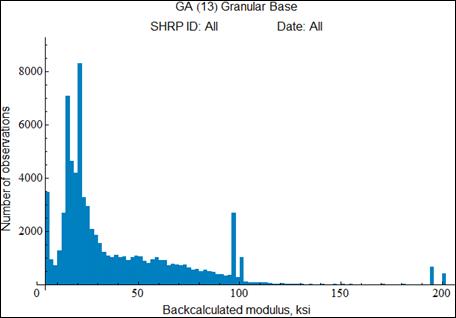
Figure 35. Graph. Backcalculated elastic moduli for all aggregate base layers for the Georgia (GA(13)) LTPP test sections classified as an AASHTO A-1-a material.
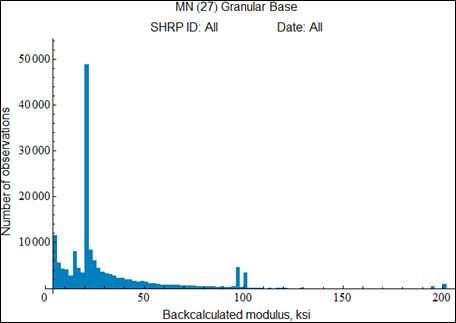
Figure 36. Graph. Backcalculated elastic moduli for all aggregate base layers for the Minnesota (MN(27)) LTPP test sections classified as an AASHTO A-1-a material.
The default resilient modulus included in the MEPDG is 40 ksi for an AASHTO A-1-a base, 30 ksi for crushed stone, and 25 psi for crushed gravel.(1) The values included in the MEPDG are for optimum conditions, and it is expected that optimum conditions do not exist in the field over time. The mean value from the distribution shown in figure 35 and figure 36 is probably more similar to the representative value that can be entered in the MEPDG—an average weighted value throughout the year.
There are outliers in the Georgia and Minnesota datasets of around 100 and 200 ksi; however, these values are believed to represent a frozen or partially frozen base layer. In addition, a fairly large number of elastic modulus values were computed around 5 ksi, which was the lower limit set during the backcalculation process. Although these values were previously eliminated during the first round of backcalculation from the LTPP database as being too low, they are believed to be representative of saturated or wet conditions, when the frozen layer thaws or water enters the aggregate base layer through lateral flow of water or cracks in the pavement surface. Similarly, the high values were also eliminated from the first round of backcalculation but may be accurate in the case where the base layer is very dry.
Most unbound aggregate base materials and soils are sensitive to changes in the applied stress, and the resilient modulus is dependent on the stress state or level. Coarse-grained aggregate base materials and coarse-grained soils generally exhibit a stress hardening pattern where the resilient modulus increases with increasing stress level, while most fine-grained soils exhibit a stress softening pattern where the resilient modulus decreases with increasing stress level. Some sands or soils at the transition between fine and coarse-grained soils exhibit no stress sensitivity in the practical range of applied stresses.
Deflection basins were measured over four drop heights on all LTPP test sections to determine the nonlinearity behavior of the unbound layers. Backcalculating the nonlinearity behavior or properties of the unbound layers was an objective during the first round of backcalculation. Determining the nonlinearity of the unbound layers was found to be problematic and resulted in a poor success rate. This round of backcalculation focused on using linear elastic methods to calculate the elastic moduli for each drop height. The layer moduli from each drop height could then be used to determine the nonlinearity coefficients of the selected constitutive equation.
Three SPS-8 projects (New York, Texas, and Utah) were used to demonstrate the variation in backcalculated elastic moduli for the four drop heights used in the LTPP deflection basin testing program. The SPS-8 projects were used in this demonstration because these sections generally have very low truck traffic and thin HMA layers—conditions that should exhibit a layer’s nonlinearity response, if present. In other words, the differences in the in-place stresses from drop heights 1 to 4 should be the greatest because the HMA is thin for these sections.
Figure 37 through figure 45 show the change in elastic layer moduli between drop heights 1 to 4 for the aggregate base, weathered soil, and subgrade at the SPS-8 project sites. The average backcalculated elastic moduli were determined for 3 to 4 test days at each site. As shown, most of the layers did not exhibit a constant change in elastic moduli with increasing drop height or stress level, with the exception of the aggregate base at the Texas site and the coarse-grained SS at the New York site. Most of the unbound layers at these three sites exhibited no stress sensitivity or the moduli increased and then decreased, making the determination of the nonlinear coefficients of a constitutive equation difficult. In specific, the change in modulus between the drop heights was considered insignificant relative to the variation or standard deviation of moduli along the test section (not illustrated in the figures) as well as with time.
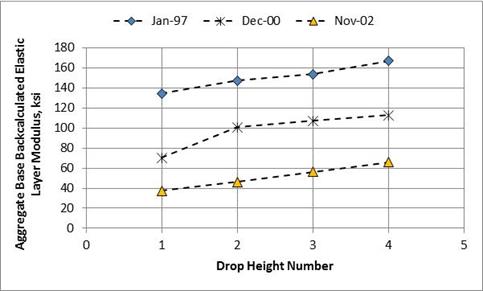
Figure 37. Graph. Comparison of backcalculated elastic moduli for the aggregate base layer from the Texas SPS-8 project 48-0801.
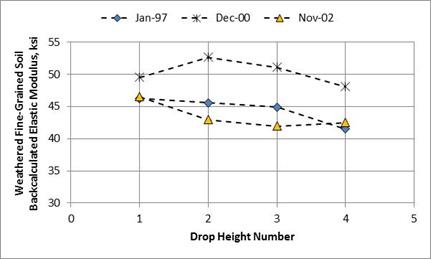
Figure 38. Graph. Comparison of backcalculated elastic moduli for the weathered fine-grained soil layer from the Texas SPS-8 project 48-0801.
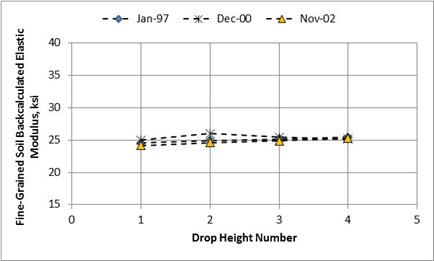
Figure 39. Graph. Comparison of backcalculated elastic moduli for the fine-grained subgrade from the Texas SPS-8 project 48-0801.
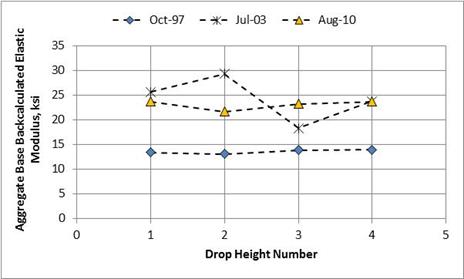
Figure 40. Graph. Comparison of backcalculated elastic moduli for the aggregate base layer from the Utah SPS-8 project 49-0803.
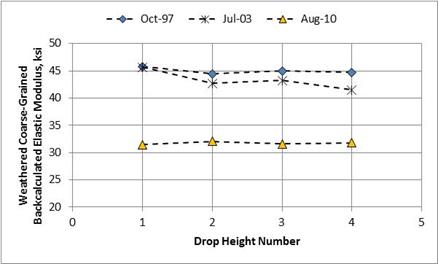
Figure 41. Graph. Comparison of backcalculated elastic moduli for the weathered coarse-grained layer from the Utah SPS-8 project 49-0803.
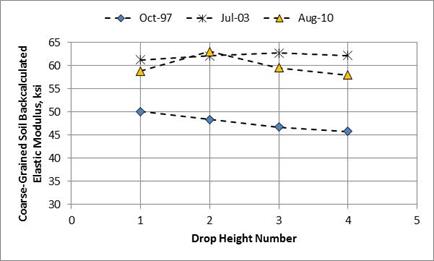
Figure 42. Graph. Comparison of backcalculated elastic moduli for the coarse-grained subgrade from the Utah SPS-8 project 49-0803.
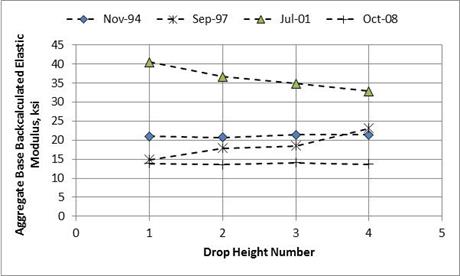
Figure 43. Graph. Comparison of backcalculated elastic moduli for the aggregate base layer from New York SPS-8 project 36-0802.
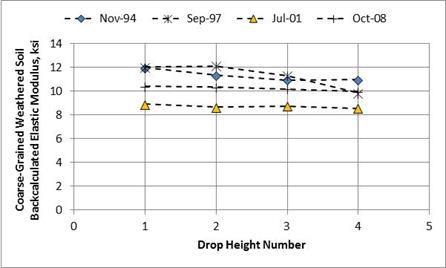
Figure 44. Graph. Comparison of backcalculated elastic moduli for the weathered coarse-grained layer from the New York SPS-8 project 36-0802.
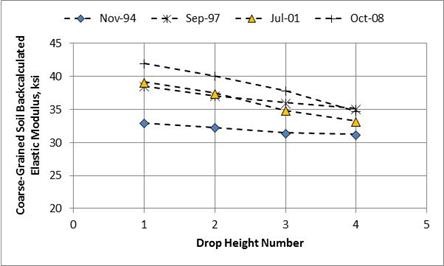
Figure 45. Graph. Comparison of backcalculated elastic moduli for the coarse-grained subgrade from the New York SPS-8 project 36-0802.
One of the unique components of the MEPDG methodology for rehabilitation design is the characterization of the in-place asphalt layers in terms of damage.(1) The MEPDG method uses backcalculated elastic layer moduli of the HMA or bituminous layer to determine the amount of damage in the existing asphalt layers by comparing the in-place backcalculated elastic layer modulus to that of an intact undamaged modulus measured in the laboratory. The damage is defined as the ratio of the backcalculated elastic layer modulus to the laboratory-measured dynamic modulus. The greater the damage or the lower the ratio, the greater the structural thickness required for an overlay. If this concept is correct, the backcalculated moduli should decrease over time as damage starts to accumulate.
Figure 46 illustrates the change in modulus over time for LTPP GPS site 27-6251. As shown, the elastic layer moduli are about the same between 1990 and 1993, while there is a significant decrease in elastic moduli for 2003 when the pavement is about 10 years older. This decrease or softening in the moduli is defined as damage by the MEPDG.(1) Conversely, figure 47 illustrates no change in moduli and no damage or softening between 1990 and 1997 for LTPP GPS site 27-1018. In other words, this section did not exhibit any damage between 1990 and 1997. Thus, the LTPP backcalculated elastic layer moduli can be used to confirm or reject this damage concept determination and its use for pavement evaluation and rehabilitation design.

Figure 46. Graph. Decreasing elastic moduli of the asphalt layer over time for use in rehabilitation design for Minnesota GPS section 27-6251.
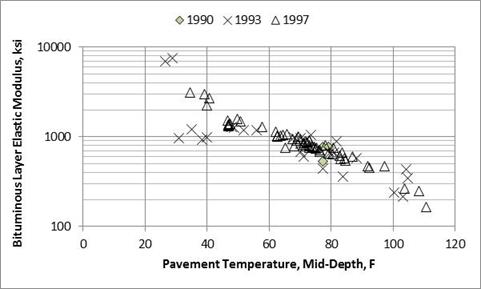
Figure 47. Graph. Decreasing elastic moduli of the asphalt layer over time for use in rehabilitation design for Minnesota GPS section 27-1018.
It can be seen that the backcalculated elastic layer modulus values were about the same at colder temperatures during the winter months and approached each other at the higher temperatures during the summer months. The difference in moduli over time is exhibited within the intermediate temperature range. Figure 48 and figure 49 illustrate the change in modulus within a narrow temperature range of 60 to 66 ºF for Minnesota section 27-1016 and section 27-6251, respectively. As shown, there is a continuous decrease in the elastic modulus from the deflection basin tests conducted between these temperatures. Thus, the time of year for measuring the amount of in place damage is probably important. If this observation holds true for many other LTPP test sections, the mathematical relationship used in the MEPDG for calculating damage may need to be revised to be temperature dependent.(1)
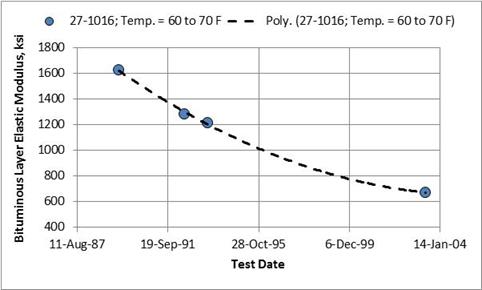
Figure 48. Graph. Decreasing elastic moduli of the asphalt layer between 60 and 66 ºF over time for use in rehabilitation design for Minnesota GPS section 27-1016.

Figure 49. Graph. Decreasing elastic moduli of the asphalt layer between 60 and 66 ºF over time for use in rehabilitation design for Minnesota GPS section 27-6251.
Minnesota section 27-6251 exhibited significant damage as defined by the MEPDG, while Minnesota section 27-1018 did not exhibit any damage.(1) Deflection basins in LTPP were measured in the wheel paths (lane F3) as well as out of the wheel paths (lane F1) to determine if the load-response properties were different between the two areas. If the damage concept is correct, then the measurements within the wheel path should be statistically different than the non-wheel path measurements for the test section exhibiting damage, and there should be no difference between the two lines for the section not exhibiting damage.
Figure 50 and figure 51 include a comparison of the backcalculated elastic layer moduli for the wheel path and non-wheel path areas for these two LTPP sections in Minnesota. As shown, a significant difference was observed for GPS section 27-6251, and no difference was observed for GPS section 27-1018. This observation provides support for the damage concept incorporated within the MEPDG.(1) If this concept holds true for many more LTPP sections, this will make it easier for agencies to determine whether there is a difference in the loaded and non-loaded areas of the pavement by simply testing along two lanes, reducing the number of cores that are now required to determine the in-place damage for rehabilitation design and managing an agency’s roadway network for planning future rehabilitation projects.
This observation by itself should be of significant value to agencies for improving their management prediction and planning capabilities. Simply measuring the deflection basins in the wheel path versus outside the wheel path provides a comparison of elastic moduli and whether damage is starting to occur. As extensive surface cracking starts to occur and spread beyond the wheel paths, however, any difference between measurements made in and outside the wheel paths is expected to decrease.
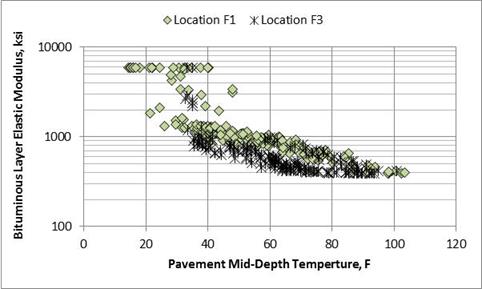
Figure 50. Graph. Elastic moduli of the asphalt layer between the wheel path and non-wheel path lanes for Minnesota GPS section 27-6251.
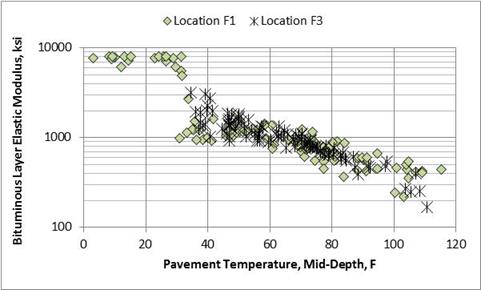
Figure 51. Graph. Elastic moduli of the asphalt layer between the wheel path and non-wheel path lanes for Minnesota GPS section 27-1018.
The MEPDG software requires the entry of laboratory-measured moduli.(1) This section compares the backcalculated elastic layer moduli to laboratory-measured moduli.
Elastic moduli were calculated and are in the CPTs in the LTPP database for all HMA-surfaced test sections. The projects included in the SPS-1 experiment were used to compare the laboratory-derived dynamic moduli and field-derived elastic moduli of the HMA layers for four test sections in Florida, Montana, New Mexico, and Wisconsin. The deflection basin test days used in this analysis were the first three recorded for each project because it is expected that no damage should have occurred shortly after the mixtures were placed. In addition, the backcalculated elastic moduli were derived from the deflection basins measured outside the wheel path to further reduce any possibility of damage skewing the comparison.
Figure 52 compares the laboratory-computed dynamic moduli using the Witczak regression equation to the backcalculated elastic moduli. A loading frequency of 20 Hz was used in the comparison. In addition, the mid-depth temperature for the layer in question was used to estimate the laboratory-derived value. As shown, there is a slight bias in the backcalculated moduli—slightly lower moduli were computed from the deflection basins in comparison to the laboratory-derived values computed with the dynamic modulus regression equation. This bias could be related to the assumed loading frequency and/or temperature used to estimate the laboratory-derived values. It could also be related to the thickness of the HMA layer used in the backcalculation process.
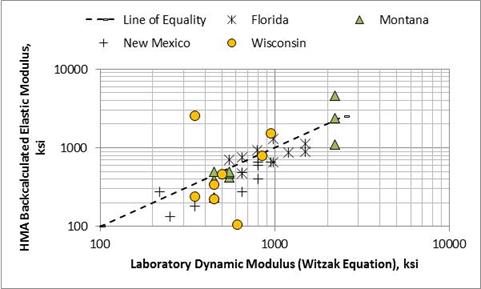
Figure 52. Graph. Comparison of the dynamic moduli computed from the Witczak regression equation and the backcalculated elastic moduli for four SPS-1 projects.
It is the authors’ opinion that temperature is not the controlling factor causing the bias, but load frequency and thickness could be contributing factors. It is suggested that the LTPP backcalculated elastic moduli can be compared to the dynamic moduli calculated using Witczak’s or another regression equation for making a recommendation on the loading frequency to be used and determine whether it is thickness dependent. By defining the equivalent load frequency and whether it is dependent on some other factor, no adjustments need to be made to the backcalculated elastic layer moduli for the HMA or bituminous layers.
Static elastic moduli were measured in the laboratory for most of the PCC mixtures placed on the SPS-2 projects. The projects included in the SPS-2 experiment were investigated to compare the laboratory equivalent and field-derived elastic moduli of the PCC layers for four test sections. The deflection basin test days used in this analysis were the first ones recorded for each project after construction because it was expected that no damage occurred shortly after the mixtures were placed. The first deflection basin measurements for many of the SPS-2 projects, however, were taken more than 1 year after placement. As such, the elastic moduli measured on the GPS test sections were compared to the backcalculated moduli because both represent long-term properties. As was shown in figure 16, there was extensive variation in the backcalculated moduli, but the bias was minimal. One observation from the comparison is that the backcalculated elastic PCC moduli from EVERCALC© and MODCOMP© need to be adjusted to remove the bias for estimating laboratory-measured static elastic moduli and results from the best fit unbonded condition method. It is suggested that more sites be included in the comparison to confirm or reject the hypothesis.
The case study sites were used to compare the laboratory-derived resilient modulus of the unbound layers at equivalent stress states under FWD testing to the average backcalculated elastic moduli. The resulting c-factors were found to be similar to the values reported in the literature as well as the default c-factors recommended for use in the MEPDG.(1) No further analyses comparing laboratory-derived resilient moduli to field-derived elastic moduli were completed under this study to determine if the c-factor was dependent on other factors.
The backcalculated elastic layer moduli for each pavement layer were reviewed from selected sites in the SMP experiment to evaluate the change in values over time or by month. Four SMP sites were used: two from a cold environment (Idaho (16-1010) and Minnesota (27-1018)) and two from a warm environment (New Mexico (35-1112) and Texas (48-1077)).
Figure 53 through figure 56 show the backcalculated elastic layer moduli for the HMA layers from the four SMP sites. The backcalculated elastic moduli for the two SMP sites located in a warm climate had significantly lower elastic moduli during the winter months in comparison to the SMP sites located in a cold climate. The elastic moduli calculated for the winter months in the cold climates exceeded values that were considered too large in comparison to typical values measured in the laboratory. This represents a significant difference between the laboratory-derived and field-derived moduli during the winter months that should be investigated. It was expected that a partially frozen layer directly beneath the HMA had an effect on the HMA field-derived moduli.
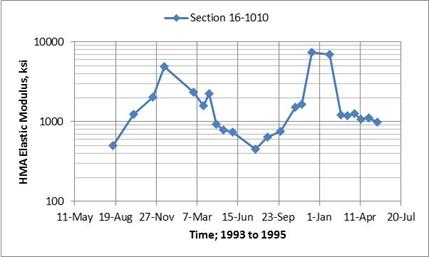
Figure 53. Graph. Comparison of HMA backcalculated elastic layer moduli for Idaho SMP section 16-1010.
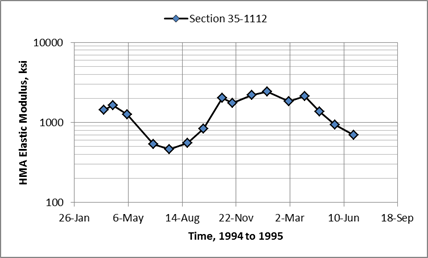
Figure 54. Graph. Comparison of HMA backcalculated elastic layer moduli for New Mexico SMP section 35-1112.
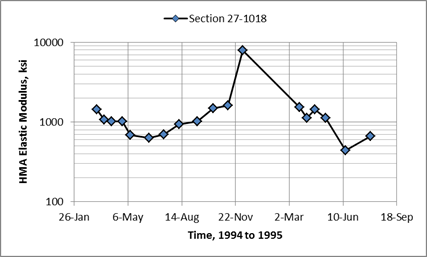
Figure 55. Graph. Comparison of HMA backcalculated elastic layer moduli for Minnesota SMP section 27-1018.
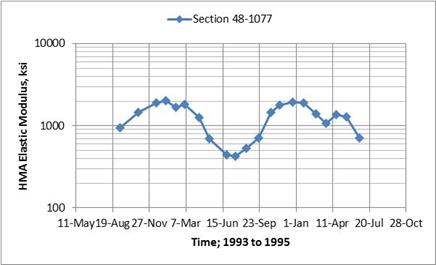
Figure 56. Graph. Comparison of HMA backcalculated elastic layer moduli for Texas SMP section 48-1077.
Figure 57 through figure 60 show the backcalculated elastic layer moduli for the unbound aggregate base from the four SMP sites. The two SMP sites located in a cold climate had very high elastic moduli during the winter months that were representative of a frozen or partially frozen layer (Idaho section 16-1010 and Minnesota section 27-1018). For the two SMP sites located in a hot climate, the elastic modulus was significantly higher during the summer months in comparison to the winter months (New Mexico section 35-1112 and Texas section 48-1077). The water content of the aggregate base layers in the summer months in a hot dry climate can be lower in comparison to the winter months and result in higher modulus values.
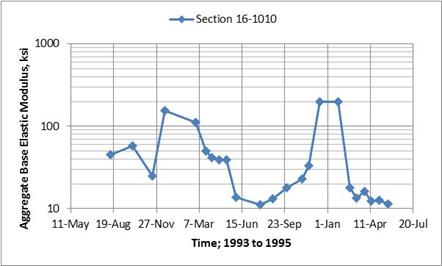
Figure 57. Graph. Comparison of aggregate base backcalculated elastic layer moduli for Idaho SMP section 16-1010.
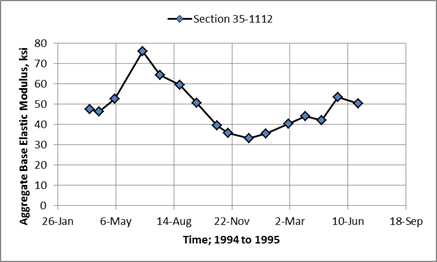
Figure 58. Graph. Comparison of aggregate base backcalculated elastic layer moduli for New Mexico SMP section 35-1112.
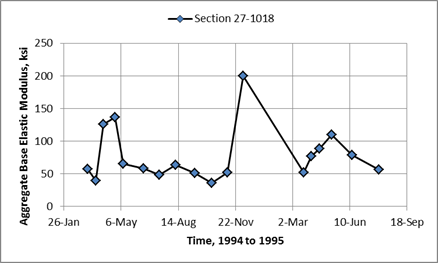
Figure 59. Graph. Comparison of aggregate base backcalculated elastic layer moduli for Minnesota SMP section 27-1018.
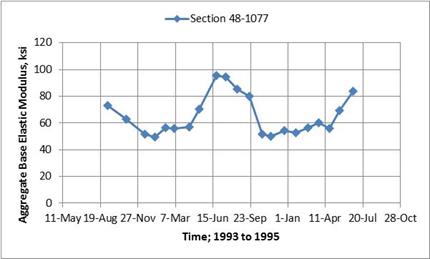
Figure 60. Graph. Comparison of aggregate base backcalculated elastic layer moduli for Texas SMP section 48-1077.
Figure 61 through figure 63 show the backcalculated elastic layer moduli for the weathered soil layers at three of the SMP sites (Idaho, Minnesota, and Texas). A weathered layer was not included in the New Mexico SMP site because a bedrock or very stiff layer existed at this site. The two SMP sites located in a cold climate had very high elastic moduli during the winter months that were representative of a frozen or partially frozen layer (Idaho section 16-1010 and Minnesota section 27-1018). For the SMP site located in a hot climate with a weathered soil layer, the elastic modulus was significantly higher during the summer months in comparison to the winter months (Texas section 48-1077). The water content of the weathered soil in the summer months in a hot dry climate can be lower in comparison to the winter months and result in higher modulus values.
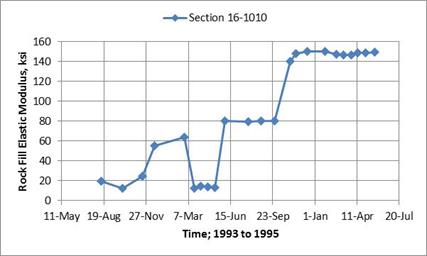
Figure 61. Graph. Comparison of weathered soil backcalculated elastic layer moduli for Idaho SMP section 16-1010.
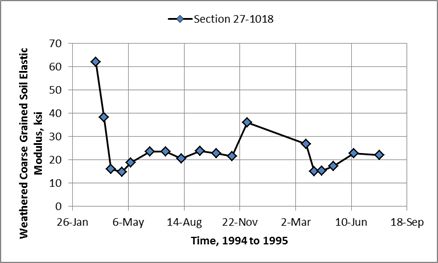
Figure 62. Graph. Comparison of weathered soil backcalculated elastic layer moduli for Minnesota SMP section 27-1018.
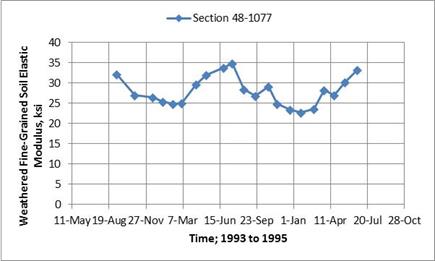
Figure 63. Graph. Comparison of weathered soil backcalculated elastic layer moduli for Texas SMP section 48-1077.
The Idaho SMP site included a thick rock fill. The elastic modulus of this layer steadily increased over time to a value of about 150 ksi. The authors completed deflection testing and backcalculation of elastic layer moduli using EVERCALC© on other commercial-type projects that included rock fills located in central Texas and Wyoming. Similar results were obtained from unpublished design reports, with the in-place elastic moduli varying from about 80 to over 150 ksi. However, time-dependent results from these other projects were unavailable. Rock fills have been placed at other LTPP test section locations and should be investigated to determine if they exhibit this same time-dependent characteristic and magnitude of in-place elastic moduli.
Figure 64 through figure 67 show the backcalculated elastic layer moduli for the SS at the four SMP sites. The two SMP sites located in a cold climate had higher elastic moduli during the winter months that would be representative of a partially frozen layer. For the two SMP sites located in a hot climate, the elastic modulus was about the same between the summer and winter months. Significant variations in water content of the deeper SSs do not usually occur unless there is an external factor causing the change in the water content, such as the lateral flow of water or seasonal perched water tables.
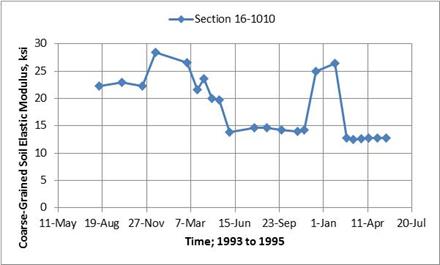
Figure 64. Graph. Comparison of SS backcalculated elastic layer moduli for Idaho SMP section 16-1010.
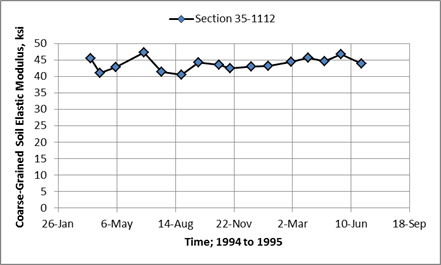
Figure 65. Graph. Comparison of SS backcalculated elastic layer moduli for New Mexico SMP section 35-1112.

Figure 66. Graph. Comparison of SS backcalculated elastic layer moduli for Minnesota SMP section 27-1018.

Figure 67. Graph. Comparison of SS backcalculated elastic layer moduli for Texas SMP section 48-1077.
The SPS-1 and -5 test sections were investigated to determine the increase in elastic moduli over time and if the values could be used to estimate the hardening that occurs with time. Figure 52 shows a comparison of the laboratory-derived dynamic moduli to the field-derived elastic moduli for the first 3 test days when the majority of the hardening occurred after HMA placement. The HMA layers were combined for most of the SPS-1 test sections and represent weighted or composite values for all of the HMA lifts or layers. Even for the SPS-5 test sections where the overlay was represented as a separate layer, there was no clear increase in the field-derived elastic moduli over time. More importantly, the HMA elastic layer moduli were heavily influenced by temperature, so the increase in HMA elastic modulus through hardening could not be determined because of the variability in the computed results.
The PCC backcalculated elastic layer moduli from selected SPS-2 test sections were used to determine if these values could be used to confirm the modulus-time or strength gain relationship included in the MEPDG software.(1) Figure 68 and figure 69 show the backcalculated elastic layer moduli from the EVERCALC© program and best fit method, respectively, for multiple SPS-2 test sections. As shown, there was too much variation in the backcalculated moduli values over time to estimate the strength or stiffness versus time relationship. Figure 70 provides a comparison between the PCC elastic modulus from the best fit unbonded method to the elastic moduli backcalculated with EVERCALC©. The PCC elastic moduli from the unbonded best fit method are consistently higher than from the moduli backcalculated with EVERCALC©.
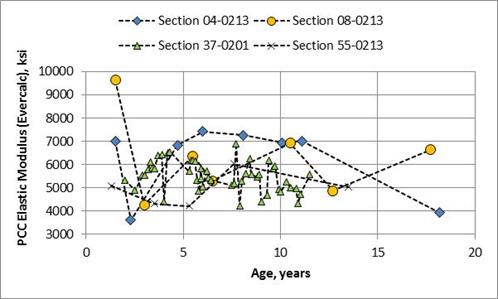
Figure 68. Graph. Backcalculated PCC elastic moduli over time from EVERCALC© for selected SPS-2 test sections.
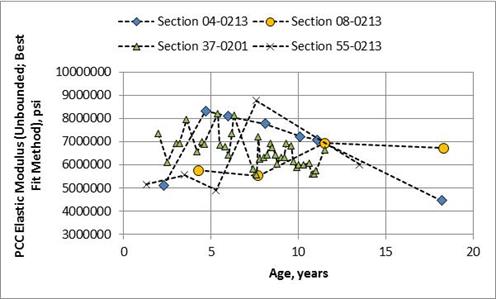
Figure 69. Graph. Backcalculated PCC elastic moduli over time from the best fit unbonded method for selected SPS-2 test sections.
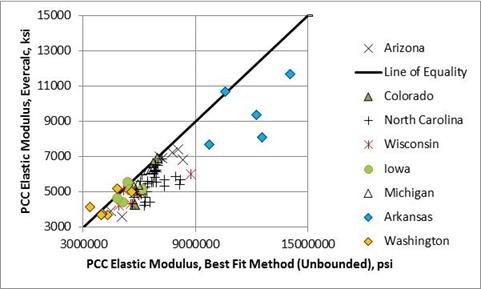
Figure 70. Graph. Comparison of backcalculated PCC elastic moduli from EVERCALC© and the best fit unbonded method for selected SPS-2 test sections.
The MEPDG provides recommended default elastic moduli for fractured PCC slabs, but two questions have been raised regarding the elastic moduli that are representative of the fractured PCC layer: (1) what is the representative elastic modulus after construction, and (2) does this value change over time? The LTPP backcalculated elastic layer moduli from SPS-6 test sections were used to determine if those two questions can be answered. Test sections from Arizona, Indiana, Michigan, Oklahoma, and Pennsylvania were used to compare the backcalculated elastic layer moduli between the fractured and intact PCC slabs. The Arizona, Michigan, and Pennsylvania projects included both crack and seat and rubblized test sections, but the Indiana and Oklahoma projects did not. The Indiana project only included the crack and seat method, while the Oklahoma project only included the rubblization method.
The Oklahoma SPS-6 project was used as one of the case studies because the existing pavement consisted of a JRCP that was rehabilitated using multiple techniques. The first layering simulation included the JRCP as one continuous layer for both the rubblization method as well as for the intact slabs. Table 16 lists the backcalculated layer moduli before and after rehabilitation for the last testing day at this project site. A second run was made for the rubblized section but with a different layering simulation: JRCP was divided into two layers above and below the reinforcement. The table also includes the values resulting from that condition for the last testing day included in the LTPP database.
| Item or Element | Section 0606 Major Restoration | Section 0608 Rubblization | |||
|---|---|---|---|---|---|
| Intact Slabsa | Crack & Seat | Intact Slabsa | One Layer | Two Layers | |
| PCC modulus | 4,799 | 3,615 | 5,082 | 301 | 284 |
| 2,013 | |||||
| Aggregate base modulus | 11.4 | 16.3 | 10.2 | 56.4 | N/A |
| Weathered soil layer modulus | 67.5 | 13.6 | 10.1 | 45.5 | 27.5 |
| Subgrade natural modulus | 33.6 | 32.5 | 37.8 | 34.5 | 35.0 |
| HMA overlay modulus | N/A | 617 | N/A | 848 | 880 |
| Average RMSE | 1.4 | 1.6 | 1.1 | 1.2 | 1.1 |
N/A = Layer not applicable or used in backcalculation process.
aBackcalculated elastic modulus values include those prior to major restoration and rubblization; there was no HMA overlay because deflection basins were measured prior to restoration or rubblization.
The simulation of two PCC layers resulted in about the same RMSE value as for the single layer simulation. The modulus of the upper PCC layer, however, was significantly lower than that of the lower PCC layer. More importantly, the elastic modulus of the upper layer was found to be only slightly lower than that of the single JRCP layer. Thus, it was decided the structure would initially be simulated as one PCC fractured layer. If high RMSE values resulted, then the PCC fractured layer was divided into two layers in an effort to reduce the RMSE.
Figure 71 shows the backcalculated elastic modulus of the rubblized PCC at the Oklahoma SPS-6 site. The elastic modulus of the rubblized PCC was less than 100 ksi after construction, but it steadily increased over time. The PCC moduli for intact slabs of the control section (without an HMA overlay) and the section with maximum restoration activities are also shown in figure 71. The elastic moduli varied from 3 to 6 million psi at this site.
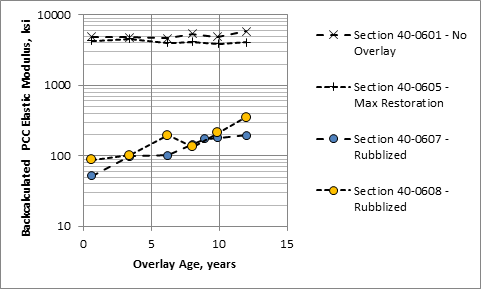
Figure 71. Graph. Backcalculated elastic moduli for the Oklahoma SPS-6 rubblized test sections.
Figure 72 shows the backcalculated elastic layer modulus for the PCC modulus for the Indiana crack and seat sections. As shown, the elastic modulus for the PCC crack and seat was about one-third the modulus of the intact slabs and then increased over time.
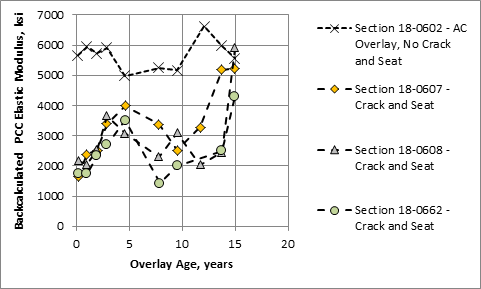
Figure 72. Graph. Backcalculated elastic moduli for the Indiana SPS-6 crack and seat test sections.
Figure 73 through figure 75 compare the backcalculated elastic moduli for the intact, crack and seat, and rubblized test sections from the Arizona, Michigan, and Pennsylvania SPS-6 projects. As shown, the Arizona crack and seat section was the only one where the elastic moduli decreased over time. All other SPS-6 sections stayed about the same or increased over time.
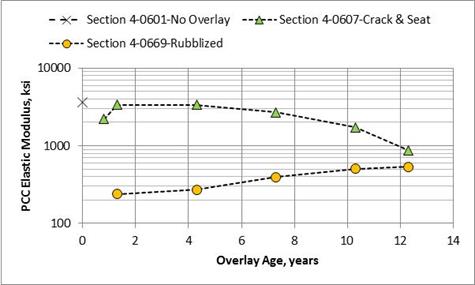
Figure 73. Graph. Backcalculated elastic moduli comparing the PCC intact, crack and seat, and rubblized test sections for the Arizona SPS-6 test sections.
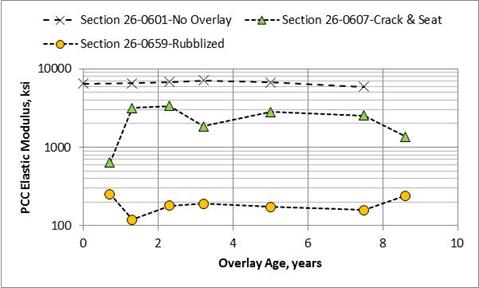
Figure 74. Graph. Backcalculated elastic moduli comparing the PCC intact, crack and seat, and rubblized test sections for the Michigan SPS-6 test sections.

Figure 75. Graph. Backcalculated elastic moduli comparing the PCC intact, crack and seat, and rubblized test sections for the Pennsylvania SPS-6 test sections.
The default values included in the MEPDG for the crack and seat method are 150,000 to 2 million psi, and for the rubblization method the default values are 50,000 to 1 million psi.(1) Both represent a wide range of moduli. The following list summarizes some observations from these SPS-6 projects relative to the range of values included in the MEPDG:(1)
Based on these observations, a detailed evaluation of the backcalculated elastic moduli for the fractured PCC slabs should be conducted for recommending a narrower range of default values included in the MEPDG.(1)
Many agencies have limits on the amount of RAP included in HMA base mixtures as well as for wearing surfaces. The asphalt industry has been pushing the use of higher percentages of RAP in asphalt mixtures in all layers of the pavement. The LTPP SPS-5 experiment was designed to provide data to evaluate the difference between mixtures with about 30 percent RAP and those without RAP. The backcalculated layer elastic modulus was used to evaluate whether there was a difference between the structural responses of the sections with and without RAP. Figure 76 provides a comparison of the RAP and virgin mixture backcalculated elastic layer moduli for the SPS-5 Minnesota project. As shown, there was no significant difference in the structural responses of the asphalt mixtures with and without RAP.
Another important observation from figure 76 is that the backcalculated elastic modulus for the existing asphalt mixture (the control section which was not overlaid) had a significantly higher in-place modulus value than the virgin and RAP mixtures of the overlay. This observation was expected because the existing HMA was much older than the two overlay mixtures, demonstrating the difference that can be caused by long-term aging.
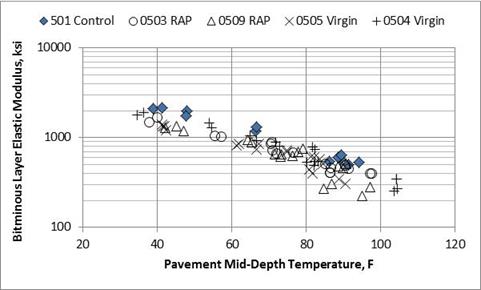
Figure 76. Graph. Comparison of RAP and virgin backcalculated elastic HMA moduli from the Minnesota SPS-5 project.
The backcalculated moduli from four additional SPS-5 projects were used to investigate the difference between the RAP and virgin mixtures in relation to the existing HMA layers. Figure 77 through figure 80 show a comparison of the backcalculated elastic moduli for the different mixtures at four of the SPS-5 projects.
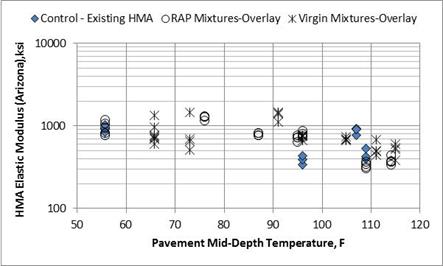
Figure 77. Graph. Comparison of RAP and virgin mix backcalculated elastic HMA moduli from the Arizona SPS-5 project.

Figure 78. Graph. Comparison of RAP and virgin mix backcalculated elastic HMA moduli from the Mississippi SPS-5 project.

Figure 79. Graph. Comparison of RAP and virgin mix backcalculated elastic HMA moduli from the Oklahoma SPS-5 project.
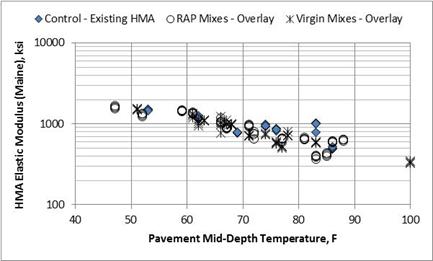
Figure 80. Graph. Comparison of RAP and virgin mix backcalculated elastic HMA moduli from the Maine SPS-5 project.
No significant and consistent difference was observed between the RAP and virgin mixtures used for the overlay. The Maine SPS-5 project exhibited the least difference between the mixtures, while the Arizona SPS-5 project exhibited the least difference across all pavement test temperatures.
The elastic moduli for the existing HMA layer for the Oklahoma project were very high across the entire temperature regime. These results are believed to be reasonable because the binder tests conducted on the extracted asphalt from the existing HMA layer suggest a severely aged material. Conversely, the elastic moduli of the existing HMA layer at the Maine project were only slightly greater than for the overlay mixtures during the summer months. The binder tests conducted on the extracted asphalt for the Maine project did not suggest a severely aged material.
The Mississippi project exhibited a lot of variation for all mixtures. This project experienced high rutting and was taken out of service because of stripping. As noted in chapter 3, it also exhibited high damage in the field-derived moduli.