Enhanced Analysis of Falling Weight Deflectometer Data for Use With Mechanistic-Empirical Flexible Pavement Design and Analysis and Recommendations for Improvements to Falling Weight Deflectometers

CHAPTER 2. LITERATURE REVIEW

This chapter presents a comprehensive review of the status of FWD equipment, market-ready and prototype models, data collection, analysis, and interpretation as they relate to the models and procedures incorporated in the MEPDG.⁽¹⁾

REVIEW OF STATUS OF FWD EQUIPMENT

The FWD is an impulse load deflection device. According to ASTM D‑4694-96, the basic components of an FWD (figure 1) are the following:⁽⁴⁾

Most FWDs are either trailer-towed or vehicle-mounted systems.⁽⁶⁾ Developed in the 1970s, the FWD emerged in the 1980s as the worldwide standard for pavement deflection testing. The equipment of four FWD manufacturers―Grontmij Pavement Consultants, Dynatest®, Foundation Mechanics, Inc. (JILS™), and KUAB―are described in the following sections. The Federal Highway Administration (FHWA) has established four regional FWD calibration centers across the United States to provide annual calibrations of the FWD equipment to ensure the equipment is operating within allowable tolerances.

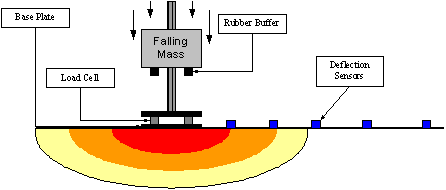
Figure 1. Diagram. FWD testing schematic.

FWD Manufacturers and Equipment

Grontmij Pavement Consultants

The Grontmij Pavement Consultants Group (manufacturers of the Carlo Bro FWD) offers the following three types of FWDs with a modular equipment design ready for upgrade (figure 2):

• The trailer-mounted FWD is mounted to the tow vehicle by a double-axle trailer, and it is supplied with a personal computer (PC), PRIMAX Data Collection FWD software (RoSy DESIGN), time-history module, transport lock, distance-measuring instrument (DMI) integrated in the software, three temperature sensors, 4-split load plate (12 or 18 inches), and 10 to 18 geophones. The system generates forces up to 78,600 lbf.
  
  
• The vehicle-mounted FWD is identical to the trailer-mounted FWD except that it is integrated into a van and has up to 18 geophones.
  
  
• The portable LWD is delivered with 4- and 12-inch-diameter loading plates, a 22‑lb weight, integrated load cell and electronic box, and a center-mounted geophone. Extension with a beam for two extra geophones is possible. A portable PC or a personal digital assistant with a data collection program installed is required.
  
  

Source: Grontmij A/S
Figure 2. Photo. Grontmij Pavement Consultants FWDs.

Dynatest®

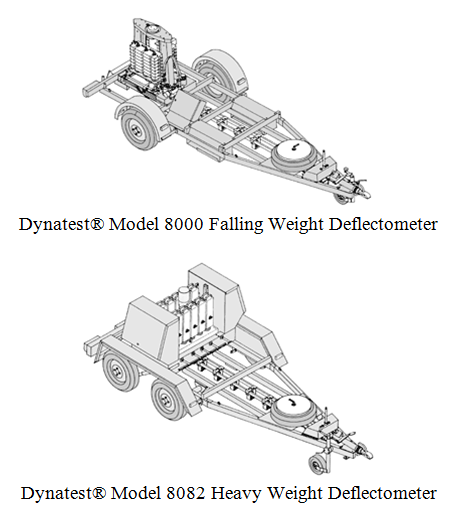
The Dynatest® FWD is a trailer-mounted system with an operations control computer located in the tow vehicle. The computer controls the complete operation of the FWD, including the lowering and raising of the load plate and deflection sensor bar as well as the sequencing of drop heights. Many FWDs are fitted with external cameras to help operators precisely align on selected testing locations.

Figure 3 shows a comparison of the two Dynatest® FWD trailer-mounted models, the 8000 and the 8082. The 8000 model supports drop masses from 110 to 770 lb, resulting in peak impact loads from 1,500 to 27,000 lbf, whereas the 8082supports drop masses from 441 to 1,543 lb, resulting in peak impact loads from 6,500 to 54,000 lbf.⁽⁷⁾ Typical testing production rates range from about 200 to 300 points per day, depending on traffic control requirements and specific testing locations.

Two different plate sizes can be used with the Dynatest® FWD—an 11.8-inch-diameter plate or a 17.7-inch-diameter plate. The smaller plate is typically used for street and highway pavements, whereas the larger plate is commonly used on airfield pavements (and generally on the heavy-weight FWD model 8082).

The Dynatest® FWD is used in FHWA’s LTPP Program, for which pavement deflection measurements have been routinely collected on more than 900 pavement sections since the late 1980s. Dynatest® also performs calibrations at its facilities in Florida and California, and some State transportation departments also have their own calibration facilities.

Source: Dynatest® Consulting, Inc.
Figure 3. Drawings. Comparison of two Dynatest® FWDs.

JILS™ (Foundation Mechanics, Inc.)

JILS™ produces three FWD systems: JILS-20, JILS-20HF, and JILS-20T with ground-penetrating radar (GPR). The JILS-20 is a trailer-mounted FWD. It is mounted on a double axle trailer. Like the Dynatest® FWDs, the system includes a 12-inch loading plate, DMI, temperature measurement hardware, and a video monitoring system. It has a separate gasoline engine with a 12-V alternator. Also, up to 10 sensors could be mounted in the vehicle. The company also provides its FWD data collection software.⁽⁸⁾ The JILS‑20HF is a heavy-load FWD. It is designed for testing pavement such as airfields or thick highway pavements. The specifications are similar to the JILS-20. The JILS-20T is identical to the JILS-20 except that it incorporates a GPR system with the FWD.

Applied Research Associates, Inc. (ARA) conducted an independent study to compare the JILS-20T and Dynatest® model 8002 FWDs.⁽⁹⁾ Table 1 summarizes the key design and performance features of both FWDs. According to study findings, the two FWDs show similar trends. For AC pavement, the JILS™ FWD gave an average deflection 0.38mil greater than the Dynatest® FWD, corresponding to a 2.4-percent average difference. In the case of chip seal, the average difference was 1.47 mil (3.4-percent difference). The study also compared backcalculated subgrade moduli, average deflection basins, and loading time history data. ARA concluded that both FWDs provided satisfactory data.⁽⁹⁾

Table 1. Comparison of JILS™ and Dynatest® FWD features.

KUAB (Engineering and Research International, Inc.)

The KUAB FWD is a trailer-mounted dynamic impulse loading device that can be towed by any suitable towing vehicle.⁽¹⁰⁾ Similar to other FWDs, the KUAB device has a loading system and series of deflection sensors. However, it also has its own defining characteristics, including a metal housing completely enclosing the loading system (see figure 4). A few other features include the following:

• Two-mass configuration: The initial load mass is dropped onto an intermediate buffering system. It transmits the force to another buffering system, which in turn transmits the load to the load plate. The use of the two-mass system creates a smoother load pulse that simulates the actual effects of a moving vehicle.⁽¹¹⁾
  
  
• Segmented load plate: This type of plate ensures a uniform pressure distribution over the full area of the plate.
  
  
• Seismometers: Seven seismometers contact the surface during testing and measure the deflection from the plate. One sensor is placed over the load, while the other six are situated behind and in front of the load to measure displacement at different distances from the plate. Each seismometer includes an LVDT. Compared with other FWD sensors, seismometers may provide more direct deflection readings and have a larger measurement range. The deflection measuring sensors have a range of 0 to 200 mil.
  
  

Four KUAB models are available (KUAB 50, KUAB 120, KUAB 150, and KUAB 240), with the primary difference being the magnitude of the load that can be applied. The KUAB 50 is the lightest, with a standard load range of 3,000 to 14,000 lbf. It could be used on highway, street, and parking lot pavements. The KUAB 120 adds an 18-inch segmented load plate and has a standard load range of 1,500 to 27,000 lbf. The KUAB 150 model offers a standard load range of 3,000 to 33,000 lbf, making it a suitable testing device for airport pavements, as well. The heaviest KUAB 240 device can impart a load of 66,000 lbf, making it suitable for use in airfield applications. All four models support up to seven deflection sensors.

As with the Dynatest® FWD, the KUAB has two loading plates available: 11.8 or 17.7 inches in diameter.

Source: Engineering and Research International, Inc.
Figure 4. Photo. KUAB FWD.⁽¹⁰⁾

Evaluation of Impulse Load Equipment

The FWDs described in the previous section have the ability to closely simulate the loading characteristics of a moving wheel load and to measure the deflection basin.⁽¹⁰⁾ However, these FWDs are relatively complex electromechanical systems, require traffic control when performing testing, and have a high initial cost.⁽¹⁰⁾

One characteristic that should be considered when interpreting FWD deflection data is the loading time. The loading time is important to consider when evaluating differences in backcalculated moduli of viscoelastic materials because shorter loading times generally result in higher backcalculated modulus values for hot mix asphalt (HMA)⁽¹¹⁾. The Dynatest® FWD produces a loading time of about 28 to 35 ms, whereas the JILS™ and KUAB devices produce a loading time of about 24 and 80 ms, respectively.⁽¹¹⁾ The Carlo Bro FWD produces a loading time of about 20 to 30 ms.

REVIEW OF STATUS OF FWD DATA COLLECTION, ANALYSIS, AND INTERPRETATION

Data Collection and Related Issues

Typical testing patterns for FWD testing vary depending on the purpose of the testing. For network-level testing, deflection testing is conducted at 500 to 1,500 ft in a single traffic lane.⁽¹¹⁾ This level of testing is normally sufficient to provide a general indicator of structural adequacy of the pavement network. For project-level testing, the test point spacing should be adequate to capture the variability in structural capacity of the pavement.⁽¹²⁾ Typical project-level testing intervals for HMA pavements are between 100 and 500 ft, with the shorter testing interval warranted for pavements in poorer condition and the larger testing interval appropriate for pavements in better condition. The recommended testing locations are on the outer wheel (OW) path of the outer traffic lane.

The primary issues related to FWD data analysis and interpretation are (1) errors in measurement (relevant to static and dynamic backcalculation) and (2) signal noise and truncation (relevant for dynamic analysis only).

FWD Data Errors

According to Irwin, there are three main sources of errors in FWD data: seating errors, random errors, and systematic errors.⁽¹³⁾ Irwin, Yang, and Stubstad showed that even very small deflection errors (on the order of 2 microns or less) can lead to very large errors in the backcalculated moduli.⁽¹⁴⁾ Irwin found the following:

• Seating errors: Seating errors were caused by the rough texture of pavements and were of more critical importance in the testing of HMA pavements. These errors could typically be eliminated by applying a seating drop at each new test point, allowing the deflection sensors to become seated. Seating drop data should not be used in analyzing the pavement structure.
  
  
• Random errors: Random errors were associated with the analog-to-digital conversion of the deflections. They were on the order of ±2 microns.⁽¹⁴⁾ Random errors could not be completely eliminated; however, they could be reduced by taking multiple readings and averaging the results. This reduction was proportional to the square root of the number of observations used in computing the mean. For example, if four replicate FWD drops (with the same height) at the same point were averaged, the random error would be reduced by half. Care must be taken to ensure that the multiple readings were random, i.e., that they were not consistently increasing or decreasing owing to liquefaction or compaction caused by the additional drops.⁽¹³⁾

Systematic Errors

Systematic errors are associated with the particular FWD equipment and its specific sensors. Systematic errors are on the order of ±2 percent. FWD specifications therefore call for an accuracy of ±2 percent or ±2 microns, whichever is greater. This specification combines the systematic error and the random error. Systematic errors can be reduced to 0.3 percent or less for each individual sensor, including the load cell, through calibration.⁽¹³⁾

Noise and Truncation of FWD Sensor Signals

The sensitivity of dynamic backcalculation solutions to signal noise is high. Basically, noisy data alter the error function surface enough to cause optimization errors. This can cause the search algorithm to diverge or to converge to a different modulus when regularization techniques are used. The remedy to noise is to preprocess the raw data by filtering out the high-frequency content of the signal (anything above 100 Hz) in deflection and load pulse data. Also, if synchronization problems occur, the dynamic backcalculation algorithm may not work as well, although one could shift the signals similar to what was done in the quasi-static viscoelastic solution.

Another issue that is relevant to dynamic analysis is signal truncation. This problem canlead to large errors in the backcalculated layer parameters when using a frequency-based solution.⁽¹⁵⁾ Therefore, signal truncation will affect the frequency-domain backcalculation. On the other hand, the time-domain backcalculation is not affected by FWD signals truncation. In fact, this is one of the major reasons the research team pursued development of a new time-domain dynamic viscoelastic solution.

Integration Drift

The use of numerical integration of acceleration or velocity information from inertial sensors to obtain position information inherently causes errors to grow with time, which is commonly known as “integration drift.” The main problem is that integrating a signal contaminated with noise and drift leads to an output that has a root mean square (RMS) value that increases with integration time even in the absence of any motion of the sensor. For a single integration, the errors are a function of the duration of the signal. For that reason, to correct for the drift, estimation of deflections using inertial sensors is usually performed with the help of externally referenced aided sensors or sensing systems or prior knowledge about the motion. With aided sensors or sensing systems, Kalman filters (KFs) or extended-Kalman filters (EKFs) are commonly used to fuse different sources of information in an attempt to correct for the drift. A more detailed discussion about the drift is presented in chapter 6, in the subsection entitled Effects of Numerical Errors and Drifts.

Analysis and Interpretation of FWD Data

Deflection measurements can be used for backcalculating the elastic moduli of the pavement structural layers and for estimating the load-carrying capacity for HMA pavements. A number of factors affect the magnitude of measured pavement deflections, which makes the interpretation of deflection results difficult. The major factors that affect pavement deflections can be grouped into the following categories: pavement structure (thickness), pavement loading (load magnitude and type of loading), and climate (temperature and seasonal effects).

There are various approaches for FWD data analysis and interpretation. These can be grouped into two categories: (1) methods of analysis for calculating pavement response (forward analysis) and (2) methods for interpretation of pavement response (backcalculation).

Forward Analysis

Methods of calculating pavement response (forward calculation) include the following: (1) closed-form solutions based on Boussinesq’s original half-space solution, (2) layered elastic solutions based on Burmister’s original two- and three-layer solutions, and (3) FEM-based solutions.^(16–18)

The method of equivalent thicknesses is based on Odemark’s assumptions.⁽¹⁹⁾ This closed-form solution is reported to produce results that are as good as or better than those from static layered elastic and FEM solutions.^(19,20) The method can also be adapted to handle nonlinear subgrade materials. However, the method cannot be used to determine the dynamic modulus master curve of an existing HMA layer.

The layered elastic solutions are the most commonly used among all methods. They are generally restricted to linear elastostatic analysis. The basic assumptions of the solution are the following:

• Surface load is uniformly distributed over a circular area.
  
  
• All layers are homogeneous, isotropic, and linearly elastic.
  
  
• Upper layers extend horizontally to infinity.
  
  
• Bottom layer is a semi-infinite half-space.

These solutions have been shown to produce good results if material behavior remains in the linear range; however, they cannot be used to determine the dynamic modulus master curve of an existing HMA layer.

The available computer programs that use the layered elastic solutions include, but are not limited to, CHEVRON, ELSYM5, and BISAR, NELAPAV, PADAL, VESYS, and KENPAVE. (See references 21–27.)

Finite element analysis (FEA) has also been used for pavement analysis, including three-dimensional (3-D) general-purpose programs, such as SAP, ABAQUS, and ANSYS, and pavement-specific programs for two-dimensional (2-D) axisymmetric (e.g., ILLIPAVE and MICHPAVE) and 3-D solutions (CAPA-3D). The main advantage of FEA is the ability to handle material variability and nonlinearity in both vertical and horizontal directions and to include any number of sophisticated constitutive models. Some of these programs do allow dynamic and/or viscoelastic analyses. However, they are either restricted to a particular constitutive model that may not be suitable for the backcalculation of the dynamic modulus master curve, and/or they involve a large number of elements and input parameters and therefore are much more time consuming to set up and to run.

In addition to the methods discussed here, efficient dynamic solutions were also used for pavement analysis. Computer programs for dynamic analysis of pavement systems use either dynamic damped-elastic finite-layer or FEM models for their forward solutions. The finite layer solutions are based on Kausel’s formulation, which subdivides the medium into discrete layers that have a linear displacement function in the vertical direction and satisfy the wave equation in the horizontal direction.⁽²⁸⁾ Examples of programs containing such solutions include UTDYNAF, UTFWD, GREEN, SAPSI, and SCALPOT. (See references 29–33.)

Backcalculation Analysis

The following paragraphs describe static and dynamic backcalculation methods.

Static Backcalculation Methods: Most existing static backcalculation routines can be classified into three major categories, depending on the techniques used to reach the solution. The first category is based on iteration techniques, which repeatedly use a forward analysis method within an iterative process. The layer moduli are repeatedly adjusted until a suitable match between the calculated and measured deflection basins is obtained. The second category is based on searching a database of deflection basins. A forward calculating scheme is used to generate a database, which is then searched to find a best match for the observed deflection basin. The third and final category is based on the use of regression equations fitted to a database of deflection basins generated by a forward calculation scheme. In principle, these three techniques can be applied to any of the forward analysis methods previously discussed. However, the iterative method is arguably the easiest to implement for dynamic backcalculation solutions.

Most iterative solutions use a search algorithm that is achieved by minimizing an objective function of any set of independent variables (i.e., layer moduli, thicknesses, etc.), which is commonly defined as the weighted sum of squares of the differences between calculated and measured surface deflections, which minimizes to the equation shown in figure 5.

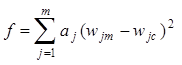
5. Equation. Objective function for the search algorithm.

Where:

m = Number of sensors.
w_jm = Measured deflection at sensor j.
w_jc = Calculated deflection at sensor j.
a_j = Weighing factor for sensor j.

The equation shown in figure 5 can be solved using nonlinear optimization methods, which locate the least value of the objective function. Many minimization techniques are available in the literature, including the following:⁽³⁴⁾

• Factored secant update method.
  
  
• Modified Levenberg-Marquardt (LM) algorithm.
  
  
• Modified Powell hybrid algorithm.

One of the problems of this approach is that the multidimensional surface represented by the objective function may have many local minima. As a result, the program may converge to different solutions if a different set of seed moduli is used. Another problem is that the convergence can be very slow, requiring numerous calls to a forward analysis program.

An example of an iterative program for static backcalculation is EVERCALC, which uses a modified LM algorithm.⁽³⁵⁾ The program seeks to minimize an objective function formed as the sum of squared relative differences between the calculated and measured surface deflections.

The search method can also take the form of solving the linear set of equations, as shown in figure 6.

6. Equation. Search method using set of equations.

Where:

[F]^k = kth iteration of the m by n matrix of partial derivatives ∂f_j /∂E_i, where j = 1 to m and m is the number of deflections measured, and i = 1 to n where n is the number of layers in the pavement with unknown moduli.
{d}^k = kth iteration difference vector, E_i^k+1- E_i^k, between the new and old moduli.
{r}^k = kth iteration residual vector, w_jc - w_jm, between the most recently calculated and the measured surface deflections.

An example of an iterative program using the above search method is MICHBACK, which uses the modified Newton-Raphson (also called secant) method. The method of least-squares is used to solve the over-determined system of equations (m equations in n unknowns, m > n) in figure 6. If desired, weighting factors can be used for each sensor measurement to emphasize some deflection measurements over others.

Dynamic Backcalculation Methods: Most dynamic backcalculation methods use dynamic, damped-elastic finite-layer or FEMs for their forward solutions. Dynamic backcalculation methods are based on either frequency- or time-domain solutions. For the former procedure, the applied load and measured deflection time histories are transformed into the frequency domain by using the fast Fourier transform (FFT). Backcalculation of layer parameters is done by matching the calculated steady-state (complex) deflection basin with the frequency component of the measured sensor deflections at one or more frequencies. In time-domain backcalculation, the measured deflection time histories are directly compared with the predicted results from the forward analysis. One of the advantages of this method is that matching can be achieved for any time interval desired. Uzan compared the two methods and concluded that time-domain backcalculation was preferred over frequency-domain backcalculation.⁽³⁶⁾

BACKCALCULATION COMPUTER PROGRAMS

Static Backcalculation Programs

Numerous computer programs for performing automated backcalculation have been developed. Some of the known static backcalculation computer programs and their characteristics are presented in table 2. Different versions of these programs exist, with improved and/or updated editions being periodically released. Most of the automated backcalculation programs rely on static analysis and a linear elastic layer program. Notable exceptions include ELMOD, which can use either Odemark’s method or the FEM in addition to the layered elastic solution, and MODCOMP and EVERCAL, which can handle nonlinear material properties.

Accuracy and Reliability

Many of the programs developed for production purposes are intended to get to an “accurate” solution reliably. While most static backcalculation programs usually converge to a solution reasonably quickly and reliably, one cannot assert the uniqueness of the set of layer moduli derived from any search method. For this reason, many programs use various controls to guide the iterative search toward an “acceptable” set of layer moduli. These include making some assumptions about the type of pavement system being analyzed (e.g., assuming that layer moduli decrease with depth, that the subgrade modulus is constant with depth, that a rigid layer exists a certain depth, etc.) and limiting the acceptable range of moduli for each individual layer type.

Required Inputs

Required inputs typically include peak sensor deflections and their location, peak load values, the number of layers in the pavement system and their thicknesses, and assumed values for Poisson’s ratios. Most programs also require seed moduli as input, although some have methods that generate these from the measured deflections or from regression equations.

Resulting Outputs

Typical outputs include the measured and calculated deflections, the differences and percent differences, the final set of layer moduli, and the error sums. Most of the existing backcalculation programs allow for 3 to 5 layers; a notable exception is the MODCOMP5 program, which allows up to 15 layers.

Table 2. Commonly available backcalculation computer programs for flexible pavements.

User Friendliness

Because many of the backcalculation programs are developed for production purposes, they are user friendly, require minimum involvement from the user, and provide various features intended to be useful for project-level analysis. Conversely, those programs written for use in research tend to lack the features needed for production. They also usually allow and require significant involvement from the user. These include dynamic backcalculation programs that rely on dynamic analysis to calculate the deflection time histories and those that use available general-use FEM programs.

Advantages and Disadvantages

Attempting to do a one-to-one comparison of different backcalculation programs for the purpose of identifying the best one is a difficult task. Each of these programs has pros and cons, and each may be particularly useful in a specific situation. Before making such comparisons, one should first define the purpose in doing backcalculation and the evaluation criteria that one will use. In general, the advantage of using simpler methods is that they are very fast and easy to use. Their disadvantage is that they are limited in their interpretation of the FWD data. For example, most static backcalculation programs are limited to five layers. This may not be sufficient to characterize realistic pavement profiles that comprise five or more layers, and such programs cannot be used to allow for variation in subgrade modulus with depth, for example. On the other hand, more advanced methods of backcalculation, which theoretically allow backcalculation of a larger number of parameters, are computationally expensive and time consuming. Also, they are not guaranteed to converge when using real field-measured data. For most State transportation departments, the ultimate purpose of backcalculation is to aid in rehabilitation design so that purpose should be a strong criterion for selecting a program.

Dynamic Backcalculation Programs

A number of computer programs have been developed for dynamic backcalculation of flexible pavement layer parameters. Each program employs a particular forward model and a specific backcalculation scheme. All of these programs require the time histories of the load and deflection sensors. Theoretically, because these time histories contain more information than just the peak values of load and deflection, dynamic backcalculation programs can backcalculate a larger number of parameters when using synthetically generated deflection time histories. However, there are serious challenges when using measured field data. For example, the frequency-domain solutions can lead to large errors if the measured FWD records are truncated before the motions fully decay in time.

Time-domain backcalculation solutions present another set of challenges. For example, the time synchronization between the load and sensor records and the digitization of the response can be problematic. Noise in the data and the ill-posed nature of the inversion problem can be amplified when matching traces of time histories, requiring special filtering and regularization techniques that are not easy to implement. In addition, unlike frequency-domain analysis, where the properties are backcalculated at each frequency independently, time-domain backcalculation precludes making a choice on the behavior of material properties with frequency; that is, they either assume a constant HMA modulus (similar to static backcalculation) or a prescribed function of the HMA layer modulus with frequency (e.g., linear relation in the log-log space). While this assumption may be acceptable for unbound materials, it may significantly affect the predicted response of the HMA layer because of its viscoelastic nature. Finally, none of these programs are considered ready for production mode, because they usually require a lot of involvement from the user, are computationally very expensive, and have not been fully evaluated for use with field-measured data. Some of the dynamic backcalculation computer programs and their characteristics are presented in table 3. A brief overview of the programs developed to date is also provided.

Table 3. Dynamic backcalculation programs for flexible pavements.

BKGREEN models the pavement as a layered elastic system in terms of dynamic Green flexibility influence functions using Kausel’s formulation of discrete Green functions for dynamic loads in linear viscoelastic layered media.^(28,31) Backcalculation is done at multiple frequencies, and the set of layer moduli is determined using a nonlinear least squares technique. The solution can cause some computational difficulties at certain frequencies because of the numerical complications associated with implementing infinite integration in computer codes.

Uzan presented two dynamic linear backcalculation procedures—one in the time domain and the other in the frequency domain.⁽³⁷⁾ Both approaches use the program UTFWIBM as the forward model and Newton’s method as the backcalculation solution. UTFWIBM uses the finite layer solutions (based on Kausel’s formulation), which subdivides the medium into discrete layers that have a linear displacement function in the vertical direction and satisfy the wave equation in the horizontal direction.

PAVE-SID is a computer program that uses the SCALPOT program to generate frequency response curves; a system identification technique is applied for matching computed frequency data to extract pavement properties.⁽³³⁾ SCALPOT computes the dynamic response of a horizontally layered viscoelastic half-space to a time-dependent surface pressure distribution.

FEDPAN is a FEM program that can perform both static and dynamic backcalculation for three-layer pavement systems using the CHEVDEF backcalculation algorithm.^(38,45) This program can simulate the effects of pavement inertia and damping in the dynamic analysis and material nonlinearity in the static analysis.

Losa used SAPSI as the forward program and a nonlinear least squares optimization technique (LM method) for multifrequency backcalculation.^(32,39) The HMA and subgrade materials were assumed to be frequency dependent while the base/subbase material was assumed to be frequency independent.

Al-Khoury et al. developed an axisymmetric layered solution as a forward model using the spectral element technique and used the modified LM and Powell hybrid methods for solving the resulting system of nonlinear equations.^(2,40,46)

Meier and Rix developed an artificial neural network (ANN) solution that has been trained to backcalculate pavement layer moduli for three-layer flexible pavement systems using synthetic dynamic deflection basins.^(47,48) The dynamic pavement response was calculated using an elastodynamic Green function solution based on Kausel’s formulation.⁽²⁸⁾

Work by Chatti developed the DYNABACK computer program that allows for different dynamic backcalculation algorithms for both frequency-based and time-based solutions.^(15,42,43) The DYNABACK program uses the SAPSI program as its forward solution and an expanded version of the modified Newton-Raphson algorithm in the MICHBACK program as its backcalculation solution.^(32,49) The solution uses the least squares minimization technique to solve the over-determined set of equations, which are real-valued and correspond to the peak transient deflections and their corresponding time lags relative to the peak load. The DYNABACK program includes two basic solutions with several options for backcalculating different layer parameters: (1) frequency-domain backcalculation at one or multiple frequencies and (2) time-domain backcalculation using peak responses or time history traces. Theoretically, single frequency backcalculation can be used to backcalculate up to 8 parameters while multiple frequency backcalculation can be used to backcalculate up to 15 parameters. The same is true for time-domain backcalculation using peak responses and traces, respectively. However, when using measured deflection time histories, the number of backcalculated parameters must be reduced to fewer than eight.

Finally, Turkiyyah has been developing an improved EVERCALCII program that uses the complete FWD sensor time histories to recover pavement layer moduli distribution and thicknesses using thin computational layers that discretize the profile.⁽⁴⁴⁾ In this solution, physical layer thicknesses can be obtained, after backcalculation of thin computational layer moduli, by grouping thin layers of similar moduli values. Two regularization techniques are employed. One involves the absolute values of the moduli to prevent physically unrealistic solutions with large layer moduli, while the second controls the gradient of the moduli in the vertical direction to prevent convergence to profiles with neighboring layers that alternate between high and low moduli. In addition, a “continuation scheme” is used to control the weights on the regularization terms to overcome the ill-posed nature of the optimization problem. Because this solution relies on backcalculating the moduli of the relatively large number of elements that make up these thin computational layers, the computational effort for solving the inverse problem is very significant. Efforts are underway to speed up the forward (FEM) solution.

MODELING ISSUES

Several specific modeling issues must be considered when selecting backcalculation solutions, as described in the following subsections.

Static Versus Dynamic Response

The FWD test consists of dropping a large weight from a specified height, which creates a 20- to 60-ms impulse load, simulating a moving wheel load. This creates waves in the pavement system and underlying subgrade soil. These elastic waves propagate with distance and are partly reflected at the interface between any given two successive layers, with the remaining wave energy penetrating and propagating to the next layer, and the process is repeated. These waves bounce up and down a few hundred times in a given test. The deflection time histories lag the load pulse, with the time lag increasing as the distance between the load plate and the sensor increases. So, clearly, the FWD test is a dynamic test. Therefore, to maximize the effective interpretation of FWD data, the forward solutions must be able to account for the following physical conditions encountered in FWD testing:

• Transient nature of the FWD load (i.e., time varying pulse load of arbitrary shape).
  
  
• Dynamic effects (i.e., ability to model viscous and inertial internal forces).
  
  
• Wave propagation effects (i.e., the ability to model the propagation of the stress waves imparted by the FWD impulse in time and space).
  
  
• Viscoelastic effects (i.e., the ability to incorporate a frequency-dependent HMA dynamic modulus or its viscoelastic equivalent, the time-dependent relaxation modulus).

The difference between static response and dynamic response can be defined in terms of the internal forces involved. In static analysis, only elastic forces are considered. On the other hand, viscous and inertial forces are considered in addition to the elastic forces in dynamic analysis. The question therefore is whether the effects of viscous and inertial forces are significant enough that one cannot afford to ignore them when characterizing the in situ conditions of a pavement system under an FWD test. Most pavement engineers argue that backcalculation is an exercise that determines pavement parameters—not properties—that are to be used within a given mechanistic framework. Therefore, it is acceptable to use static analysis and to backcalculate parameters that are compatible with the current mechanistic-empirical design framework that is grounded in static and not dynamic analysis. However, advocates for dynamic analysis maintain that such an approach takes advantage of more information provided by the test, which allows for backcalculating more parameters such as layer thicknesses or, perhaps more important, the modulus versus frequency curve of the HMA layer. Also, in certain cases, such in the presence of a stiff layer or water table at shallow depth, the effect of dynamics on pavement response is more important.

Time-Domain Versus Frequency-Domain Backcalculation

Uzan compared the two methods and concluded that time-domain backcalculation is preferred over frequency-domain backcalculation.⁽³⁶⁾ Measured field data from FWD tests using current technology contain several types of measurement and calibration errors, and thus the developed algorithms and computer programs for dynamic backcalculation, must address the following serious challenges:

• Large errors can be introduced when the measured deflection signals are truncated before the motions fully decay in time. Such errors are observed when frequency-domain solutions are used.
  
  
• The periodicity of the signal assumed in the discrete Fourier transform (DFT) algorithm cannot accurately disclose the frequency content of the transient FWD time histories with short duration.
  
  
• The DFT is very sensitive to noise, which is always present in the FWD.^(42,50,51)
  
  
• The DFT is impractical for representing the fundamental properties of a viscoelastic material, such as creep compliance or dynamic modulus, because of a large number of harmonics necessary for modeling them.^(52,53) This is also the reason most of the frequency-domain solutions use the damping ratio concept and hence fail to model and/or backcalculate the fundamental properties of a viscoelastic material.
  
  
• The means of an FWD load pulse and corresponding deflection time histories are all nonzero. Dynamic solutions that have been developed in the context of soil dynamics and earthquake engineering take advantage of the FFT, which reduces computation time by orders of magnitude. However, the FFT algorithm assumes a zero mean function. This assumption is valid for earthquake records but not for an FWD pulse. By default, the output of the FFT algorithm is equal to (A*N)/2, where A is the amplitude of the input series except for the first element of the Fourier series (when the frequency is equal to zero). The standard magnitude of the DFT of the input series is equal to the FFT amplitude normalized such that the sum of the squares of the inputs is equal to the mean of the squares of the outputs (i.e., the total power determined from the time series equals the mean power determined from the spectrum) as shown in the equation in figure 7.

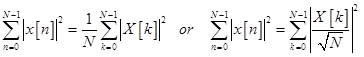
Figure 7. Equation. Parseval theorem.

Where x[n] is the deflection time series; X[k] is the DFT of x[n], both of length N. With the normalization shown in figure 7, the DFT of a nonzero-mean function at zero frequency will be the equation shown in figure 8.

Figure 8. Equation. DFT of a nonzero-mean function at zero frequency.

However, when the input has a nonzero mean, the amplitude of the DFT at zero frequency should be the mean value of the input. Therefore, this normalization is correct only if the input series has a steady state component that is equal to the mean value. However, FWD loading is a transient loading with a mean value but not a steady state component. Also, functions with a nonzero average value may produce a zero frequency component that obscures more interesting components. Adding a large enough quiet zone to a transient nonzero mean signal is a workaround that is often used. However, doing so affects analysis time, and it may not be useful in this case.

All the above issues emphasized the necessity of using a time-domain-based dynamic solution that is also able to model the viscoelastic response of the HMA layer(s).

Linear Versus Nonlinear Material Response

When pavement structures are thin enough or the applied loads and corresponding stresses are high enough, the subgrade material will likely exhibit stress-softening, nonlinear behavior (i.e., its response increases at a higher rate than the load or stress increases). This behavior translates to the subgrade modulus changing with depth and radial distance from the load. If the forward model uses a layered solution that assumes linear material behavior, it can only use one modulus value for an entire layer. Consequently, the backcalculated modulus to match the measured deflections is an averaged value. Typically, the backcalculated subgrade modulus is higher than the value obtained from laboratory measurement by a factor of 2 to 3, although this difference is not entirely caused by nonlinear material response.

On the other hand, granular (cohesionless) materials used in bases and subbases are stress dependent in a different (positive) way (i.e., their modulus increases with increasing confinement). Similar to the subgrade modulus, this leads to a base/subbase modulus that varies with depth and radial distance from the load, and any linear backcalculation exercise can only lead to an averaged modulus value. The combination of these phenomena often leads to a base modulus that is lower than the subgrade modulus despite the base material being superior to the subgrade material. One way of addressing this problem is to introduce an artificial layer. However, a more direct way of addressing the problem is to treat the subgrade as a nonlinear elastic material with a stress-dependent modulus i.e., with a modulus that varies with stress.⁽⁵⁴⁾ Ullidtz argued that the effect of the positive nonlinearity in granular base/subbase layers on backcalculation results was less important.⁽⁵⁴⁾

Ideally, only the FEM can model the variation of moduli with depth and radial distance. However, some models based on layered elastic theory can handle nonlinear behavior approximately (e.g., NELAPAVE and KENPAVE). Ullidtz combined the method of equivalent thickness with a stress-dependent subgrade modulus to handle material nonlinearity and reported that this approach was superior to FEM.⁽⁵⁴⁾

Bedrock or Stiff Layer Effect

A stiff layer condition can exist if there is shallow bedrock, a stiff clay layer, or a groundwater table. The effect of a stiff layer at a shallow depth can be very significant. Assuming the subgrade layer to be a semi-infinite half-space, while in reality the subgrade layer is only a few yards thick, causes the backcalculated moduli for the upper pavement layers to be incorrect. Generally, when the stiff layer is deeper than about 39 ft, its presence has little or no influence on the backcalculated moduli. The depth to the stiff layer can be evaluated by using a relationship between the deflection, δ_Z, and 1/r, where r is the radius at which it occurs (see figure 9). Several regression equations for different HMA layer thicknesses can be used as a function of r_o and deflection basin parameters.⁽⁵⁵⁾

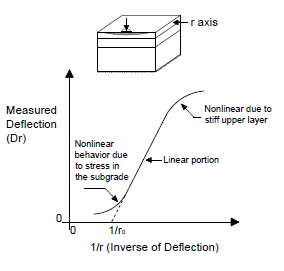
Credit: Washington State Department of Transportation
Figure 9. Drawing and Graph. Plot of inverse of deflection offset versus measured deflection.⁽⁵⁵⁾

An alternative and arguably better way to determine the depth to the stiff layer is to use the free vibration response from FWD deflection sensor measurements and one-dimensional wave propagation theory.⁽⁵⁶⁾ Chatti et al. modified Roesset’s equations to account for different conditions, as shown in the equations in figure 10 (for saturated subgrade with bedrock, use the first equation; for nonsaturated subgrade with bedrock or groundwater table, use the second equation).⁽⁴²⁾

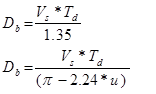
Figure 10. Equation. Calculation of the depth to the stiff layer using the modified Roesset’s equations.

Where:

V_s = S-wave velocity of subgrade material.
T_d = Natural period of free vibration (see figure 11).
u = Poisson’s ratio of subgrade.

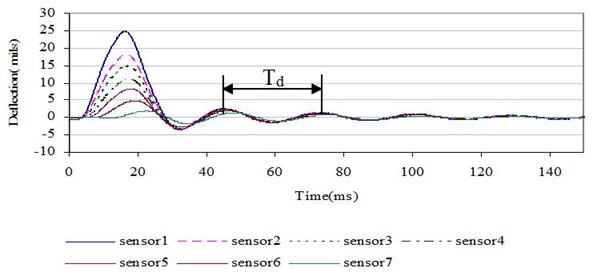
Figure 11. Graph. Natural period T_d from sensor deflection time histories.

The procedure for estimating the depth to the stiff layer described above requires knowledge of the shear wave velocity of the subgrade V_s, which is a function of the modulus value as shown in figure 12.

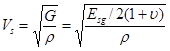
Figure 12. Equation. Shear wave velocity.

However, in the analysis of field data, subgrade properties (shear-wave velocity, unit weight (ρ), and Poisson ratio (u)) are not generally known and therefore need to be either measured or assumed. The method proposed by Lee et al. can be used for estimating the modulus of the subgrade E_sg.⁽³⁵⁾

Temperature and Moisture Effects

Temperature and moisture conditions in the pavement vary over time. This variation occurs daily as well as seasonally. A pavement is strongest during the freezing season (in a freezing climate) because of the frozen state of the underlying materials. On the other hand, even in a freezing environment, the pavement can be at its weakest state during a thaw period, even if it is temporary (e.g., on a sunny day in late winter or during the warmest hours around midday). In areas where there is little or no freezing, seasonal variations can be very important in terms of moisture changes, which affect the modulus of the subgrade and to a lesser extent that of the base layer. For the HMA layers, hourly temperature variations during a given day need to be taken into account because temperature gradients exist in the pavement that can lead to modulus variation with depth. Also, seasonal variations can have a major effect on the modulus of an HMA layer. These effects must be considered when performing backcalculation. It is crucial to test the pavement at different times of the year to gain information about the seasonal variation. Testing should also be conducted at different times during the day to account for daily temperature variations.

Other Effects

Several other issues may need to be addressed in backcalculation analysis, including the following:

• Major cracks in the pavement or testing near a pavement edge or joint can cause the deflection data to depart drastically from the assumed conditions.
  
  
• Layer thicknesses are often not known, and subsurface layers can be overlooked.
  
  
• Layer thicknesses are not uniform, and materials in the layers are not homogeneous.
  
  
• Some pavement layers are too thin to be backcalculated in the pavement model.

RELEVANCE TO MEPDG USE

The required input material properties for HMA pavements in the new MEPDG that are relevant to the use of FWD data and backcalculation results are the following: (1) the time-temperature dependent dynamic modulus, E*, for the HMA layer(s), (2) the resilient moduli for the unbound base/subbase and subgrade materials, and (3) the elastic modulus of the bedrock, if present.⁽¹⁾ The MEPDG also provides an option for considering nonlinear material parameters for the unbound layers for level 1 analysis.⁽¹⁾ However, the performance models used in the software have not been calibrated for nonlinear conditions.

HMA Materials

For HMA materials, level 1 analysis requires conducting E* (complex modulus) laboratory testing at loading frequencies and temperatures of interest for the given mixture. Level 2 analysis does not require E* laboratory testing; instead, the user can enter asphalt mix properties (gradation parameters) and laboratory binder test data (from G* testing or other conventional binder tests).⁽⁴⁾ The MEPDG software calculates the corresponding asphalt viscosity values; it then uses the modified Witczak equation to predict E* and develops the master curve for the HMA mixture.⁽¹⁾ The same procedure is used for level 3 analysis to estimate the HMA dynamic modulus, except no laboratory test data are required for the binder.

For rehabilitation design, the determination of the HMA layer dynamic modulus follows the same general concepts described above, except that the software allows for a modified procedure to account for damage incurred in the HMA layer during the life of the existing pavement. The procedure therefore determines a “field damaged” dynamic modulus master curve as follows:

• For level 1 analysis, the MEPDG calls for the following procedure:⁽¹⁾

1. Conduct FWD tests in the OW path over the project to be rehabilitated; calculate the mean backcalculated HMA modulus, E_i, for the project, including cracked as well as uncracked areas; record the HMA layer temperature at the time of testing; and determine the layer thickness along the project from coring or GPR testing.
   
   
2. Determine mix volumetric parameters and asphalt viscosity parameters from cores and follow the same procedure for determining binder viscosity-temperature properties as for new or reconstruction design.
   
   
3. Develop an undamaged dynamic modulus master curve using the data from step 2 and the modified Witczak equation.
   
   
4. Estimate damage, d_j = E_i/E*, with E_i obtained from step 1 and E* obtained from step3 at the same temperature recorded in the field.
   
   
5. Calculate α'= (1 – d_i) α, where α is a function of mix gradation parameters.
   
   
6. Determine the field damaged dynamic modulus master curve using α' instead of α in the modified Witczak equation.
   
   

• For level 2 and level 3 analyses, no FWD testing is required. The level 2 procedure is similar to the level 1 procedure, except that the indirect resilient modulus, M_ri, obtained from laboratory tests (NCHRP 1-28A) on specimens from field cores, is used in the damage parameter calculation, dj = M_ri/E*.⁽⁵⁷⁾ For the level 3 procedure, no coring or testing is required; instead, typical estimates of HMA mix parameters are entered, and the software calculates the undamaged master curve. The damage factor d_j is estimated from visual condition data.

Unbound Materials

For unbound materials (and bedrock), only level 1 analysis calls for FWD testing in rehabilitation and reconstruction designs. The resilient modulus, M_r, for each unbound layer (including the subgrade) can be either determined in the laboratory using cyclic triaxial tests or backcalculated using standard backcalculation procedures. As discussed in the previous section, while the MEPDG does allow for the generalized nonlinear, stress-dependent model in the design procedure, this approach is not recommended at this time because the performance models in the software have not been calibrated for nonlinear conditions; therefore, the MEPDG does not discuss the option of backcalculating the k₁, k₂, and k₃ parameters in the nonlinear model. The discussion in MEPDG only includes the backcalculation and use of “effective” moduli that would account for any stress sensitivity, cracks, or any other anomalies in any layer within the existing pavement. For level 2 analysis, correlations with strength test data are used. For level3, the guide lists typical modulus values based on soil classification but warns that they are very approximate and strongly recommends some form of testing, especially using FWD testing and backcalculation (level 1).⁽¹⁾

The MEPDG notes that the reason for caution is related to using the wrong assumptions. Either a fairly strong subgrade material may be erroneously assumed to be semi-infinite while it may actually be less than 3 ft thick (e.g., as part of an embankment) or, conversely, a weak subgrade soil may be assumed to be semi-infinite while it may, in reality, be overlying a stronger soil or bedrock. The MEPDG also notes that for granular materials, moduli values that matched FWD backcalculated results were 50- to 70-percent higher than the typical laboratory-tested values, while for subgrade soils, they were two to three times the typical laboratory-determined values.⁽¹⁾

Cementitiously Stabilized Materials

Similar to unbound materials, only level 1 analysis calls for FWD testing in rehabilitation and reconstruction designs. The modulus, E or M_r, for any cementitiously stabilized layer (including lean concrete and cement stabilized base, as well as lime/cement/flyash stabilized soils) can be either determined in the laboratory or backcalculated using standard backcalculation procedures. Layer thicknesses can be obtained by coring or using NDT techniques such as the GPR. The MEPDG recommends performing limited testing on cored lime-stabilized soil specimens to verify/confirm the backcalculated values and notes that backcalculation of modulus values for layers less than 6 inches thick located below other paving layers may be problematic, thus requiring laboratory testing. For level 2 analysis, correlations with strength test data are used. For level 3, the MEPDG calls for estimating the moduli based on experience or historical records and lists typical modulus values. The MEPDG also notes that semi-rigid cementitiously stabilized materials are more prone to deterioration due to repeated traffic loads when used in HMA pavements and suggests some typical (minimum) values for such deteriorated materials.⁽¹⁾

Using Static Backcalculation in the Current MEPDG Procedure

It should be clear from the previous discussion that the analysis in the MEPDG software always uses an E* master curve and therefore does not accept a constant modulus value for the HMA layer(s). This is necessary because the analysis calculates different HMA moduli for the different sublayers comprising the HMA layer(s) as a function of depth, speed, and temperature, as explained in appendix CC of the MEPDG.⁽¹⁾ For rehabilitation of existing pavements, the current MEPDG procedure (level 1) calls for (static) backcalculation of layer moduli, which leads to constant backcalculated moduli for all layers, including the HMA layer. To maintain compatibility of backcalculated layer moduli with the forward analysis in the software, the MEPDG procedure calls for adjusting the HMA dynamic modulus using the damage factor d_j (ratio of backcalculated HMA modulus to predicted E* value using Witczak equation). This effectively shifts the undamaged master curve down while essentially maintaining the variation with frequency as predicted by the Witczak equation. The procedure also calls for adjusting the master curve using the aged viscosity value in the predictive E* equation, which would shift the master curve upward; however, this upward shift would be negligible compared with the downward shift using the backcalculated modulus for the damaged HMA layer, E_i (as explained previously in the HMA Materials section).

Feasibility of Using Dynamic Backcalculation for Future Versions of MEPDG

Ideally, one should be able to determine a curve of HMA layer moduli as a function of frequency using a (dynamic) frequency-based backcalculation algorithm. This would give a more direct estimation of the HMA layer modulus with frequency from actual field conditions as opposed to relying on a laboratory-derived curve such as the Witczak equation. However, care should be taken in interpreting and using such data with the existing MEPDG performance predictions because they have been calibrated using laboratory-derived moduli. Also, recent analyses showed that while dynamic backcalculation methods can backcalculate layer moduli and thicknesses accurately from synthetically generated FWD data for pavement systems with three or more layers, they must address some serious challenges when using field data.⁽⁴³⁾ The frequency-domain method can lead to large errors if the measured FWD records are truncated before the motions fully decay in time. Dynamic, time-domain backcalculation algorithms present another challenge in that they cannot directly determine the HMA modulus as a function of frequency. They either assume a constant HMA modulus (similar to static backcalculation) or a prescribed function of the HMA layer modulus with frequency.